In an equilateral triangle, three identical coins of radii 1 units each, are kept so that they touch each other and also the sides of a triangle. Find the area of the triangle.
The correct Answer is:(4√3+6) sq. unit
The given arrangement of coins is as shown in the given figure.
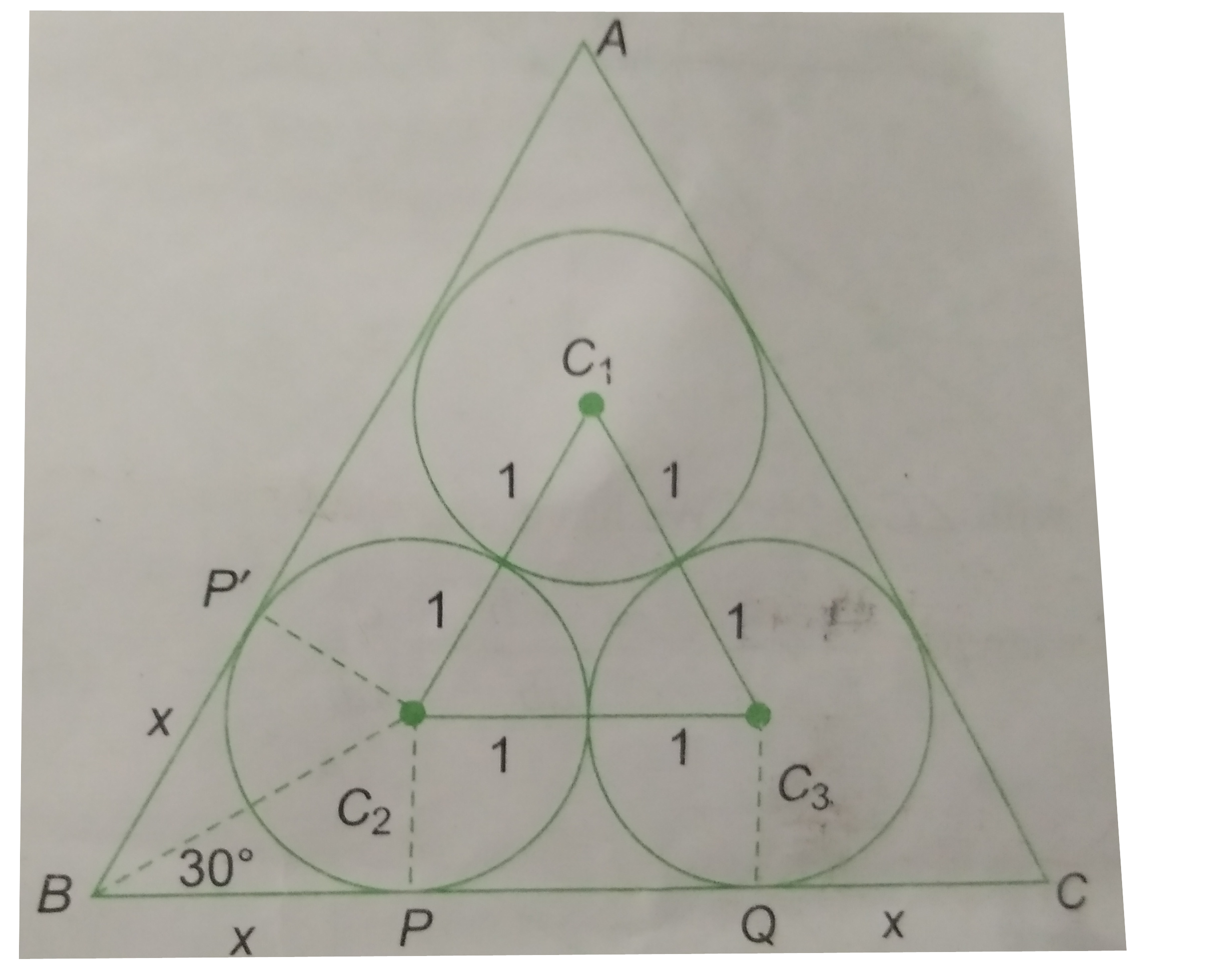
To find the area of the triangle, we need to find its side.
For circle with centre C2, BP and BP' are two tangents from B to circle,
Therefore, BC2 must be angle bisector of ∠B.
∠B=60∘ (∴ΔABC is equilateral)
∴∠C2BP=30∘
∴tan30∘=1x
⇒x=√3
∴BC=BP+PQ+QC
=x+2+x=2+2√3
Area of an equilateral triangle is √34a2, where a is side length.
∴Area of ΔABC=√34(2+2√3)2
=√3(1+3+2√3)
=(4√3+6) sq. unit
Topper's Solved these Questions
- TRIGONOMETRIC FUNCTIONS BOOK - CENGAGECHAPTER - TRIGONOMETRIC FUNCTIONS EXERCISE - Exercise 2.2
8 Videos
- TRIGONOMETRIC FUNCTIONS BOOK - CENGAGECHAPTER - TRIGONOMETRIC FUNCTIONS EXERCISE - Exercise 2.3
4 Videos
- TRIGONOMETRIC FUNCTIONS BOOK - CENGAGECHAPTER - TRIGONOMETRIC FUNCTIONS EXERCISE - SINGLE CORRECT ANSWER TYPE
38 Videos
- TRIGONOMETRIC EQUATIONSBOOK - CENGAGECHAPTER - TRIGONOMETRIC EQUATIONSEXERCISE - Archives (Matrix Match Type)
1 Video
- TRIGONOMETRIC RATIOS AND TRANSFORMATION FORMULASBOOK - CENGAGECHAPTER - TRIGONOMETRIC RATIOS AND TRANSFORMATION FORMULASEXERCISE - Matrix Match Type
1 Video
Similar Questions
In an equilateral triangle, three coins of radii 1 unit each are kept so that they touch each other and also the sides of the triangle. The area of the triangle is (fig) 4:2√3 (b) 6+4√3 12+7√34 (d) 3+7√34
View SolutionIn an equilateral triangle, three coins of radii 1 unit each are kept so that they touch each other and also the sides of the triangle. The area of the triangle ABC is
View Solution
Knowledge Check
Area of equilateral triangle of side a unit is
A√32a2B√34a2C√32aD√34aThe side of an equilateral triangle is 10 cm. Find its area (in cm2).
A10√3cm2B25√3cm2C50√3cm2D75√3cm2The perimeter of a rectangle and an equilateral triangle are same. Also, one of the sides of the rectangle is equal to the side of the triangle. The ratio of the areas of the rectangle and the triangle is
A√3:1B1:√3C2:√3D4:√3
In an equilateral triangle 3 coins of radii 1 unit each are kept so that they touch each other and also the sides of the triangle. Are of the triangle is- 4+2√3 b. 6+4√3 c. 12+7√34 d. 3+7√34
View SolutionIn an equilateral triangle , 3 coins of radii 1 unit each are kept so each other and also the side of the triangle .the area of the triangle is:
View SolutionEach side of an equilateral triangle measure 10 cm. Find the area of the triangle .
View SolutionIf the side of an equilateral triangle is 16 cm, then the area of the triangle is
View SolutionThe perimeter of a rectangle and an equilateral triangle are same. Also, one of the sides of the rectangle is equal to the side of the triangle. The ratio of the areas of the rectangle and the triangle is
View Solution
