In ΔABC if AB=x,BC=x+1,∠C=π3, then the less integer value of x is
A
6
B
7
C
8
D
none of these
Video Solution
Text Solution
The correct Answer is:B
Using cosine rule, we get
x2=(x+1)2+b2−2(x+1)bcos.π3
⇒0=2x+1+b2−(x+1)b
⇒b2−(x+1)b+2x+1=0
Since b is real, we have
⇒(x+1)2−4(2x+1)≥0
→x2−6x−3≥0
⇒x≥3+√12
The least integral value of x is 7
|
Topper's Solved these Questions
- PROPERTIES AND SOLUTIONS OF TRIANGLEBOOK - CENGAGECHAPTER - PROPERTIES AND SOLUTIONS OF TRIANGLEEXERCISE - Exercise (Multiple)
24 Videos
- PROPERTIES AND SOLUTIONS OF TRIANGLEBOOK - CENGAGECHAPTER - PROPERTIES AND SOLUTIONS OF TRIANGLEEXERCISE - Exercise (Comprehension)
34 Videos
- PROPERTIES AND SOLUTIONS OF TRIANGLEBOOK - CENGAGECHAPTER - PROPERTIES AND SOLUTIONS OF TRIANGLEEXERCISE - Exercise 5.11
4 Videos
- PROGRESSION AND SERIESBOOK - CENGAGECHAPTER - PROGRESSION AND SERIESEXERCISE - ARCHIVES (MATRIX MATCH TYPE )
1 Videos
- PROPERTIES OF TRIANGLE, HEIGHT AND DISTANCEBOOK - CENGAGECHAPTER - PROPERTIES OF TRIANGLE, HEIGHT AND DISTANCEEXERCISE - Question Bank
32 Videos
Similar Questions
Knowledge Check
In ΔABC, if AB=AC and ∠B=50∘, then ∠C is equal to
A40∘B50∘C80∘D130∘In ΔABC, DE || BC. If AD = 2x - 1, AE = 2x + 5, BD = x - 3 and CE = x - 1, then the value of x is:
A8B9C10D11In a right-angled triangle ABC, right angled at B, AB=x2, BC = x + 2 and AC = x + 3. The value of x is :
A5B10C12D14
In ΔABC,AD=BC. Find x.
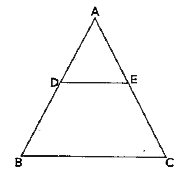
View SolutionIn the figure ΔABC,DE∣∣AB. If AD=2x,DC=x+3,BE=2x−1CE=x then find the value of x.
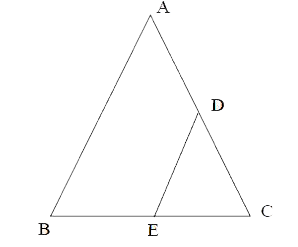
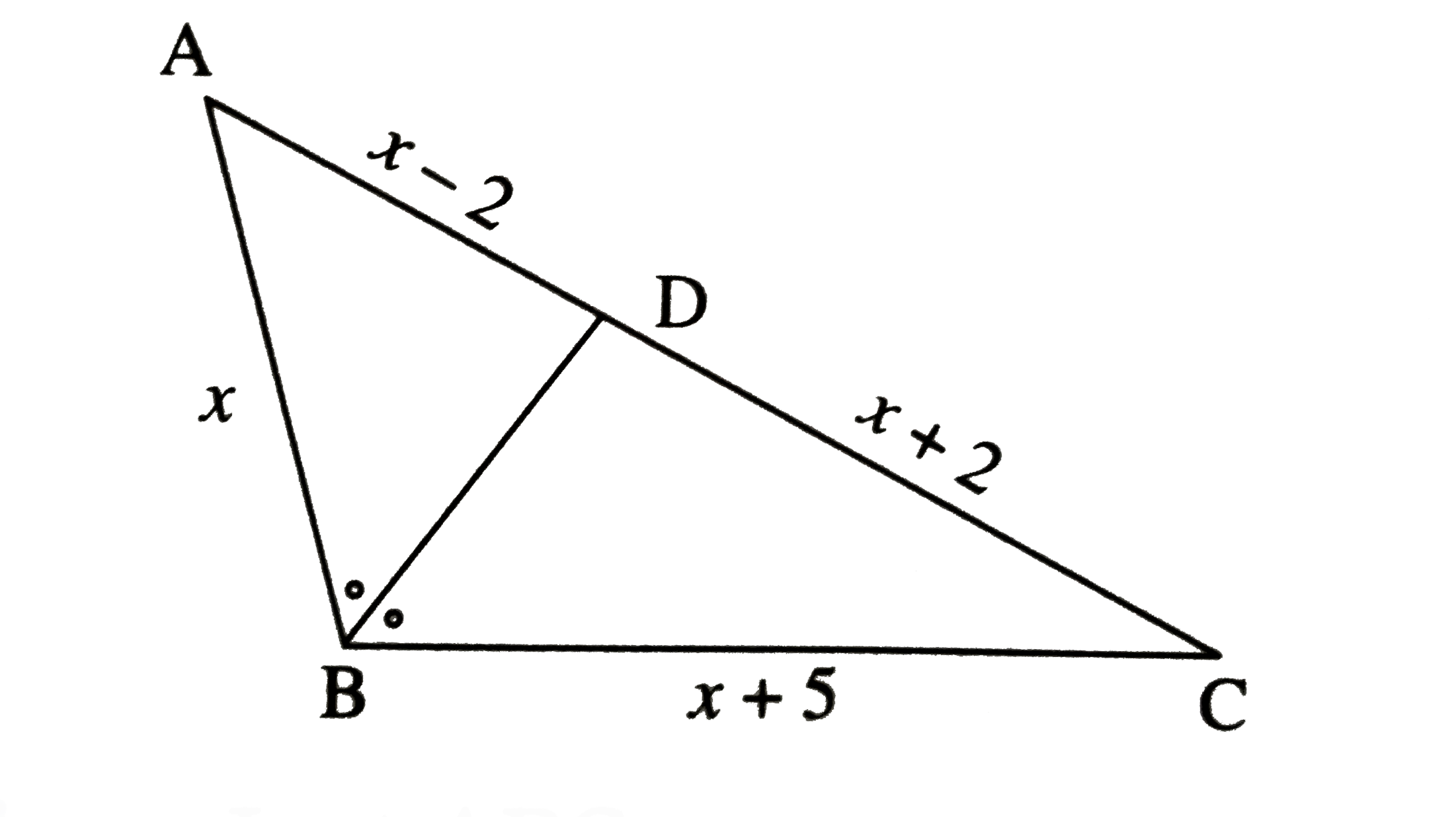
View SolutionIn ΔABC set BD bisects ∠ABC. If AB−x,BC=x+5,AD=x−2,DC=x+2, then find the value of x.
View SolutionIn ΔABC, when DE || BC and AD = x, DB = 3x + 4, AE = x + 3 and EC = 3x + 19 then value of x is:
View SolutionIn ΔABCBC⊥AC at D.E is a point on BC such that ∠BEA=x∘ If ∠EAC=36∘and∠EBD=40∘, then the value of x is:
View Solution
