One end of a spring of negligible unstretched length and spring constant k is fixed at the origin (0,0) . A point particle of mass m carrying a positive charge q attached at its other end . The entire system is kept on a smooth horizontal surface . When a point dipole p pointing towards the charge q is fixed at the origin , the spring gets stretched to a length l and attains a new equilibrium positition (see figure below) . if the point mass is now displaced slightly by Δl<<l from its equilibrium position and released it is found to oscillate at frequency 1δ√km. The value of δ is ________.
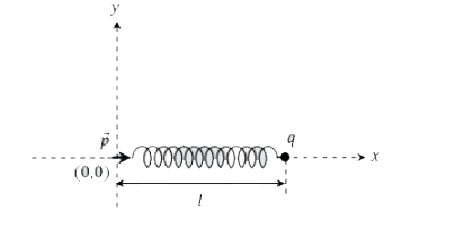
The correct Answer is:03.14
Original frequency f=2λ√km
if dipole appears
At equilibrium
2kp(l+x0)3.qx0 . . . . .(i)

Now disturbed
→x2kp(l+x0+x)3.q−k(x0+x)=m
ma=2kpq(l+x0)3[1+xl+x0]−3−k(x0+x)
=2kpq(l+x0)3[1−3xl+x0kx0−kx=−kx[3x0l+x0+1]
As l is negative
ma=−kx.4, a=4km
New frequency :
f.=12π√4km=2f =1π√km
λ=π=3.14.
Topper's Solved these Questions
- JEE ADVANCED 2020BOOK - JEE ADVANCED PREVIOUS YEARCHAPTER - JEE ADVANCED 2020EXERCISE - SECTION-2
6 Videos
- JEE ADVANCEDBOOK - JEE ADVANCED PREVIOUS YEARCHAPTER - JEE ADVANCEDEXERCISE - PHYSICS SECTION - IV Matrix Match Type
2 Videos
- JEE ADVANCED 2021BOOK - JEE ADVANCED PREVIOUS YEARCHAPTER - JEE ADVANCED 2021EXERCISE - QUESTION
38 Videos
Similar Questions
A particle of mass m moves in circular orbits with potential energy N(r)=Fr, wjere F is a positive constant and r its distance from the origin. Its energies are calculated using the Bohr model. If the radius of the the nth orbit (here h is the Planck's constant)
View SolutionOne mole of a monatomic ideal gas is taken through a cycle ABCDA as shown in the P-V diagram. Column II gives the characteristics involved in the cycle. Match them with each of the processes given in Column I.

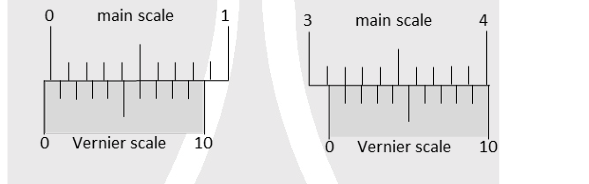
View SolutionThe smallest division on the main scale of a vernier calipers is 0.1cm. Ten divisions of the vernier scale correspond to nine divisions of the main scale. The figure below on the left shows the reading of this calipers with no gap between in two jaws. The figure on the right shows the reading with a solid sphere held between the jaws. The correct diameter of the sphere is.
View SolutionA block of mass 2.0 kg is moving on a frictionless horizontal surface with a velocity of 1.0 m/s figure towards abnother block of equal mass kept at rest. The spring constant of the spring fixed at one end is 100 The spring constant of the spring fixed at one end is 10 N/m. Find the maximum compression of the spring.
View SolutionA block of mass m is attached to one end of a mass less spring of spring constant k. the other end of spring is fixed to a wall the block can move on a horizontal rough surface. The coefficient of friction between the block and the surface is mu then the compession of the spring for which maximum extension of the spring becomes half of maximum compression is .
View SolutionA rod of length l and mass m, pivoted at one end, is held by a spring at its mid - point and a spring at far end. The spring have spring constant k. Find the frequency of small oscillations about the equilibrium position.

View SolutionA spring of spring constant k stores 5 J of energy when stretched by 25 cm. It is kept vertical with one end fixed. A mass m is attached to the other end. It maskes 5 Oscillation per second. Then
View SolutionA Bead of mass m is attached to one end of a spring of natural length 'R' and spring cosntant 'k=((sqrt3+1)mg)/R' . The other end of the spring is fixed at point 'A' on a smooth vertical ring of radius 'R' as shown
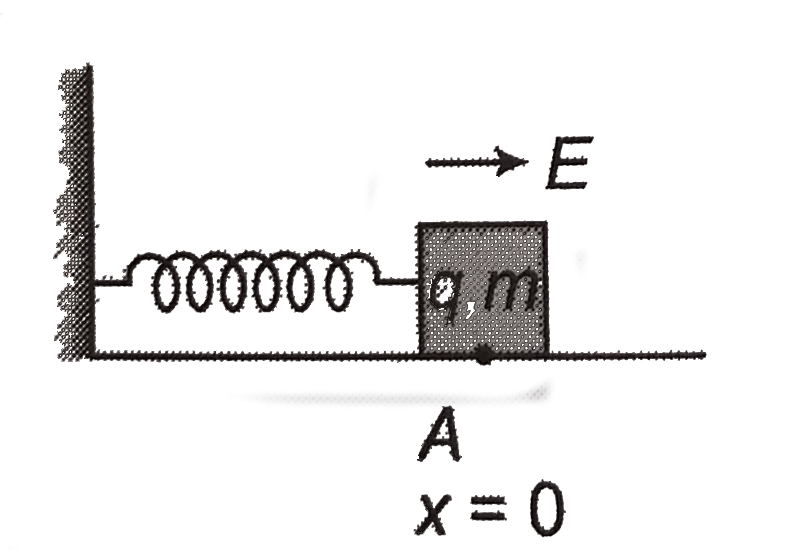
View SolutionA block of mass m having charge q is attached to a spring of spring constant k. This arrangement is placed in uniform electric field E on smooth horizontal surface as shown in the figure. Initially spring in unstretched. Find the extension of spring in equilibrium position and maximum extension of spring.
View SolutionA block of mass M is tied to a spring of force constant k and placed on a smooth horizontal surface. The natural length of the spring is L. P is a point on the spring at a distance (L)/(4) from its fixed end. The block is set in oscillations with amplitude A. Find the maximum speed of point P on the spring.
View SolutionA block of mass m, lying on a smooth horizontal surface, is attached to a spring (of negligible mass) of spring constant k. The other end of the spring is fixed, as shown in the figure. The block is initally at rest in its equilibrium position. If now the block is pulled withe a constant force F, the maximum speed of the block is :

View SolutionA block of mass m, lying on a smooth horizontal surface, is attached to a spring (of negligible mass) of spring constant k. The other end of the spring is fixed, as shown in the figure. The block is initally at rest in its equilibrium position. If now the block is pulled withe a constant force F, the maximum speed of the block is :
View SolutionA bead of mass m can slide without friction on a fixed circular horizontal ring of radius 3R having a centre at the point C. The bead is attached to one of the ends of spring of spring constant k. Natural length of spring is R and the other end of the spring is R and the other end of the spring is fixed at point O as shown in the figure. If the bead is released from position A, then the kinetic energy of the bead when it reaches point B is
View SolutionA block of mass m, lying on a smooth horizontal surface, is attached to a spring (of negligible mass) of spring constant k. The other end of the spring is fixed, as shown in the figure. The block is initally at rest in its equilibrium position. If now the block is pulled withe a constant force F, the maximum speed of the block is :

View SolutionA block of mass m sliding on a smooth horizontal surface with a velocity →V meets a long horizontal spring fixed at one end and having spring constant k as shown in figure. Find the maximum compression of the spring?

View Solution
