Let ABCD be a tetrahedron such that the edges AB,ACandAD are mutually perpendicular. Let the area of triangles ABC,ACDandADB be 3, 4 and 5sq. units, respectively. Then the area of triangle BCD is 5√2 b. 5 c. √52 d. 52
5√2
5
√52
52
The correct Answer is:a
Area of △BCD=12∣∣∣−−→BC×−−→BD∣∣∣
12(bˆi−cˆj)×(bˆi−dˆk)
=12∣∣bdˆj+bcˆk+dcˆi∣∣
12√b2c2+c2d2+d2b2
Now 6= bc, 8 =cd, 10= bd
b2c2+c2d2+d2b2=200
substituting the value in (i) , we get
A=12√200=5√2

Topper's Solved these Questions
- DIFFERENT PRODUCTS OF VECTORS AND THEIR GEOMETRICAL APPLICATIONS BOOK - CENGAGECHAPTER - DIFFERENT PRODUCTS OF VECTORS AND THEIR GEOMETRICAL APPLICATIONS EXERCISE - Exercise
337 Videos
- DETERMINANTSBOOK - CENGAGECHAPTER - DETERMINANTSEXERCISE - Question Bank
23 Videos
- DIFFERENTIAL EQUATIONSBOOK - CENGAGECHAPTER - DIFFERENTIAL EQUATIONSEXERCISE - Question Bank
25 Videos
Similar Questions
Knowledge Check
A and B are isotopes. B and C are isobars. If dA,dB and dC be the densities of nuclei A, B and C respectively then
AdA>dB>dCBdA<dB<dCCdA=dB=dCDdA=dB<dC
If D,E,F are the mid-points of the sides BC,CAandAB respectively of a triangle ABC, prove by vector method that AreaofDEF=14(areaofABC).
View SolutionIn the given figure, ABCD is a rectangle with AD=4 units and AE=EB.EF is perpendicular to DB and is half of DF . If the area of the triangle DEF is 5 sq. units, then what is the area of ABCD ? 18√3 sq. units (b) 20 sq. units (c) 24 sq. units (d) 28 sq. units
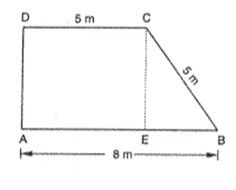
View SolutionIn quadrilateral ABCD show in Figure. ABDC and AD⊥AB. Also, AB=8m, DC=BC=5m. Find the area of the quadrilateral.
View SolutionABC is a triangle in which D is the mid-point of BC ,E andF are mid-points of DC and AE respectively. If area of ABC is 16cm2, find the area of DEF
View SolutionIn ABC,∠A=900andAD is an altitude. Complete the relation BDDA=AB() .
View SolutionIf A(1,-1,2),B(2,1,-1)C(3,-1,2) are the vertices of a triangle then the area of triangle ABC is
(A)√12 (B) √3(C) √5 (D) √13
View SolutionABCD is a cyclic quadrilateral whose diagonals ACandBD intersect at P . If AB=DC, Prove that : PAB≅PDC PA=PDandPC=PB ADBC
View Solution
