Find the equation of parabola
(i) having focus at (0,-3) its directrix is y = 3.
(ii) having end points of latus rectum (5,10) and (5,10) and which opens towards right.
(iii) having vertex at origin and focus at (0,2)

Video Solution

Text Solution

Verified by Experts

(i) Here, focus S(0,-3) and directrix y=3 are at same distance from origin is vertex of the parabola and parabola opens downwards.
So, we consider equation $x^{2} = - 4 a y$ .
Here focus is $(0, - 3) \equiv (0, - a)$
$∴ a = 3$
So, equation of parabola is $y^{2} = - 12 x$ .
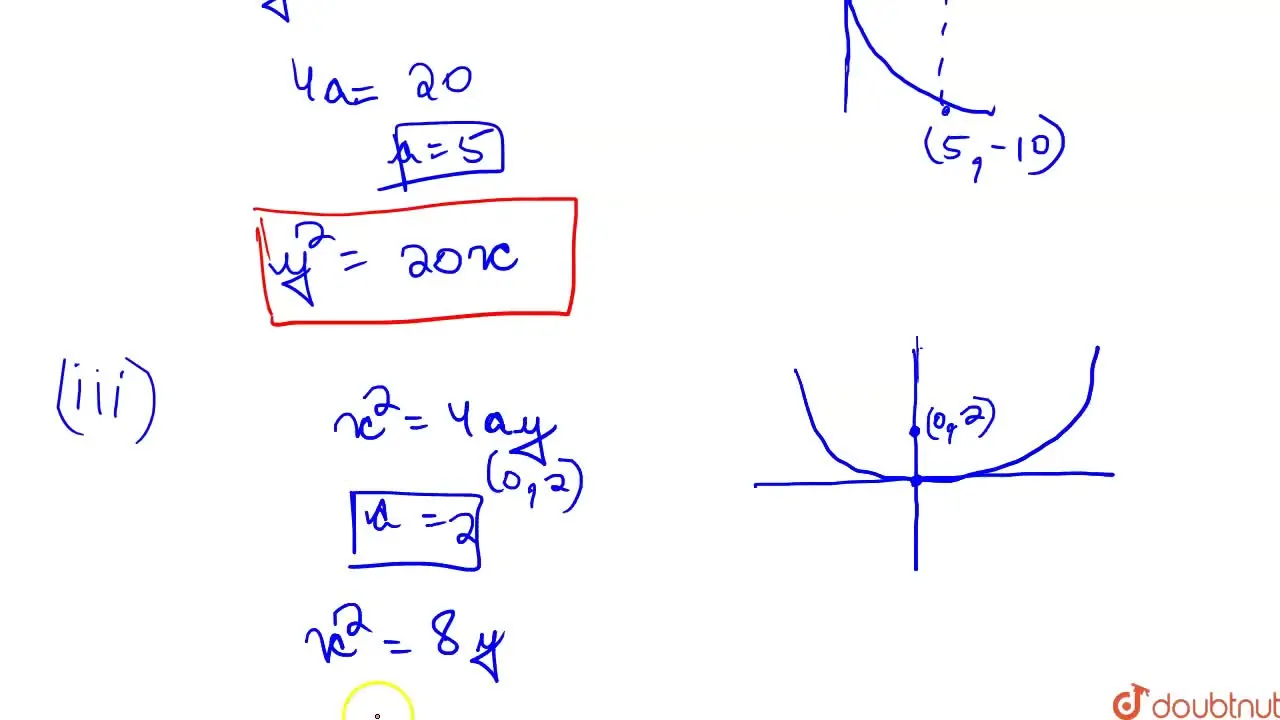
(ii) End points of latus are A (5,10) and B (5,-10).
$∴ S \equiv (5, 0)$
Also, parabola opens towards right.
So, we consider equation $y^{2} = 4 a x$ .
$∴$ Vertex is (0,0) and a=5.
So, equation of parabola is $y^{2} = 20 x$ .
(iii) Vertex is (0,0) focus S(0,2).
So, directrix is y=-2.
So, we consider equation $x^{2} = 4 a y$ , where a=2.
$∴$ Equation of parabola is $x^{2} = 8 y$ .

Updated on:21/07/2023

Find the equation of parabola having focus at (-1, -2) and directrix x-2y+3=0.
A $4 x^{2} + y^{2} + 4 x y + 2 x + 32 y - 16 = 0$
B $4 x^{2} + y^{2} + 4 x y + 4 x + 32 y + 16 = 0$
C $x^{2} + 4 y^{2} + 4 x y + 4 x + 32 y + 16 = 0$
D $4 x^{2} + y^{2} + 4 x y - 2 x + 32 y + 16 = 0$
The equation of a parabola having focus (3, 0) and directrix x + 3 = 0, is
A $y^{2} = 12 x$
B $y^{2} = - 12 x$
C $x^{2} = 12 y$
D $x^{2} = - 12 y$
If the focus of a parabola is at (0,-3) and its directrix is y = 3, then its equation is
A $x^{2} = - 12 y$
B $x^{2} = 12 y$
C $y^{2} = - 12 x$
D $y^{2} = 112 x$