In the adjoining figure , and AB = 4cm find the value of CD.
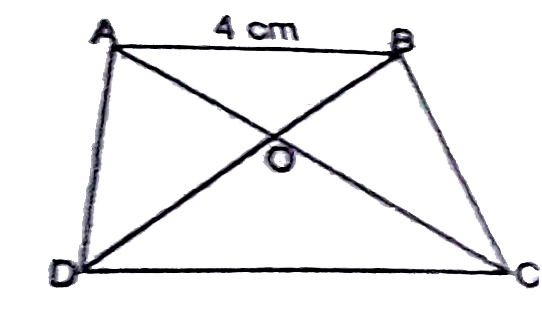
The correct Answer is:CD= 8 cm
Step by step video, text & image solution for In the adjoining figure , (AO)/(OC)= (BO)/(OD) = 1/2 and AB = 4cm find the value of CD. by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Topper's Solved these Questions
- TRIANGLES BOOK - NAGEEN PRAKASHANCHAPTER - TRIANGLES EXERCISE - Exercise 6c
11 Videos
- TRIANGLES BOOK - NAGEEN PRAKASHANCHAPTER - TRIANGLES EXERCISE - Exercise 6d
24 Videos
- TRIANGLES BOOK - NAGEEN PRAKASHANCHAPTER - TRIANGLES EXERCISE - Eercise 6b
1 Videos
- STATISTICS BOOK - NAGEEN PRAKASHANCHAPTER - STATISTICS EXERCISE - Revision Exercise Long Answer Questions
5 Videos
- VOLUME AND SURFACE AREA OF SOLIDSBOOK - NAGEEN PRAKASHANCHAPTER - VOLUME AND SURFACE AREA OF SOLIDSEXERCISE - Revisions Exercise Long Answer Questions
5 Videos
Similar Questions
In figure OAOC=OBOD=12and AB=5cm. Find the value of DC
View SolutionIn the given figure if AOOC=BOOD=12and AB=5cm then find CD
View SolutionIn Fig. 4.96, AOOC=BOOD=12 and AB=5cm . Find the value of DC . (FIGURE)
View SolutionIn the adjoining figure, AB=8cm,DM=6cmandBC=6cm. Find the length of DN
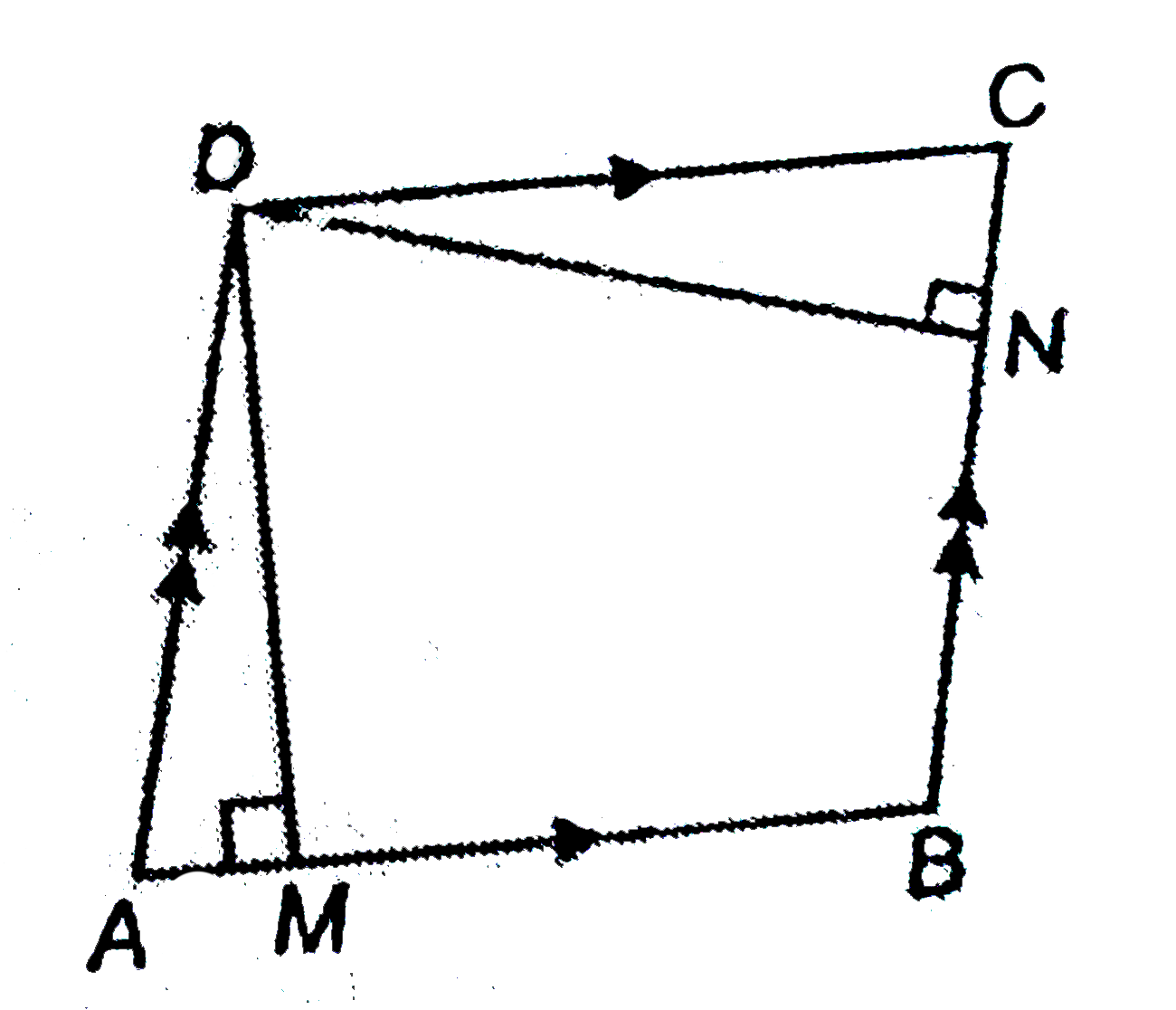
View SolutionIn the adjoining figure, AP=8cm, BP=2cm and ∠CPA=90∘. Find the length of chord CD.
View SolutionIn the adjoining figure AB||CD, find x and y

View SolutionIn the adjoining figure AB||CD .Find the value of x
View SolutionIn the adjoining figure,AB=AC.Find the value of x
View SolutionThe radius of a circle is 10 cm and the perpendicular form the centre to a chord is 8cm. Find the length of the chord.
(ii) The radius of a circle is 10 cm. its one chord is 16 cm long. Find the perpendicular distance of this chord form the centre.
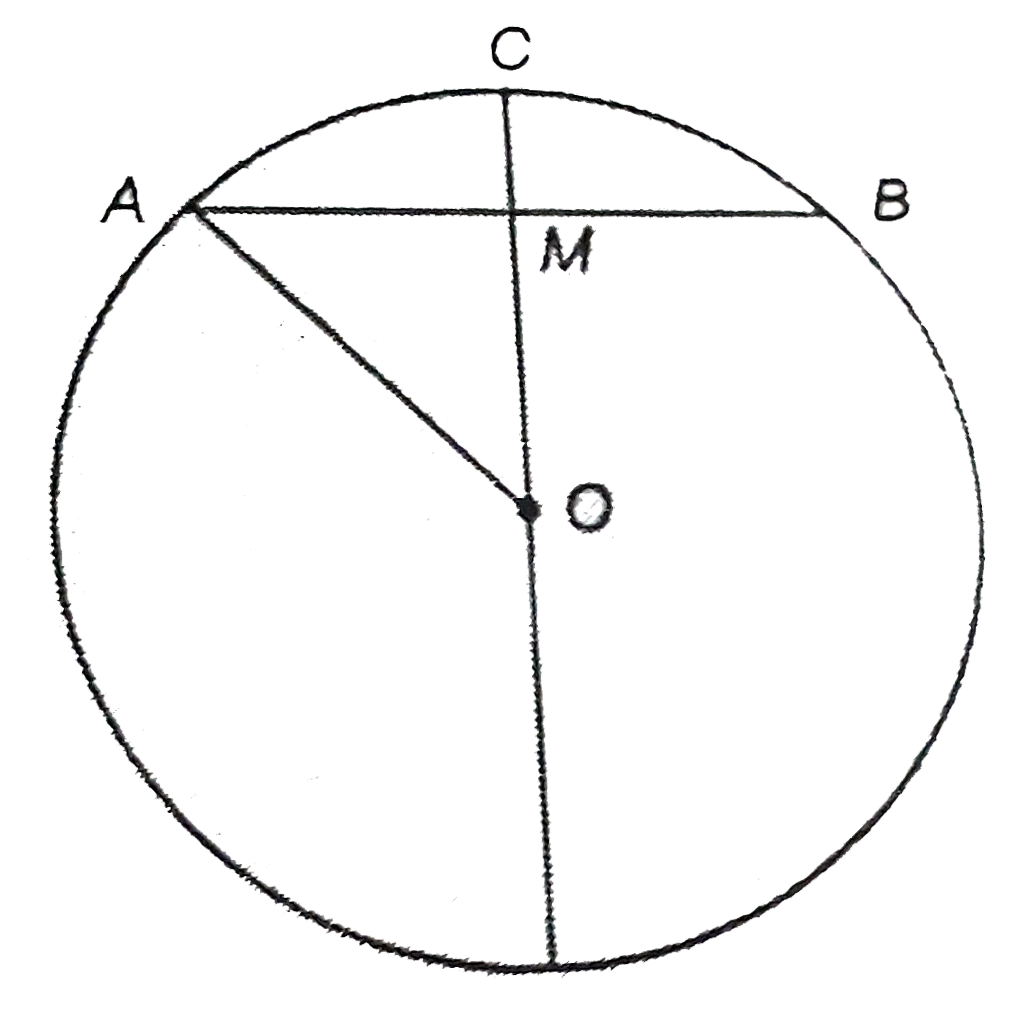
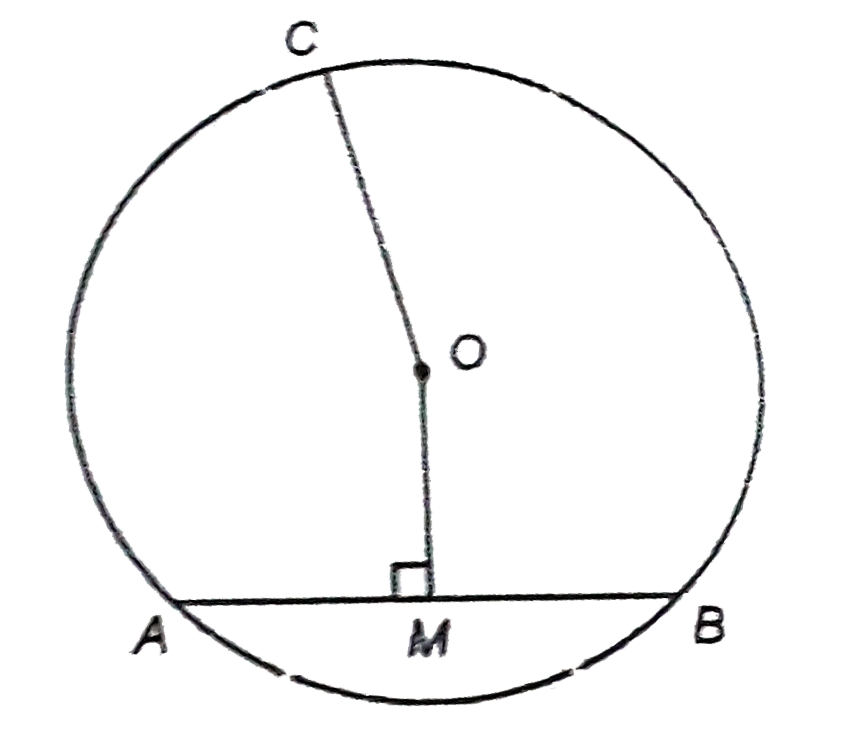
(iii) in the adjoining figure O is the centre of circle. the radius of circle is 17 cm . if OC=8cm, then find the length of chord AB.
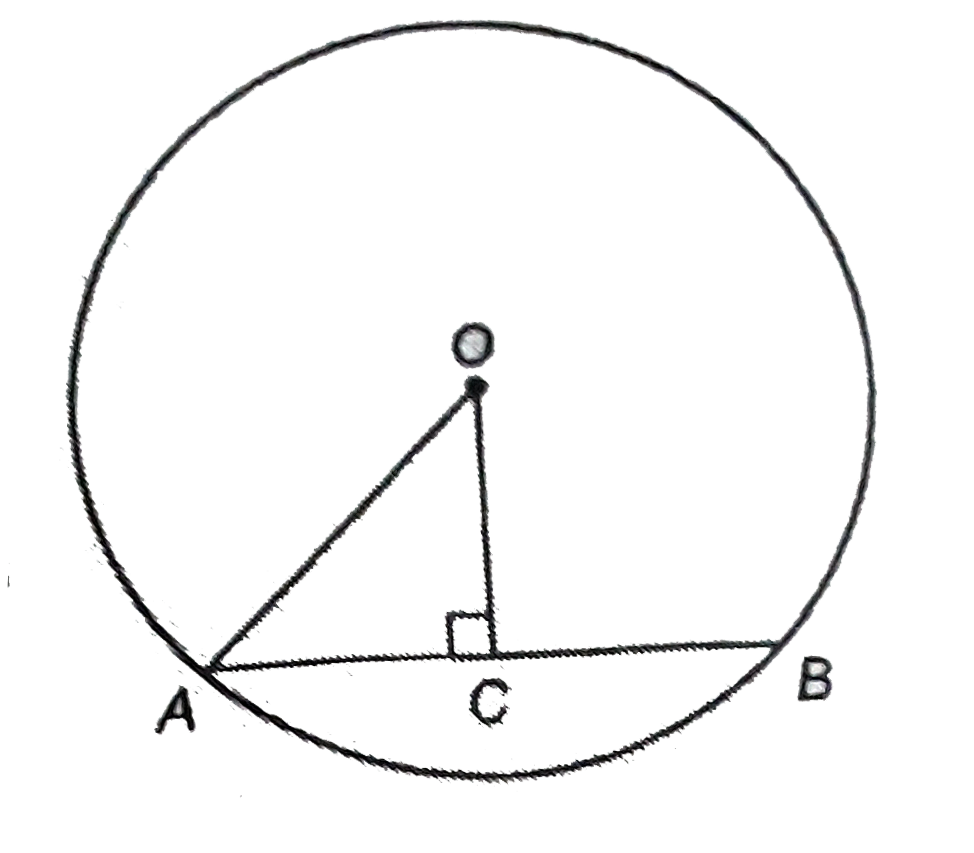
(iv) In the adjoining figure, OM⊥AB, radius OC=5cm and chord AB=8cm.Find the length of OM.
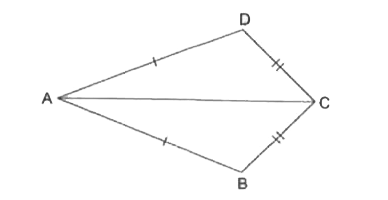
View SolutionIn the adjoining figure,
AB = AD and CB = CD.
Is △ABC≅△ADC ?,
View Solution
