Find the coffiecient of x2y3z4 w in the expansion of (x−y−z+w)10
Video Solution
Text Solution
(X−Y−Z+W)10=ΣP+q+r+s=10n!p!Q!R!S!(x)p(−y)q(−z)r(W)s
we want to get x2y3z4 w this implies that p=2,q = 3 ,r = 4 ,s=1
∴Cofficient of x2y3z4Wis10!2!3!4!1!(−1)3(−1)4=−12600
|
Topper's Solved these Questions
- Solutions of Triangle & Binomial TheoremBook:ALLENChapter:Solutions of Triangle & Binomial TheoremExercise:EXERCISE-I
Explore 29 Videos
- Solutions of Triangle & Binomial TheoremBook:ALLENChapter:Solutions of Triangle & Binomial TheoremExercise:EXERCISE-II
Explore 11 Videos
- Solutions of Triangle & Binomial TheoremBook:ALLENChapter:Solutions of Triangle & Binomial TheoremExercise:Do yourself -6
Explore 4 Videos
- SOLUTION AND PROPERTIES OF TRIANGLEBook:ALLENChapter:SOLUTION AND PROPERTIES OF TRIANGLEExercise:All Questions
Explore 62 Videos
- TRIGNOMETRIC RATIOS AND IDENTITIESBook:ALLENChapter:TRIGNOMETRIC RATIOS AND IDENTITIESExercise:All Questions
Explore 1 Video
Similar Questions
Knowledge Check
The sum of all the coefficients of the terms in the expansion of (x+y+z+w)6 which contain x but not y, is
A36B26C36−26Dnone of theseIn the expansion of (1+x)2(1+y)3(1+z)4(1+w)5, the sum of the coefficient of the terms of degree 12 is :
A61B71C81D91In the expansion of (1+x)2(1+y)3(1+z)4(1+w)5, the sum of the coefficient of the terms of degree 12 is :
A61B71C81D91
Find the totoal number of distnct or
dissimilar terms in the expansion of
(x+y+z+w)n,n∈N
View SolutionIf n is positive integer, then the no. of terms in the expansion of (x+y+z+w)n is
View Solution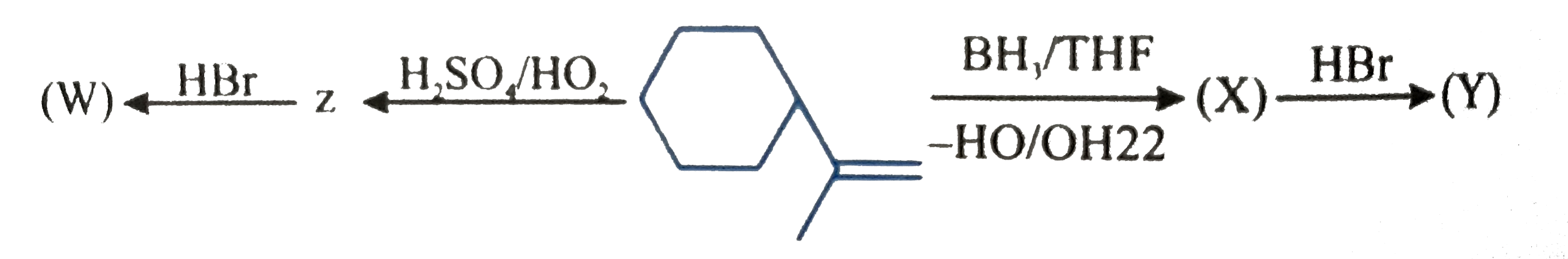
Identify x,y,z and w in the following reaction:
View SolutionFind x+y+z+w
View SolutionFind the number of integral solutions of x+y+z+y<25, where x≻2,y>1,z≥2,w≥0
View Solution