Period T=2pisqrt((l cos theta)/(g))

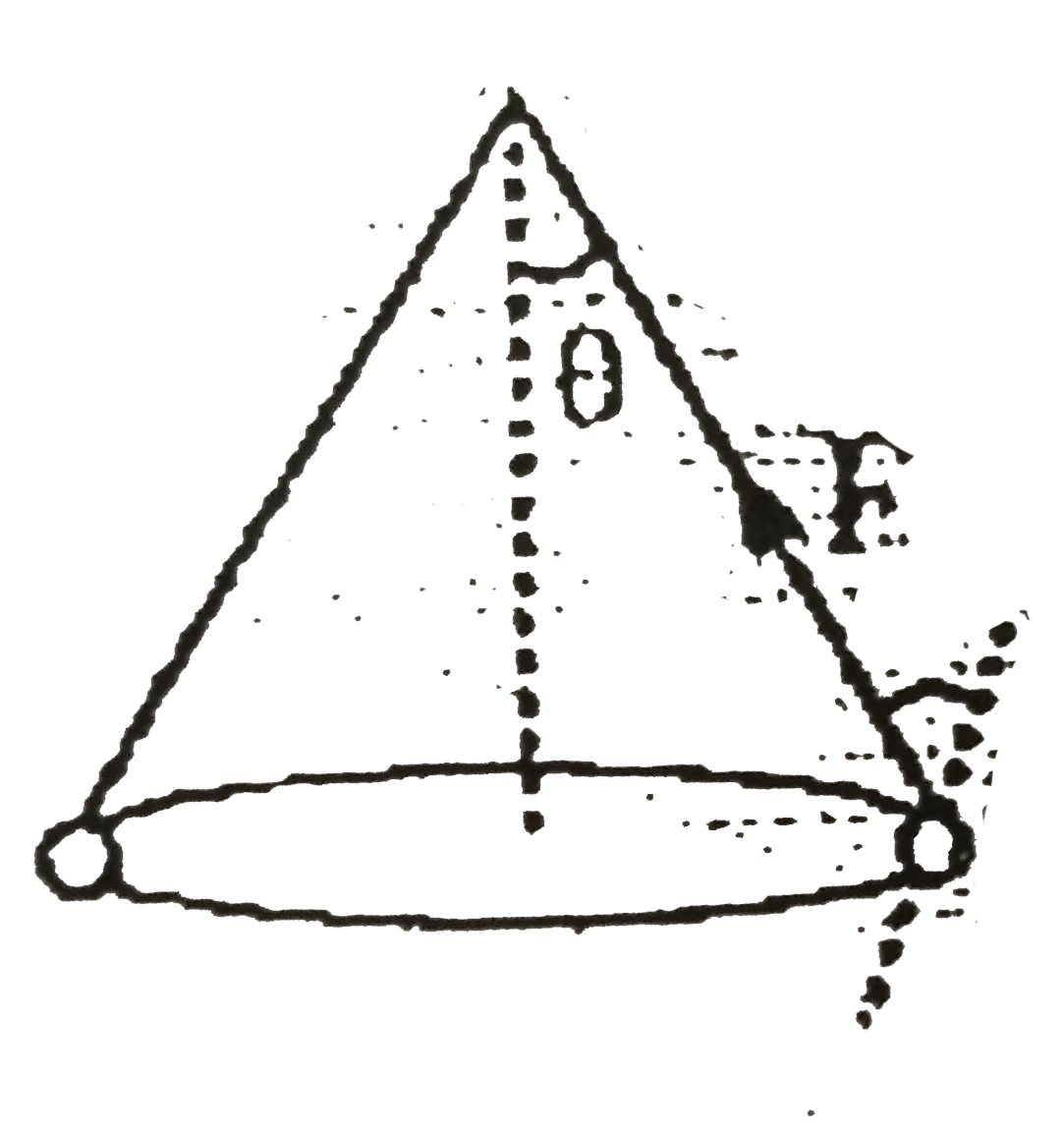
A particle of mass m suspended by a string of length l revolves in a horizontal circle such that $θ$ is the angle which the string makes with vertical. Which of the following statement (s) is/are correct ?

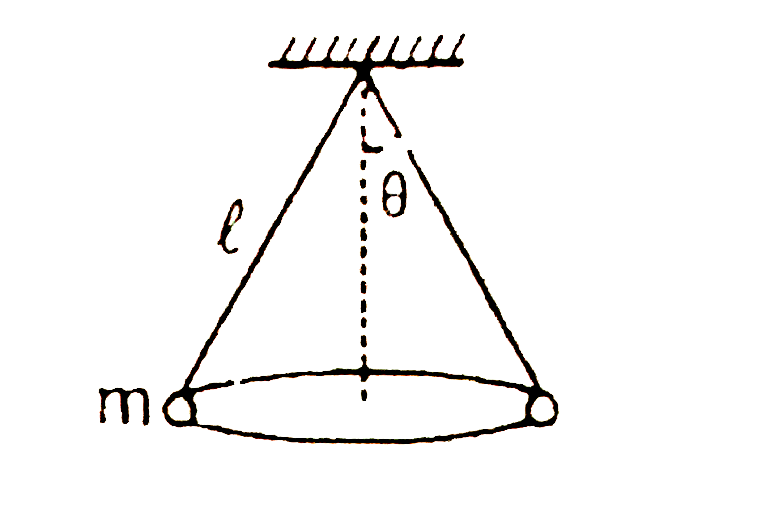ATension in string $= m g cos θ$
BSpeed v of particle $= \sqrt{\frac{l g {sin}^{2} θ}{cos θ}}$
CTime period of revolution $= 2 π \sqrt{\frac{l cos θ}{g}}$
DCentripetal acceleration $= g tan θ$
A rod of mass m is released on smooth horizontal surface making angle $θ$ with horizontal. Then which of the following statement is correct ?
AAcceleration of rod along vertical is less than g
BAcceleration of centre of mass along horizontal is zero.
CAngular acceleration of rod is not constant
DMomentum of the rod along vertical will remain constant
An object of mass M is tied to a string of l and revolve in a horizontal circle .If length is reduced by $l / 2$ , then period is
A $T / \sqrt{2}$
B $2 \sqrt{2} T$
C $T / 4$
D $2 T$

A particle of mass m suspended by a string of length l revolves in a horizontal circle ( $θ$ is the angle the string makes with vertical). Which of the following statement(s) is // are correct?