The resultant of 2 forces of magnitude 1N and 13N is 6√5N. Find the tangent of the angle made by resultant with 1N force.
Let P=1N
Q=13N ltbgt R=6√5N
By Parallelogram rule of vector addition
R2=P2+Q2+2PQcosθ
(6√5)2=(1)2+(13)2+2×1×13cosθ
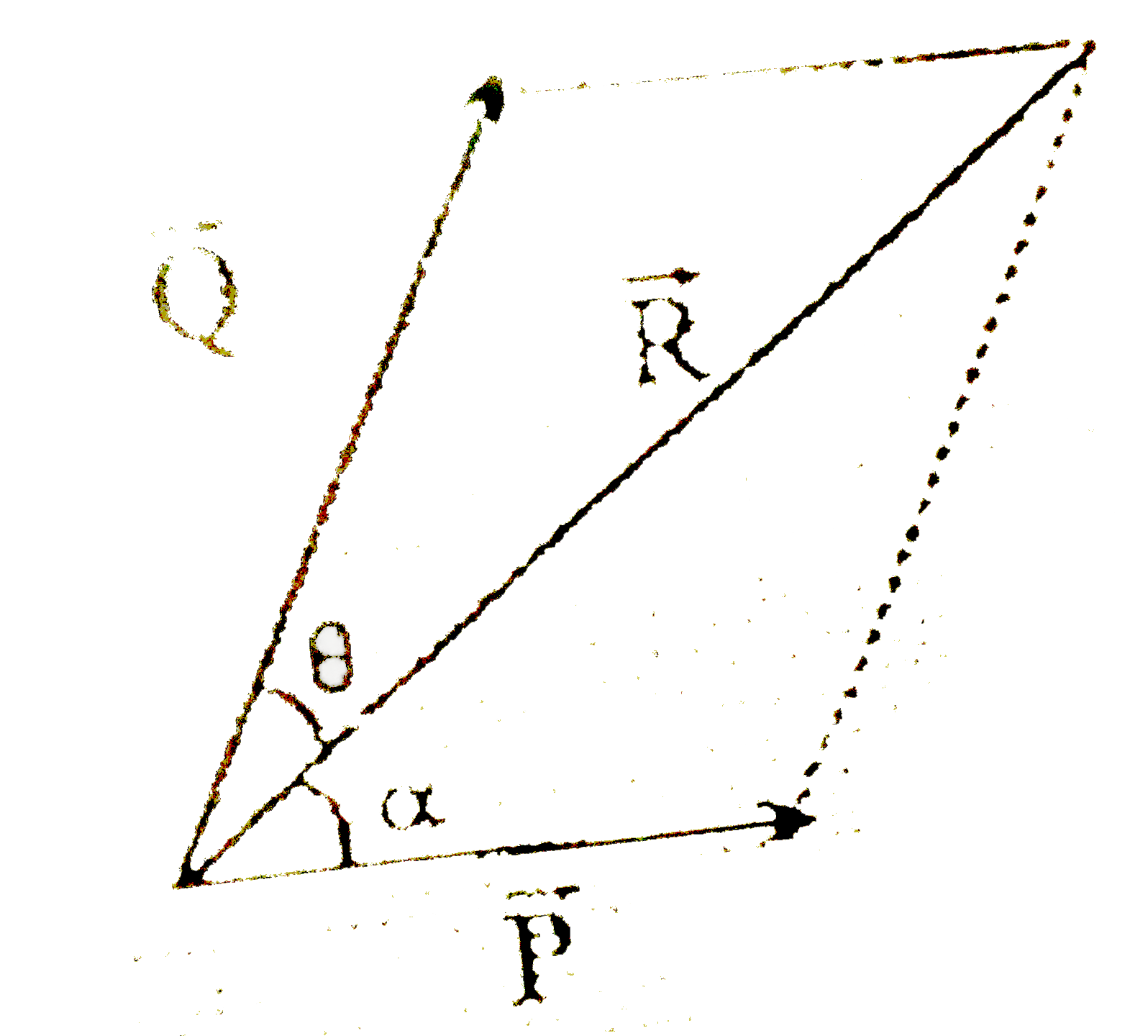
θ=cos−1(513) . . . .(1)
tanα=QsinθP+Qcosθ=13sinθ1+13cosθ
13×12131+13×513=126=2
α=tan−12 . . . .(2)
Ans. Angle between given vectors is
θ=cos−1(513)
Angle of resultant with 1Nforce<br>=alpha-tan^(-1)(2)
Topper's Solved these Questions
- TEST PAPERSBOOK - BANSALCHAPTER - TEST PAPERSEXERCISE - PHYSICS PART (A)
32 Videos
- TEST PAPERSBOOK - BANSALCHAPTER - TEST PAPERSEXERCISE - PHYSICS PART (B)
3 Videos
- TEST PAPERSBOOK - BANSALCHAPTER - TEST PAPERSEXERCISE - PHYSICS PART- C
4 Videos
- SEMICONDUCTORSBOOK - BANSALCHAPTER - SEMICONDUCTORSEXERCISE - CBSE Question
32 Videos
Similar Questions
The resultant of two forces of magnitude 5 N and 3 N trisects the angle between them. Calculate the angle between them.
View SolutionThe resultant of two forces of magnitude 12N and 3N on a body is 13.74N. Find the angle between the two. What is the direction of the resultant force w.r.t. 12N force?
View Solution
Knowledge Check
The resultant force of 5 N and 10 N can not be
A12 NB8 NC4 ND5 NThe resultant force of 5 N and 10 N cannot be
A12 NB8 NC4 ND5 NThe resultant of two forces of 10N and 5N can never be :-
A12NB5NC10ND4N
The resultant of two forces of magnitudes 4N and 4√2 N makes 90∘ with the smaller force. Then angle between those two forces is ?
View SolutionTwo perpendicular forces of magnitudes 10 N and 5 N act at a point. Find their resultant.
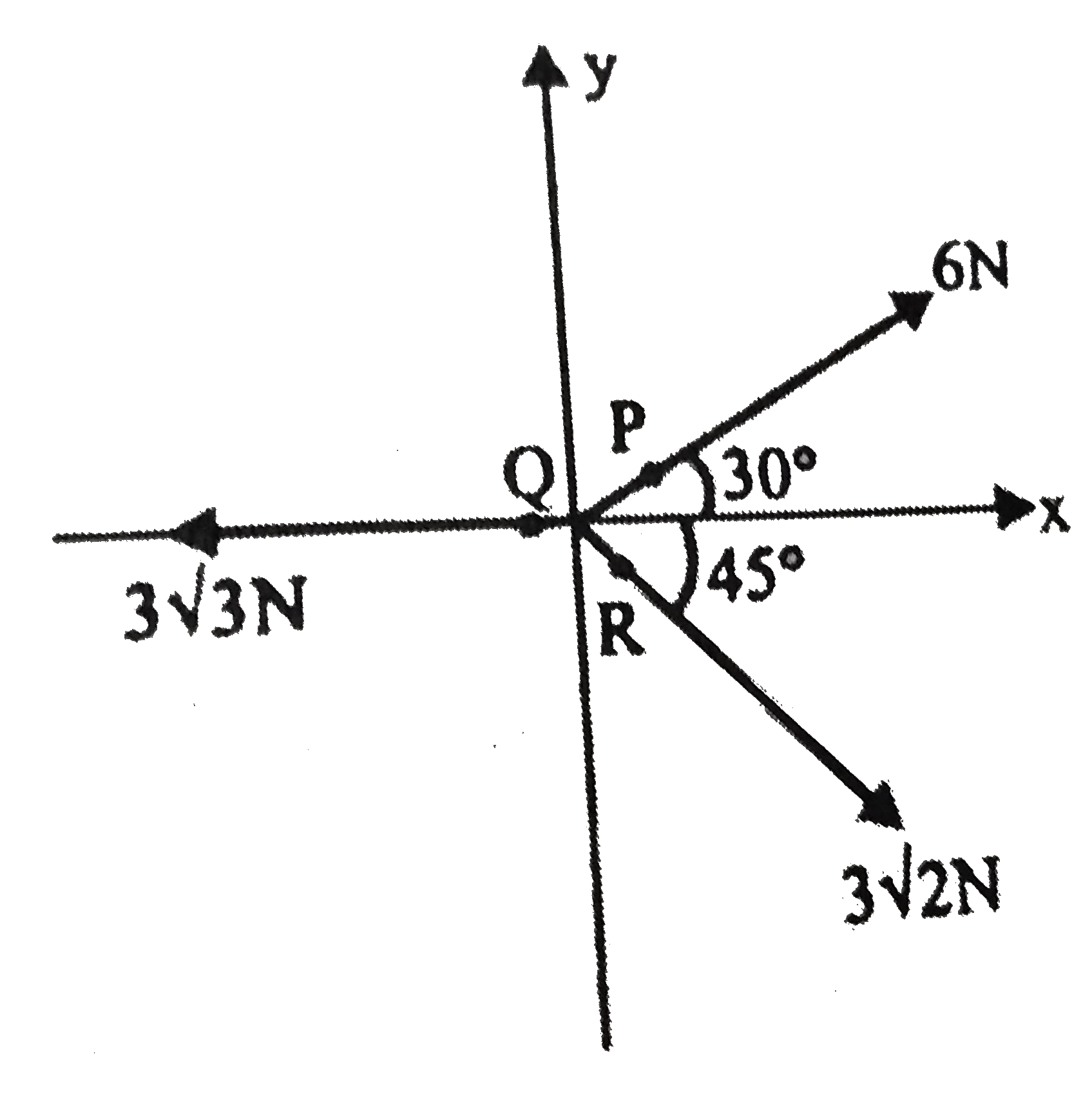
View SolutionThree ant P,Q and R are pulling a grain with forces of magnitude 6N,3√3N and 3√2N as shown in the figure. Find the magnitude of resultant force (in N ) acting on the grain .
View SolutionTwo forces of magnitude 1 N and 13 N resultant of magnitude 6√5N, then that is the angle between two forces.
View SolutionTwo forces each of magnitude 2N, act at an angle of 60∘. What is the magnitude of the resultant force ?
View Solution