In each of the following, check how the points A,B and C are situated.
(i) $A (- 2, 2), B (8, - 2), C (- 4, - 3)$
(ii) $A (- a, - b), B (a, b), C (a^{2}, a b), a > 1$
(iii) $A (4, 0), B (- 1, - 1), C (3, 5)$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:(i) ABC is triangle right angled at A
(ii) collinear points
(iii) ABC is right angled isoceles triangle

(i) $A (- 2, 2), B ((8, - 2), C (- 4, - 3)$
$A B = \sqrt{{(8 - (- 2))}^{2} + {(- 2 - 2)}^{2}}$
$= \sqrt{100 + 16} = 2 \sqrt{29}$
$B C = \sqrt{{(8 - (- 4))}^{2} + {(- 2 - (- 3))}^{2}}$
$= \sqrt{144 + 1} = \sqrt{5} = \sqrt{29}$
$C A = \sqrt{{(- 2 - (- 4))}^{2} + {(2 - (- 3))}^{2}}$
$= \sqrt{4 + 25} = \sqrt{29}$
Thus, $A B^{2} + C A^{2} = B C^{2}$
So, triangle is right angled at A.
(ii) $A (- a, - b), B (a, b), C (a^{2}, a b)$
$A B = \sqrt{{(2 a)}^{2} + {(2 b)}^{2}} = 2 \sqrt{a^{2} + b^{2}}$
$B C = \sqrt{{(a^{2} - a)}^{2} + b^{2} {(a - 1)}^{2}} = (a - 1) \sqrt{a^{2} + b^{2}}$
$A C = \sqrt{{(a^{2} + a)}^{2} + b^{2} {(a + 1)}^{2}} = (a + 1) \sqrt{a^{2} + b^{2}}$
Thus, $A B + B C = A C$ .
So, points are collinear.
(iii) $A (4, 0), B (- 1, - 1), C (3, 5)$ .
$A B = \sqrt{{(- 1 - 4)}^{2} + b^{2} {(- 1 - 0)}^{2}} = \sqrt{25 + 1} = \sqrt{26}$
$B C = \sqrt{{(3 + 1)}^{2} + b^{2} {(5 + 1)}^{2}} = \sqrt{16 + 36} = \sqrt{52}$
$C A = \sqrt{{(4 - 3)}^{2} + b^{2} {(0 - 5)}^{2}} = \sqrt{1 + 25} = \sqrt{26}$
Thus, $A B = C A and B C 62 = A B^{2} + C A 62$
So, triangle ABC is right angled isoceles.

Updated on:21/07/2023

|

Class 12 MATHS THREE DIMENSIONAL GEOMETRY

Topper's Solved these Questions

Similar Questions

Knowledge Check

The points
(i) $A (0, - 1), B (2, 1), C (0, 3), D (- 2, 1)$
are the vertices of a
Asquare
Brectangle
Cparallelogram
Dnone
Match the following columns.

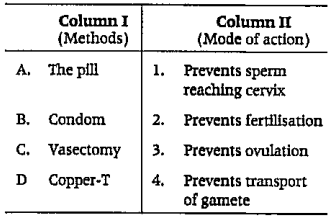
a) A-3, B- 1, C- 4 , D- 2 .
b) A- 4 , B- 1 , C- 2, D- 3.
c) A- 2 , B- 3, C- 1, D- 4.
d) A- 3, B- 4, C-1, D- 2.
A
B
C
D

CENGAGE-COORDINATE SYSYEM -Exercise 1.2

Home
Ask Doubt
Profile