Two friends visit a restaurant randomly during 5 pm to 6 pm . Among the two, whoever comes first waits for 15 min and then leaves. The probability that they meet is :
14
116
716
916
The correct Answer is:C
Step by step video, text & image solution for Two friends visit a restaurant randomly during 5 pm to 6 pm . Among the two, whoever comes first waits for 15 min and then leaves. The probability that they meet is : by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- PROBABILITY BOOK - VK JAISWALCHAPTER - PROBABILITY EXERCISE - Exercise-2 : One or More than One Answer is/are Correct
4 Videos
- PROBABILITY BOOK - VK JAISWALCHAPTER - PROBABILITY EXERCISE - Exercise-3 : Comprehension Type Problems
12 Videos
- PERMUTATION AND COMBINATIONS BOOK - VK JAISWALCHAPTER - PERMUTATION AND COMBINATIONS EXERCISE - Exercise-5 : Subjective Type Problems
13 Videos
- QUADRATIC EQUATIONS BOOK - VK JAISWALCHAPTER - QUADRATIC EQUATIONS EXERCISE - EXERCISE (SUBJECTIVE TYPE PROBLEMS)
45 Videos
Similar Questions
Two friends visit a restaurant randomly during 5pm to 6pm. Among the two, whoever comes first waits for 20 minutes and then leaves. The probability that they meet is
View SolutionTwo persons A and B agree to meet at a place between 11 to 12 noon. The first one to arrive waits for 20 minutes and then leave. if the time of their arrival be independent and at random, then the probabity that A and B meet is:
View Solution
Knowledge Check
There are some experiment in which the outcomes cannot be identified discretely. For example, an ellipse of eccentricity 2√2/3 is inscribed in a circle and a point within the circle is chosen at random. Now, we want to find the probability that this point lies outside the ellipse. Then, the point must lie in the shaded region shown in Figure. Let the radius of the circle be a and length of minor axis of the ellipse be 2b. Given that
1−b2a2=89orb2a2=19
Then, the area of circle serves as sample space and area of the shaded region represents the area for favorable cases. Then, required probability is
p=Area of shaded regionArea of circle
=πa2−πabπa2=1−ba=1−13=23
Now, answer the following questions.
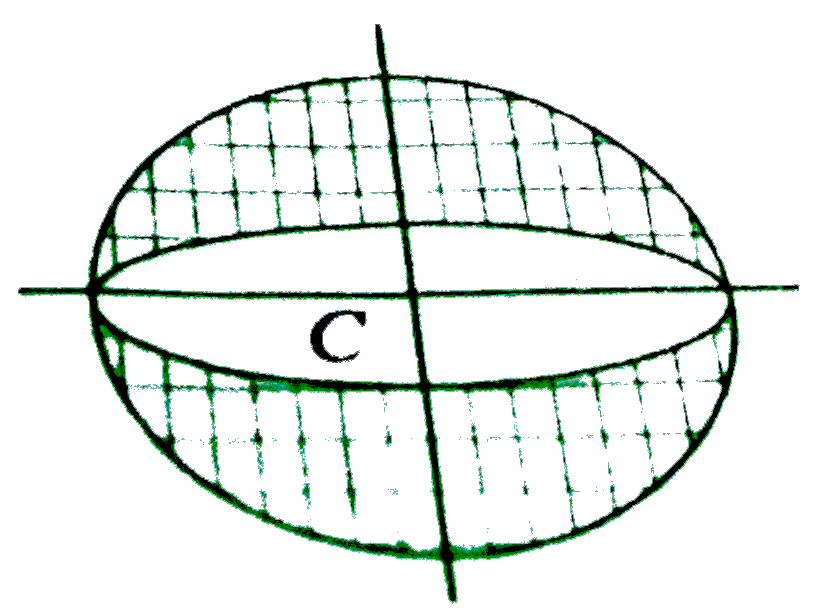
Two persons A and B agree to meet at a place between 5 and 6 pm. The first one to arrive waits for 20 min and then leave. If the time of their arrival be independant and at random, then the probability that A and B meet isA1/3B1/3C2/3D5/9Two persons ordered tea in a restaurant and waited for a friend to arrive. The first person mixed hot tea and cold milk in the cup and wated for the fried and the second mixed hot tea and hot milk in the cup after the arrival of the friend. Milk in the cup after the arrival of the friend. If the temperature of the two cups of tea are T1andT2 respectively, then.
AT1<T2BT1=T2CT1>T2DT1≥T2Two numbers are selected randomly from the set S={1,2,3,4,5,6} without replacement. The probability that minimum of the two numbers is less than 4, is
A1/15B14/15C1/5D4/5
A friendly hockey match among Aakashians played from 3pm to 5pm. Shekhar arrives to see the match (not before the match starts). Find the probability that the will miss the only goal of the match which takes place at the 15th minute of the match.
View SolutionThere are two urns containing 5 red and 6 white balls and 3 red and 7 white balls respectively. If two balls are drawn from the first urn without replacement and transferred to the second urn and then a draw of another two balls is made from it, what is the probability that both the balls drawn are red?
View SolutionA bag contains 6 red balls and 10 green balls. 3 balls are drawn from it one by one randomly without replacement. If the third ball drawn is red, then the probability that first two balls are green is:
View SolutionIn a bag, there are 15 red and 5 white balls. Two balls are drawn in succession without replacement. The first ball drawn is found to be red. The probability that second ball is also red is equal to (A) 310 (B) 710 (C) 519 (D) 1419
View SolutionTwo numbers are selected randomly from the set S={1,2,3,4,5,6} without replacement one by one. The probability that minimum of the two numbers is less than 4 is (a) 115 (b) 1415 (c) 15 (d) 45
View Solution
