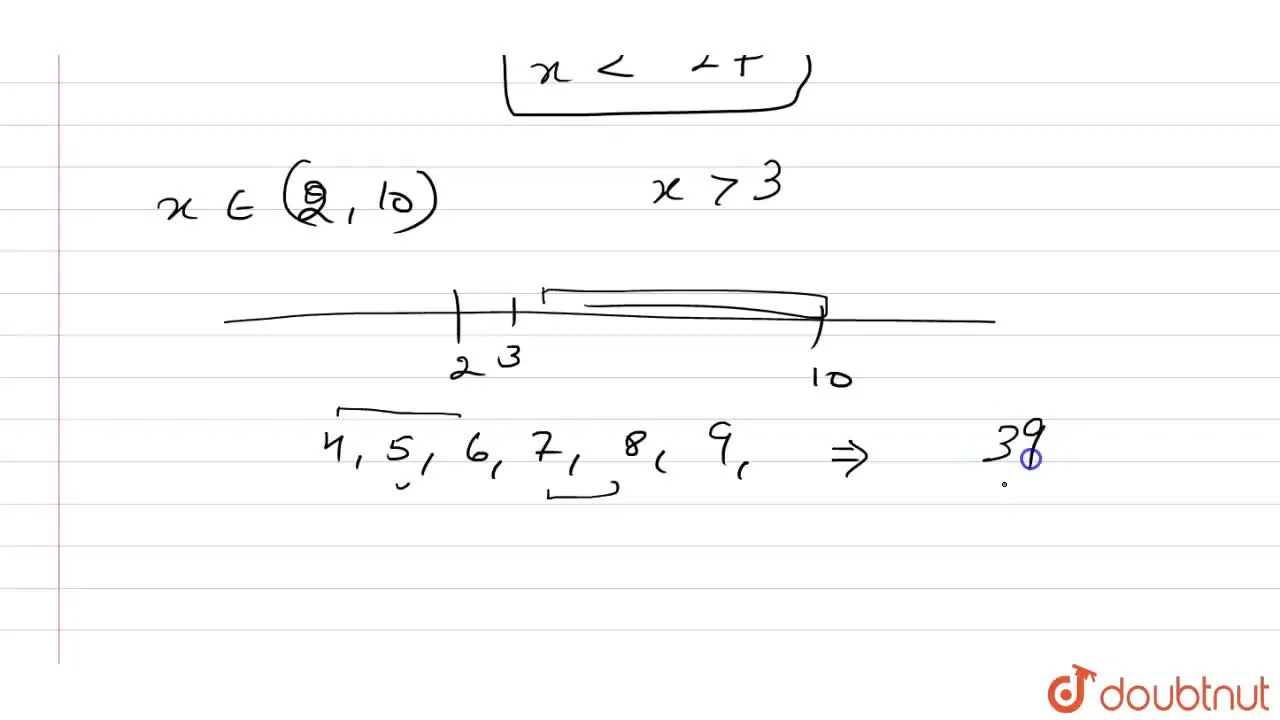Find the sum of all integers satisfying the inequalities log5(x−3)+12log53<12log5(2x2−6x+7)andlog3x+log√3x+log13x<6
The correct Answer is:0039
Step by step video, text & image solution for Find the sum of all integers satisfying the inequalities log_(5)(x-3)+1/2log_(5)3lt1/2log_(5)(2x^(2)-6x+7) and log_(3)x+log_(sqrt3)x+log_(1/3)xlt6 by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Related Playlists
Similar Questions
Consider the inequalities log5(x−3)+12log53<12log5(2x2−6x+7)andlog3x+log√3x+log13x<6.
View SolutionNumber of integers satisfying the inequality log(x+3)(x2−x)<1 is
View SolutionNumber of integers satisfying the inequality (log)12|x−3|≻1 is.....
View SolutionNumber of integers ≤ 10 satisfying the inequality 2log12(x−1)≤13−1logx2−x8 is :
View SolutionSum of the values of x satisfying the equation log3(5x−6)logx√3=1 is
View SolutionFind the number of integers which do not satisfy the inequality log12(x+5)2>log12(3x−1)2.
View SolutionThe sum of all the roots of the equation log2(x−1)+log2(x+2)−log2(3x−1)=log24
View SolutionNumber of integers ≤10 satisfying the inequality 2(log)12(x−1)≤13−1(log)x2−x8 is............
View SolutionFind the sum of all solutions of the equation 3(log9x)2−92log9x+5=3√3
View SolutionSum of integers satisfying √(log)2x−1−12(log)2(x3)+2>0 is......
View Solution