Consider a ΔABC whose sides AB,BCandCA are represented by the straight lines 2x+y=0,x+py=qandx−y=3 respectively. The point P is (2,3). If P is orthocentre,then find the value of (p+q) is
The correct Answer is:50
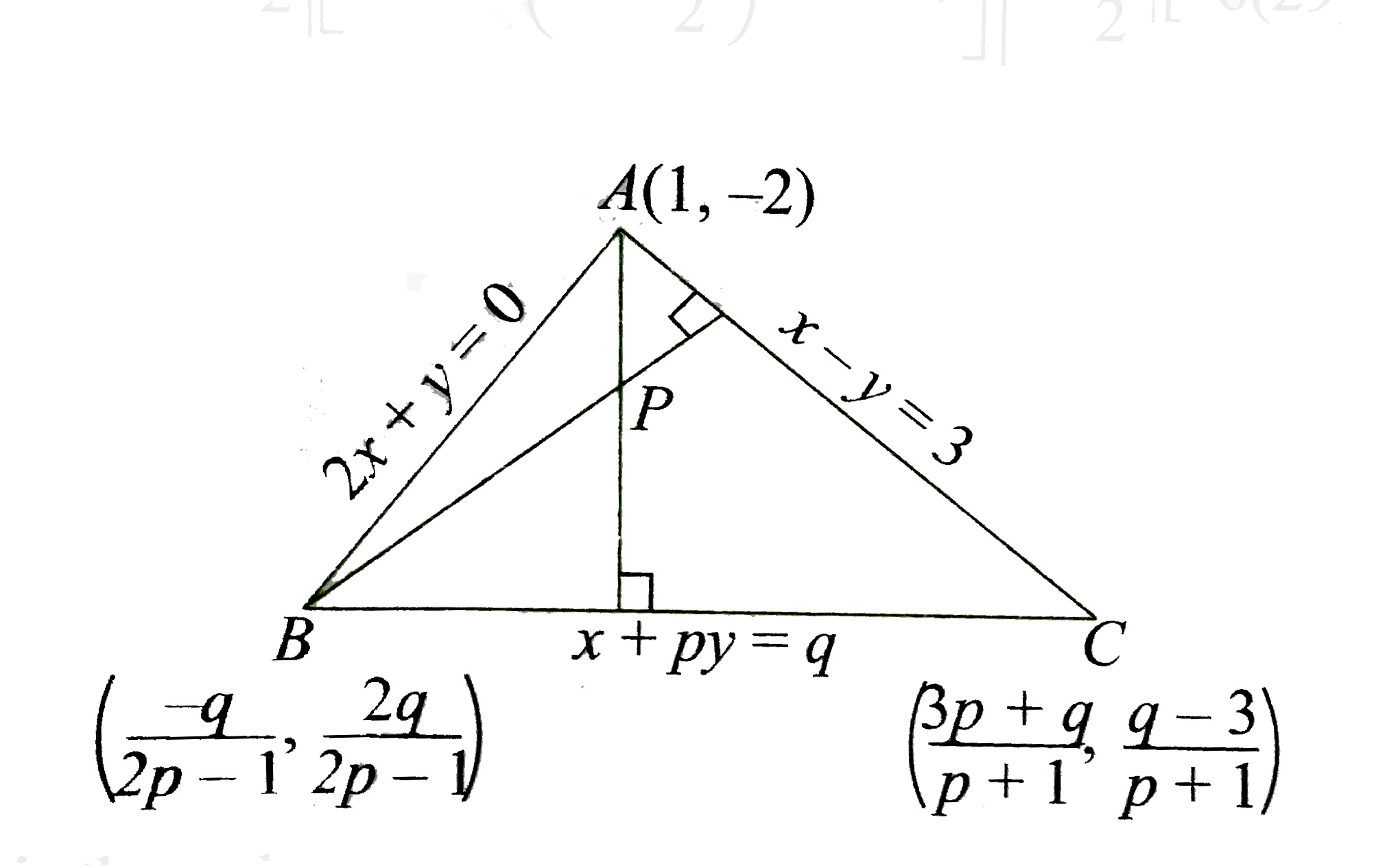
P is the orthocenter. Therefore,
AP⊥BC
or (−1p)(3+22−1)=−1
or 5p=1 or p=5
Since BP⊥AC, we have
27−2q18+q=−1
or q =27+18
or q = 45
∴p+q=5+45=50
Topper's Solved these Questions
- STRAIGHT LINESBOOK - CENGAGECHAPTER - STRAIGHT LINESEXERCISE - JEE Main Previous Year
9 Videos
- STRAIGHT LINESBOOK - CENGAGECHAPTER - STRAIGHT LINESEXERCISE - JEE Advanced Previous Year
4 Videos
- STRAIGHT LINESBOOK - CENGAGECHAPTER - STRAIGHT LINESEXERCISE - Exercise (Matrix)
8 Videos
- STRAIGHT LINEBOOK - CENGAGECHAPTER - STRAIGHT LINEEXERCISE - Multiple Correct Answers Type
8 Videos
- THEORY OF EQUATIONSBOOK - CENGAGECHAPTER - THEORY OF EQUATIONSEXERCISE - JEE Advanced Previous Year
9 Videos
Similar Questions
Consider a ΔABC whose sides AB,BCandCA are represented by the straight lines 2x+y=0,x+py=qandx−y=3 respectively. The point P is (2,3). If P is orthocentre,then find the value of (p+q) is
View SolutionConsider a △ABC whose sides BC, CA and AB are represented by the straight lines x−2y+5=0,x+y+2=0and8x−y−20=0 respectively. The area of △ABC equals
View Solution
Knowledge Check
The equations of the sides AB and CA of a ΔABC are x+2y=0 and x−y=3 respectively. Given a fixed point P(2, 3).
Q. If P be orthocentre of ΔABC then equation of side BC is :Ay+5=0By−5=0C5y+1=0D5y−1=0The value of p for which the lines 2x+y−3=0,3x−y−2=0 and x−py+5=0 may intersect at a point is
A2B3C5D6The equations of the sides AB and CA of a ΔABC are x+2y=0 and x−y=3 respectively. Given a fixed point P(2, 3).
Q. Let the equation of BC is x+py=q. Then the value of (p+q) if P be the centroid of the ΔABC is :A14B-14C22D-22
In the adjoining figure the sides AB,BC and CA of the triangle ABC are 2x+y=0,x+py=q and x−y=3 respectively. The point P inside the triangle is (2,3) then match the entries of column I and column II.

View SolutionIf the straight lines 3x+2y-3=0,5x+2py-7=0 are parallel.find the value of p
View SolutionIf the equations px+2y−3=0,x+3y−4=0 and px2+3y2+3xy+(q−3)x−3y−1=0(p≠23) has a unique solution then find the value of (p+q).
View SolutionIf a parabola touches the lines y=xandy=−x at P(3,3)andQ(2,−2) respectively, then
View SolutionFind the value of P ,if the straight lines x+P=0,y+2=0 and 3x+2y+5=0 are concurrent.
View Solution
