Let ABCD be a parallelogram the equation of whose diagonals are AC:x+2y=3; BD: 2x + y = 3. If length of diagonalAC=4 units and area of ABCD=8 sq. units. (i) The length of the other diagonal is (ii) the length of side AB is equal to
A
10/3
B
2
C
20/3
D
none of these
Video Solution
Text Solution
The correct Answer is:C
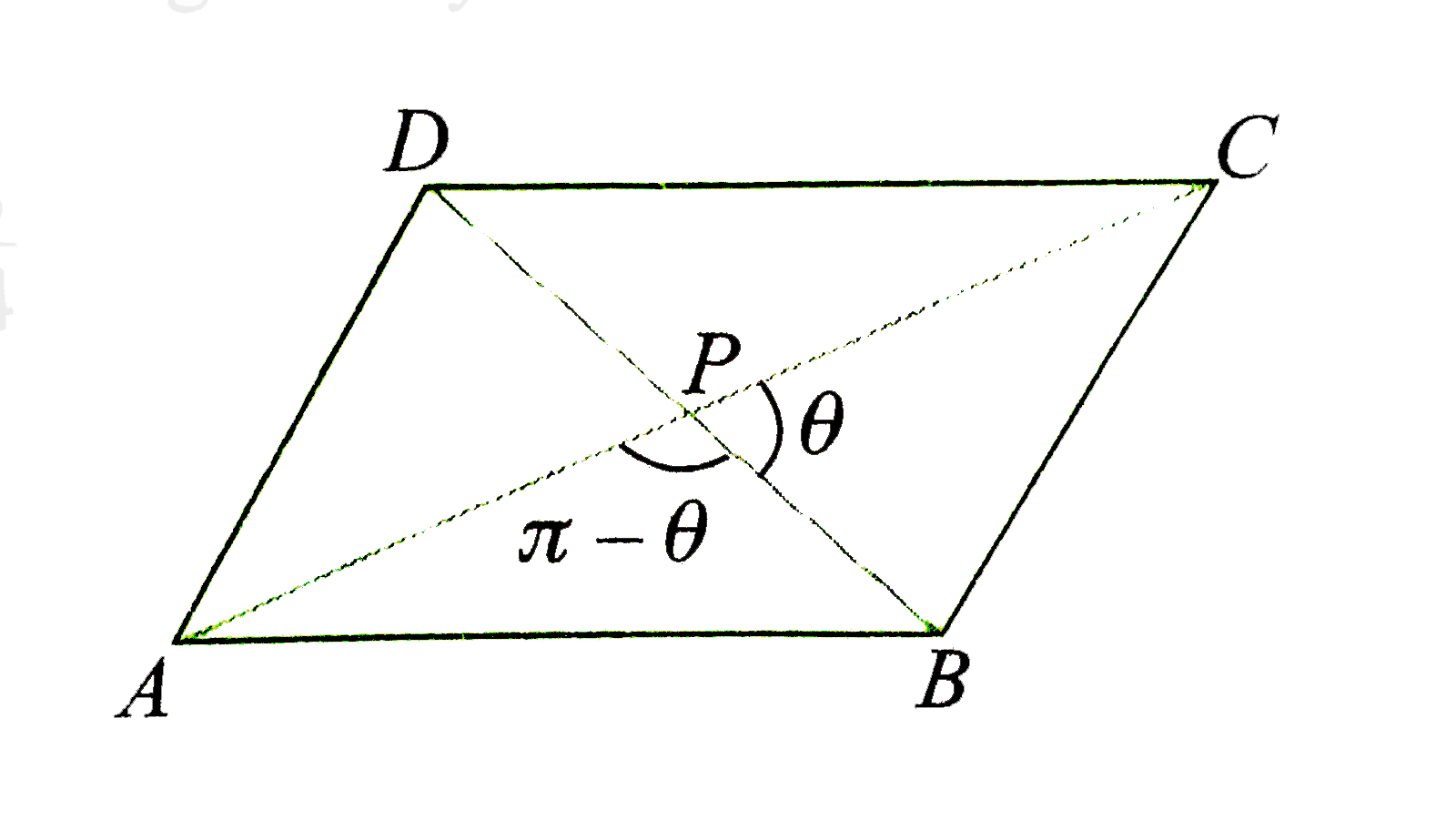
Angle between the diagonal is given by
tan θ=∣∣
∣∣−12+21+1∣∣
∣∣=34
or sinθ=35
Area of ΔCPB is
12×PC×PBsinθ=2
or PB=103
or BD=203
|
Updated on:21/07/2023
Topper's Solved these Questions
- BOOK - CENGAGECHAPTER - STRAIGHT LINESEXERCISE - Exercise (Matrix)8videos
STRAIGHT LINES
- BOOK - CENGAGECHAPTER - STRAIGHT LINESEXERCISE - Exercise (Numerical)13videos
STRAIGHT LINES
- BOOK - CENGAGECHAPTER - STRAIGHT LINESEXERCISE - Exercise (Multiple)30videos
STRAIGHT LINES
- BOOK - CENGAGECHAPTER - STRAIGHT LINEEXERCISE - Multiple Correct Answers Type8videos
STRAIGHT LINE
- BOOK - CENGAGECHAPTER - THEORY OF EQUATIONSEXERCISE - JEE Advanced Previous Year9videos
THEORY OF EQUATIONS
Similar Questions
Knowledge Check
- Question 1 - Select One
Let ABCD be a parallelogram whose equations for the diagonals AC and BD are x+2y=3 and 2x+y=3, respectively.
The length of side AB is equal toA2√58/3B4√58/9C3√58/9D4√58/9 - Question 2 - Select One
If the length of the diagonal AC of a square ABCD is 5.2 cm, then the area of the square is :
A15.12 sq. cmB13.52 sq. cmC12.62 sq. cmD10.00 sq. cm. - Question 3 - Select One
If the length of the diagonal AC of a square ABCD is 5.2 cm, then the area of the square is :
A15.12 sq . CmB13.52 sq. cmC12.62 sq. cmD10 sq. cm
ABCD is a parallelogram, AB=14cm,BC=18cm and AC=16cm Find the length of the other diagonal?
View SolutionIn a rhombus ABCD,∠A=60∘andAB=6cm. The length of the diagonal BD is equal to
View SolutionABCD is a parallelogram. E & F are centroid of triangles ABD and BDC respectively. Find the length of EF if length of diagonal AC = 12 cm.
View SolutionLet ABCD be a convex quadrillateral with AB= a, BC= b, CD= c and DA=d. Suppose a2+b2+c2+d2=ab+bc+cd+da and the area of ABCD is 60 square units. If the length of one of the diagonals is 30 units, determine the length of the other diagonal.
View SolutionABCD is a rhombus with side 6 cm. If one of the diagonals is of 3√3 cm then length of other diagonal is
View Solution

