If $t$ is a real number satisfying the equation $2 t^{3} - 9 t^{2} + 30 - a = 0,$ then find the values of the parameter $a$ for which the equation $x + \frac{1}{x} = t$ gives six real and distinct values of $x$ .

Video Solution

Text Solution

Verified by Experts

We have, $2 t^{3} - 9 t^{2} + 30 - a = 0$ .
Any real root $' t_{0}'$ of this equation gives two real and distinct values of x from $x + \frac{1}{x} = t$ if $| t_{0} | > 2$ .
Thus, we need to find the condition for the equation in 't' to have three real and distinct roots, none of which lies in [-2, 2].
Let $f (t) = 2 t^{3} - 9 t^{2} + 30 - a$
$f' (t) = 6 t^{2} - 18 t = 0 \Rightarrow t = 0, 3$

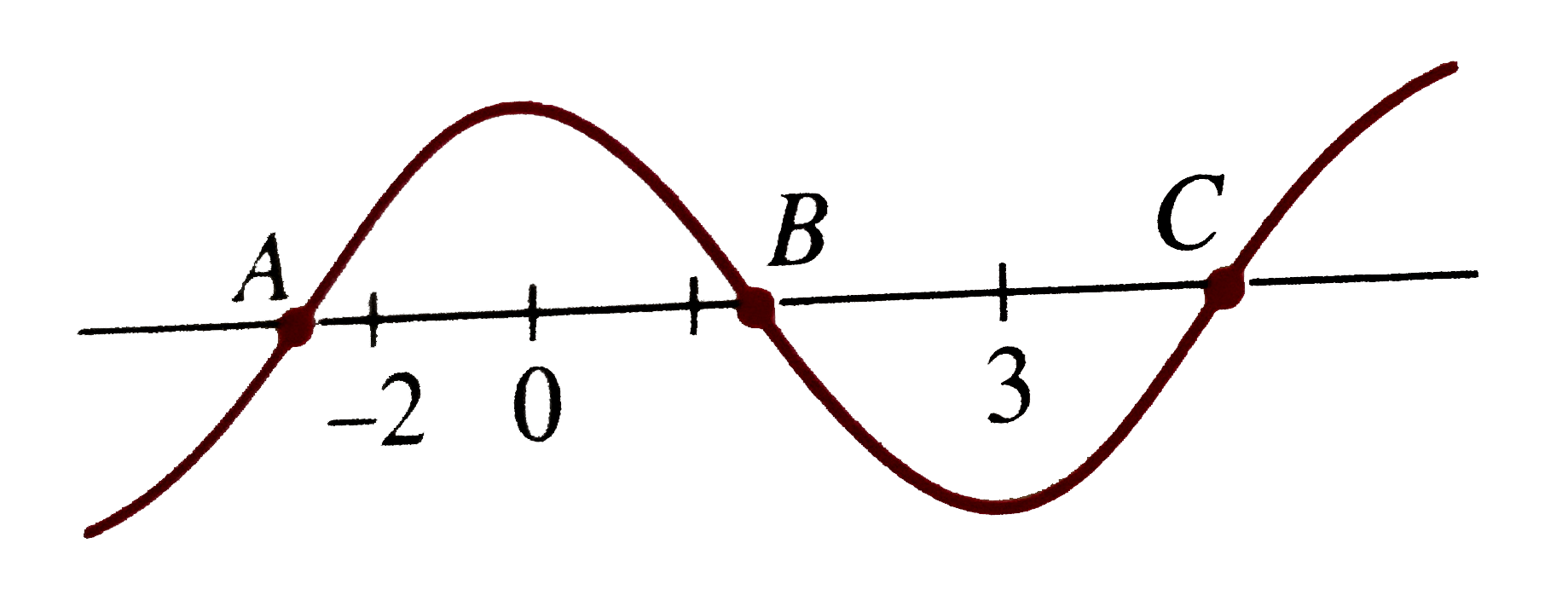
So the equation $f (t) = 0$ has three real and distinct roots if $f (0) \cdot f (3) < 0$
$\Rightarrow (30 - a) (54 - 81 + 30 - a) < 0 \Rightarrow (30 - a) (30 - a) < 0$
$\Rightarrow (a - 3) (a - 30) < 0 \Rightarrow a \in (3, 30) (i)$
Also, none of the roots lies in [-2,2] if $f (- 2) > 0$ and $f (2) > 0 (. ∵ - 2, 2 \in (A, B))$
$- 6 - 36 + 30 - a > 0$ and $16 - 36 + 30 - a > 0$
$- 22 - a > 0$ and $10 - a > 0 \Rightarrow a + 22 < 0$ and $a - 10 < 0$
$\Rightarrow a < - 22$ and $a < 10$
$\Rightarrow a < - 22 (i i)$
From (i) and (ii), no real value of a exists.

Updated on:21/07/2023