Doubtnut Promotions Sticky

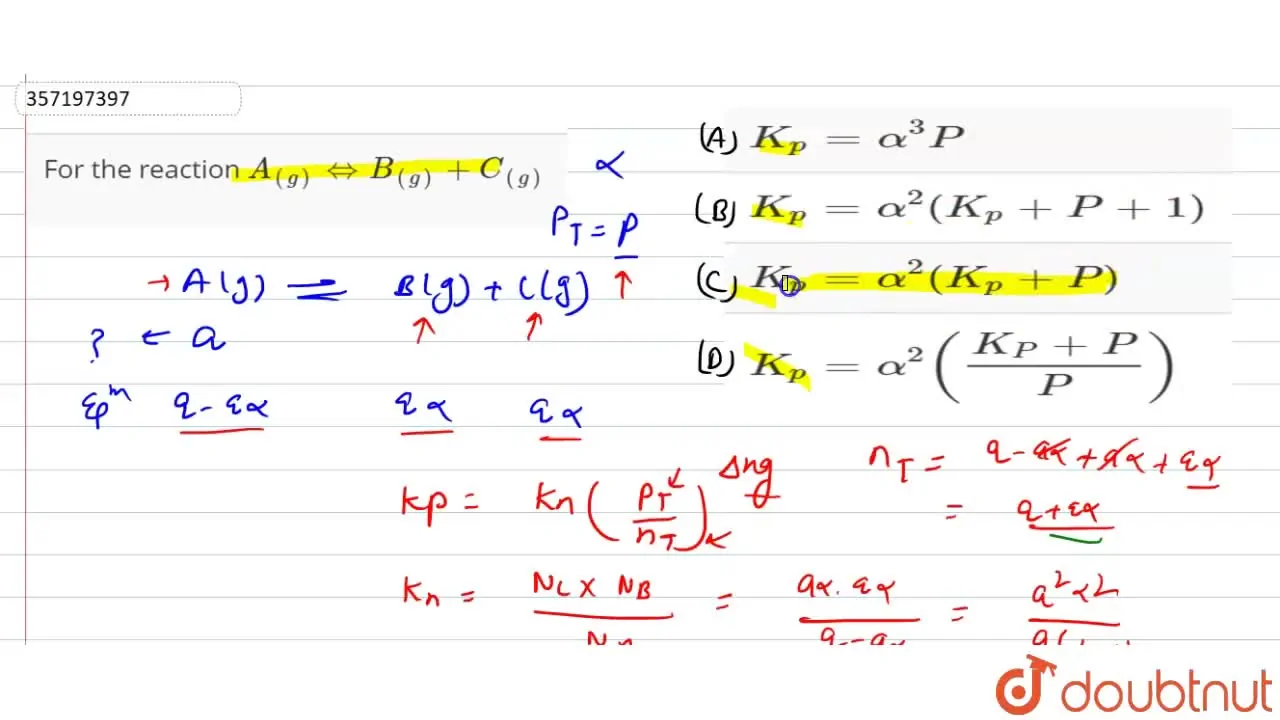
For the reaction A(g)⇔B(g)+C(g)

A

Kp=α3P

B

Kp=α2(Kp+P+1)

C

Kp=α2(Kp+P)

D

Kp=α2(KP+PP)

Video Solution
Doubtnut Promotions Banner
Text Solution
Verified by Experts

The correct Answer is:C

|
Answer

Step by step video, text & image solution for For the reaction A_((g)) hArr B_((g)) + C_((g)) by Chemistry experts to help you in doubts & scoring excellent marks in Class 12 exams.

Updated on:21/07/2023
Class 12CHEMISTRYATOMIC STRUCTURE

Similar Questions

Knowledge Check

  • Question 1 - Select One

    For the reaction A(g)+B(g)⇔C(g)+D(g), the degree of dissociation α would be –

    A√K√K+1
    B√K+1
    C√K±1
    D√K−1
  • Question 2 - Select One

    The rate expression for the reaction A(g)+B(g)→C(g) is rate=k[A]2[B]1/2. What changes in the initial concentrations of A and B will cause the rate of reaction to increase by a factor of eight?

    A[A]=[A]:[B] = 2[B]
    B[A]=2[A]:[B] = 4[B]
    C[A]=[A]:[B] = 4[B]
    D[A]=4[A]:[B] = [B]
  • Question 3 - Select One

    For the reaction A_((s)) + 2B_((g)) ƒhArr 3C_((g)) . At constant pressure on addition of inert gas, the equilibrium state

    Aremains unaffected
    BShift towards right
    CShift towards left
    Dmay shift towards right or left