A pendulum consists of a small mass m at the end of a string of length L. The pendulum is pulled aside making an angle θ with the vertical and released. Taking the suspension point as the axis, at the instant of release
torque on the pendulum is mgL cosθ
torque on the pendulum is mgL sinθ
angular acceleration of pendulum is gcosθL
angular acceleration of pendulum is gsinθL
The correct Answer is:B, D
To solve the problem regarding the pendulum, we need to find the torque and angular acceleration at the moment of release when the pendulum is displaced to an angle θ from the vertical.
1. Understanding the Setup:
- We have a pendulum consisting of a mass m attached to a string of length L.
- The pendulum is pulled aside to an angle θ and then released.
2. Identifying Forces:
- The weight of the mass m acts downwards and can be resolved into two components:
- mgcosθ (perpendicular to the string)
- mgsinθ (parallel to the direction of motion)
3. Calculating Torque:
- The torque τ about the pivot point (suspension point) is given by the formula:
τ=r×F
- Here, r is the length of the string L and F is the force causing the torque, which is mgsinθ.
- Since the force is acting perpendicular to the line of action, the torque can be calculated as:
τ=L⋅(mgsinθ)=mgLsinθ
4. Finding Angular Acceleration:
- The relationship between torque and angular acceleration α is given by:
τ=Iα
- The moment of inertia I for a point mass at a distance L from the pivot is:
I=mL2
- Substituting the values of torque and moment of inertia into the equation gives:
mgLsinθ=mL2α
- Dividing both sides by mL2 (and canceling m and L):
α=gsinθL
Final Answers:
- The torque on the pendulum at the moment of release is:
τ=mgLsinθ
- The angular acceleration of the pendulum at that moment is:
α=gsinθL
- We have a pendulum consisting of a mass m attached to a string of length L.
- The pendulum is pulled aside to an angle θ and then released.
- The weight of the mass m acts downwards and can be resolved into two components:
- mgcosθ (perpendicular to the string)
- mgsinθ (parallel to the direction of motion)
- The torque τ about the pivot point (suspension point) is given by the formula:
τ=r×F
- Here, r is the length of the string L and F is the force causing the torque, which is mgsinθ.
- Since the force is acting perpendicular to the line of action, the torque can be calculated as:
τ=L⋅(mgsinθ)=mgLsinθ
- The relationship between torque and angular acceleration α is given by:
τ=Iα
- The moment of inertia I for a point mass at a distance L from the pivot is:
I=mL2
- Substituting the values of torque and moment of inertia into the equation gives:
mgLsinθ=mL2α
- Dividing both sides by mL2 (and canceling m and L):
α=gsinθL
- The torque on the pendulum at the moment of release is:
τ=mgLsinθ
- The angular acceleration of the pendulum at that moment is:
α=gsinθL
Topper's Solved these Questions
- KINEMATICSBook:FIITJEEChapter:KINEMATICSExercise:ASSERTION-REASON MCQ ( SINGLE CORRECT)
Explore 8 Videos
- KINEMATICSBook:FIITJEEChapter:KINEMATICSExercise:PARAGRAPH -V
Explore 2 Videos
- KINEMATICSBook:FIITJEEChapter:KINEMATICSExercise:SECTION(II) : MCQ ( SINGLE CORRECT)
Explore 47 Videos
- HEAT AND TEMPERATUREBook:FIITJEEChapter:HEAT AND TEMPERATUREExercise:NUMERICAL BASES QUESTIONS
Explore 1 Video
- LAWS OF MOTIONBook:FIITJEEChapter:LAWS OF MOTIONExercise:COMPREHENSION-III
Explore 2 Videos
Similar Questions
A simple pendulum consists of a ball of mass m connected to a string of length L. The ball is pulled aside so that the string makes an angle of 53∘ with the verticle and is released. Find the ratio of the minumum and the tension in the string.
View SolutionAS simple pendulum consists of a 50 cm long string connected to a 100 g ball. The ball is pulled aside so that the string makes an angel of 370 with the verticl and is then released. Find the tension in the string when the bob is at its lowest position.

View Solution
Knowledge Check
A conical pendulum of length L makes an angle θ with the vertical. The time period will be
 A2π√lcosθgB2π√LgcosθC2π√LtanθγD2π√Lgtanθ
A2π√lcosθgB2π√LgcosθC2π√LtanθγD2π√LgtanθA pendulum bob of mass m charge q is at rest with its string making an angle θ with the vertical in a uniform horizontal electric field E. The tension in the string in
AmgsinθBmgCqEsinθDqEcosθA simple pendulum consisting of a mass M attached to a string of length L is released from rest at an angle α. A pin is located at a distance l below the pivot point. When the pendulum swings down, the string hits the pin as shown in figure. The maximum angle θ which the string makes with the vertical after hitting the pin is
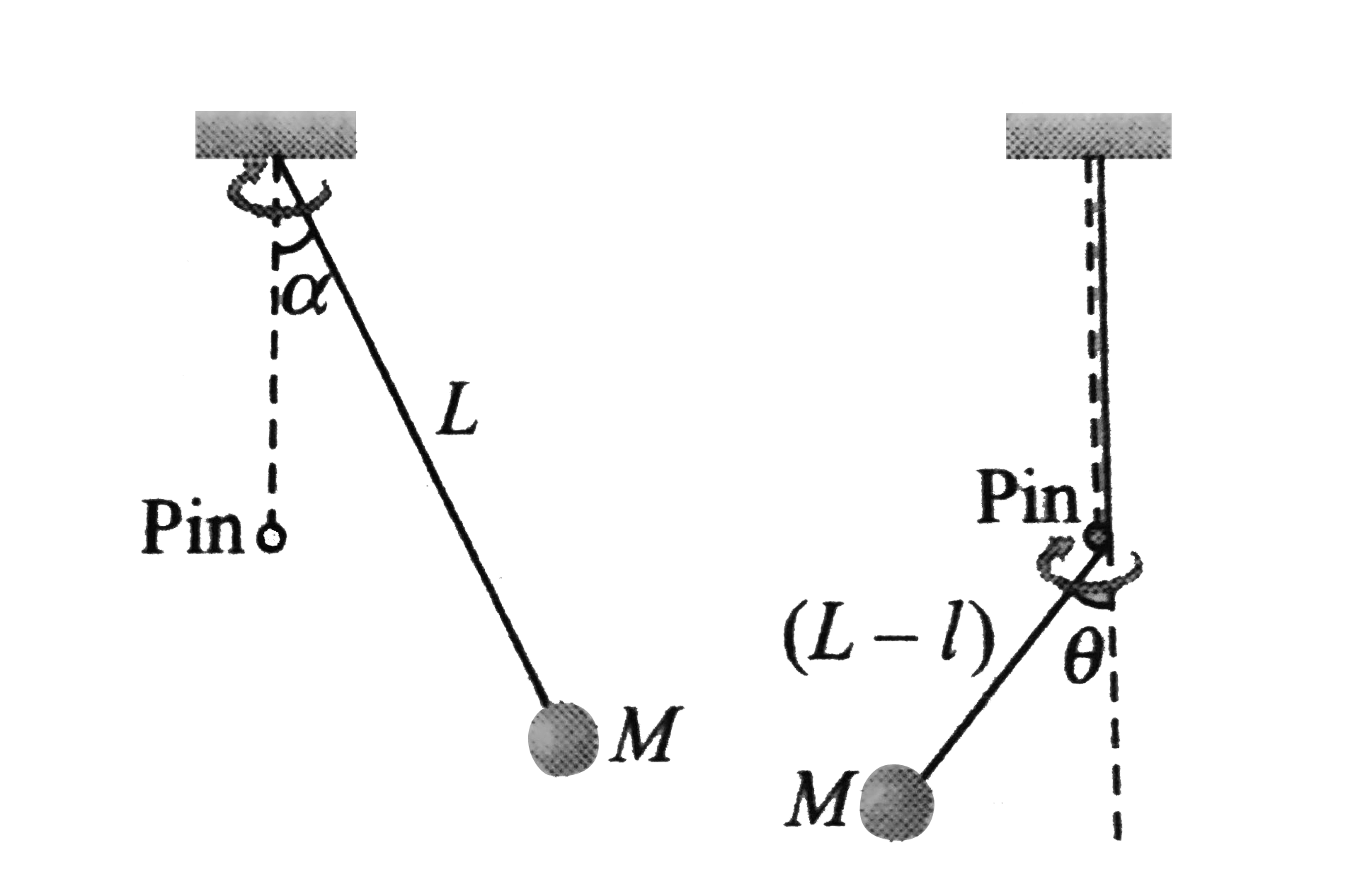 A(a) cos−1[Lcosα+lL+l]B(b) cos−1[Lcosα+lL−l]C(c) cos−1[Lcosα−1L−l]D(d) cos−1[Lcosα−1L+l]
A(a) cos−1[Lcosα+lL+l]B(b) cos−1[Lcosα+lL−l]C(c) cos−1[Lcosα−1L−l]D(d) cos−1[Lcosα−1L+l]
A simple pendulum of length l ils pulled aside to make an angle θ with the vertical. Find the magnitude of the torque of the weitht w of the bob about the point of suspension. When is the torque zero?
View SolutionThe figure shows a pendulum of length l suspended at a distance x vertically above a peg.
(a) The pendulum bob is deflected through an angle θ and then released. Find the speed of the bob at the instant
(b) The pendulum is released when θ=90∘. For what x (position of peg) will the pendulum complete the circle ?
(c) The pendulum is released when θ=60∘. what is the velocity of the bob as it passes position E.

View SolutionA pendulum bob of mass m and length L is released from angle θ with the vertical. Find
(a) the speed of the bob at the bottom of the swing and
(b) tension in the string at that time
View SolutionA heavy small sized sphere is suspended by a string of length 2. The sphere is rotated uniformly in a horizontal circle with the string making an angle θwith the vertical. The time period of this conical pendulum is
View SolutionA simple pendulum of length l and mass (bob) m is suspended vertically. The string makes an angle θ with the vertical. The restoring force acting on the pendulum is
View Solution
