A capacitor of capacitance C, which is initially charged up to a potential differnce ε, is connected with a battery of emf ε/2 such that the positive terminal of the battery is connected with the positive plate of the capacitor. After a long time.
i. find the total charge flow through the battery
ii. find the total work done by the battery
iii. Find the heat dissipated in the circuit during the process of charging.
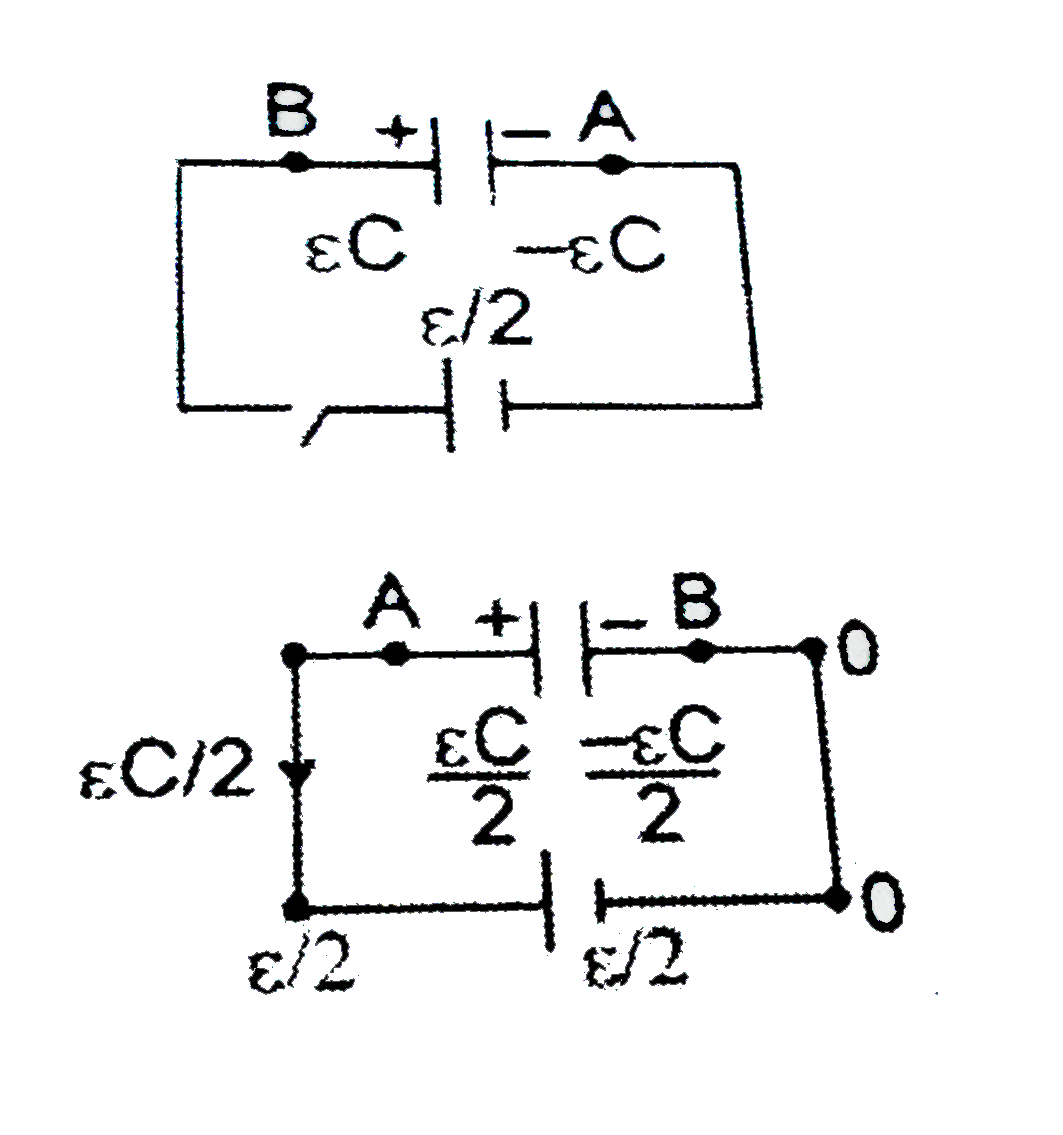
(i) Let potential of A is 0 so at B it is ε2. So final charge on capacitor =Cε/2
Charge flow through the capacitor =(Cε/2−Cε)=−Cε/2
So charge is entering into battery.
(ii) finally, Change in energy of capacitor =Ufinal−Uinitial
=12C(ε2)2−ε2C2=18ε2C−12ε2C=−3ε2C8
Work done by battery =ε2×(−εC2)=−ε2C4
(iii) Work done by battery = Change in energy of capacitor + Heat produced
Heat produced =3ε2C8−ε2C4=ε2C8
Topper's Solved these Questions
- CAPACITANCEBook:RESONANCEChapter:CAPACITANCEExercise:Miscellaneous Solved Example
Explore 3 Videos
- CAPACITANCEBook:RESONANCEChapter:CAPACITANCEExercise:Exercise - 1
Explore 79 Videos
- ATOMIC PHYSICSBook:RESONANCEChapter:ATOMIC PHYSICSExercise:Advanved level problems
Explore 17 Videos
- COMMUNICATION SYSTEMSBook:RESONANCEChapter:COMMUNICATION SYSTEMSExercise:Exercise 3
Explore 13 Videos
Similar Questions
A capacitor of capacitance C which is initially charged up to a potential differnce ε is connected with a battery of emf ε such that the positive terminal of the battery is connected with the positive plate of the capacitor. Find the heat loss in the circuit the process of charging.
View SolutionA capacitor of capacitance C, which is initially uncharged , is connected with a battery of emf ε. Find the heat dissipated in the circuit during the process of charging .
View Solution
Knowledge Check
A capacitor of 5μF is charged to a potential of 100V. Now, this charged capacitor is connected to a battery of 100V with the posiitive terminal of the battery connected to to the negative plate of the capacitor . For the given situation, mark the correct statement(s).
AThe charge flowing through the 100V battery is 500μC,BThe charge flowing through the 100V battery is 1000VCWork done by on the battery is 0.1JDWork done on the battery is 0.1J.A battery is of emf E is being charged from a charger such that positive terminal of the battery is connected to terminal A of charger and negative terminal of the battery is connected to terminal B of charger. The internal resistance of the battery is r.
APotential difference across points A and B must be more than E.BA must be at higher potential than BCIn battery, current flows from positivity terminal to the negative terminalDNo current flows through battery
20 coulomb charge flows through 15 volt battery in a certain interval. Find work done (in J) by the battery?
View SolutionA capacitor of capacitance C is charged to a potential difference V0 and then connected with a resistance and a battery of EMF 3V0 such that the positively charged plate of the capacitor is connected to the positive terminal of the battery. The total heat generated in the resistance until the current in the circuit becomes zero is n(CV20). the value of n is ________.
View SolutionA capacitor of capacitance as C is given a charge Q. At t=0,it is connected to an ideal battery of emf (ε)through a resistance R. Find the charge on the capacitor at time t.
View SolutionA capacitor of capacitance as C is given a charge Q. At t=0,it is connected to an ideal battery of emf (ε)through a resistance R. Find the charge on the capacitor at time t.
View SolutionIn a telegraph______is connected to positive terminal of a battery and the negative terminal of the battery is connected to__________
View SolutionThere is a capacitor with capacitance C, charged up to a potential difference of V. It is disconnected from the battery then both plates are connected to each other by a metal wire. How much heat will be dissipated?
View Solution