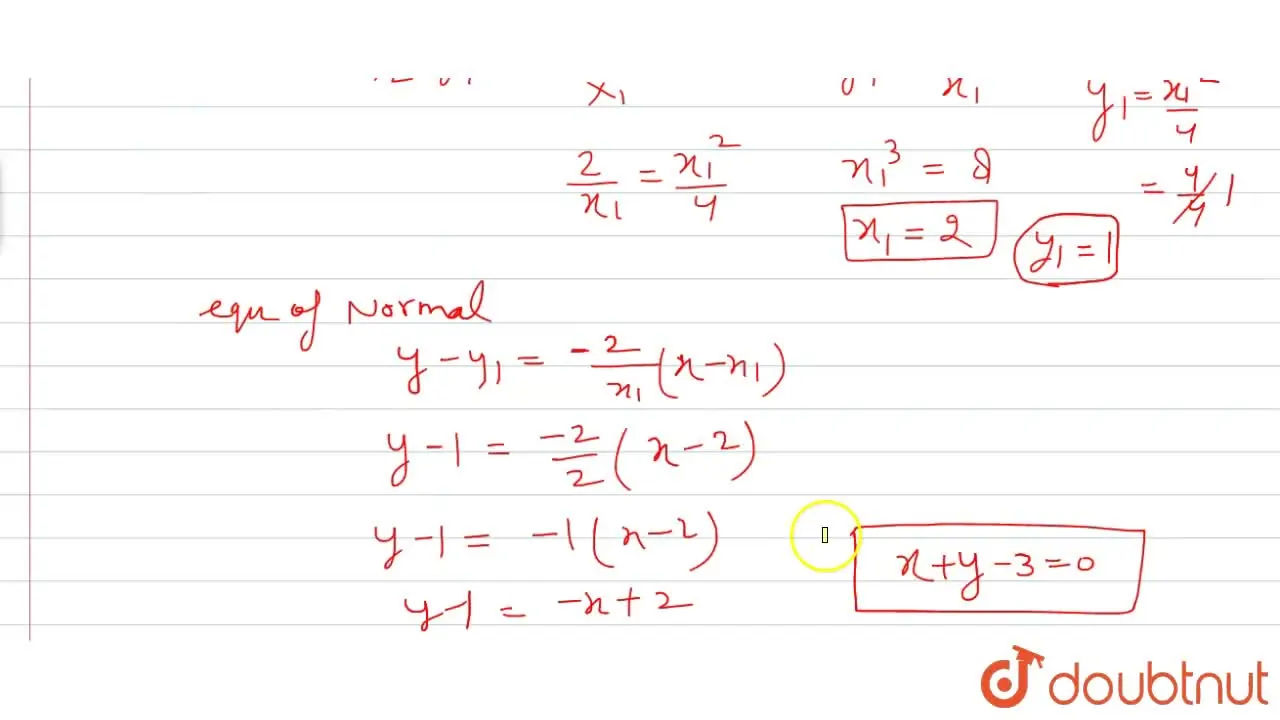
Find the equation of the normal to the curve x2=4ywhich passes through the point (1, 2).
The correct Answer is:(i) 2x+y=4,y=2x (ii) x+y=3
Step by step video, text & image solution for Find the equation of the normal to the curve x^2=4ywhich passes through the point (1, 2). by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Related Playlists
- BOOK - RESONANCECHAPTER - APPLICATION OF DERIVATIVESEXERCISE - Exersise -1B6videos
APPLICATION OF DERIVATIVES
- BOOK - RESONANCECHAPTER - APPLICATION OF DERIVATIVESEXERCISE - Exersise -1C4videos
APPLICATION OF DERIVATIVES
- BOOK - RESONANCECHAPTER - APPLICATION OF DERIVATIVESEXERCISE - Self Practice Problems34videos
APPLICATION OF DERIVATIVES
- BOOK - RESONANCECHAPTER - COMBINATORICSEXERCISE - Exercise-2 (Part-II: Previously Asked Question of RMO)8videos
COMBINATORICS
Similar Questions
Find the equation of the normal to curve x2=4ywhich passes through the point (1, 2).
View SolutionFind the equation of the normal at a point on the curve x2=4y, which passes through the point (1,2). Also find the equation of the corresponding tangent.
View SolutionFind the equation of the tangents to the curve 3x2−y2=8, which passes through the point (43,0).
View SolutionThe number of normals to the curve 3y3=4x which passes through the point (0,1) is
View SolutionFind the equation of the line parallel to the line 3x−4y+2=0 and passing through the point (−2,5).
View SolutionFind the equation of the normal to the curve ay2=x3, at the point (am2,am3). If normal passes through the point (a,0) then find the slope.
View SolutionFind the equation of the normal to curve y2=4x at the point (1,2).
View SolutionFind the equation of tangent and normal to the curve y=3x2−x+1 at the point (1,3) on it.
View SolutionFind the equation of the curve for which the length of the normal is constant and the curves passes through the point (1,0).
View Solutionfind the equation of the curve which passes through the point (2,2) and satisfies the differential equation y−xdydx=y2+dydx
View Solution