Find the greatest and least values of f(x) =x2−12x X∈[−1,3].
The possible points of maxima/minima are critical points points and the boundary points. For X∈[−1,3] and f(x)=x3−12x
X=2 is the only critical point.
Examining the value of f(x) at points x =- 1,2,3 We can find greatest and least values.
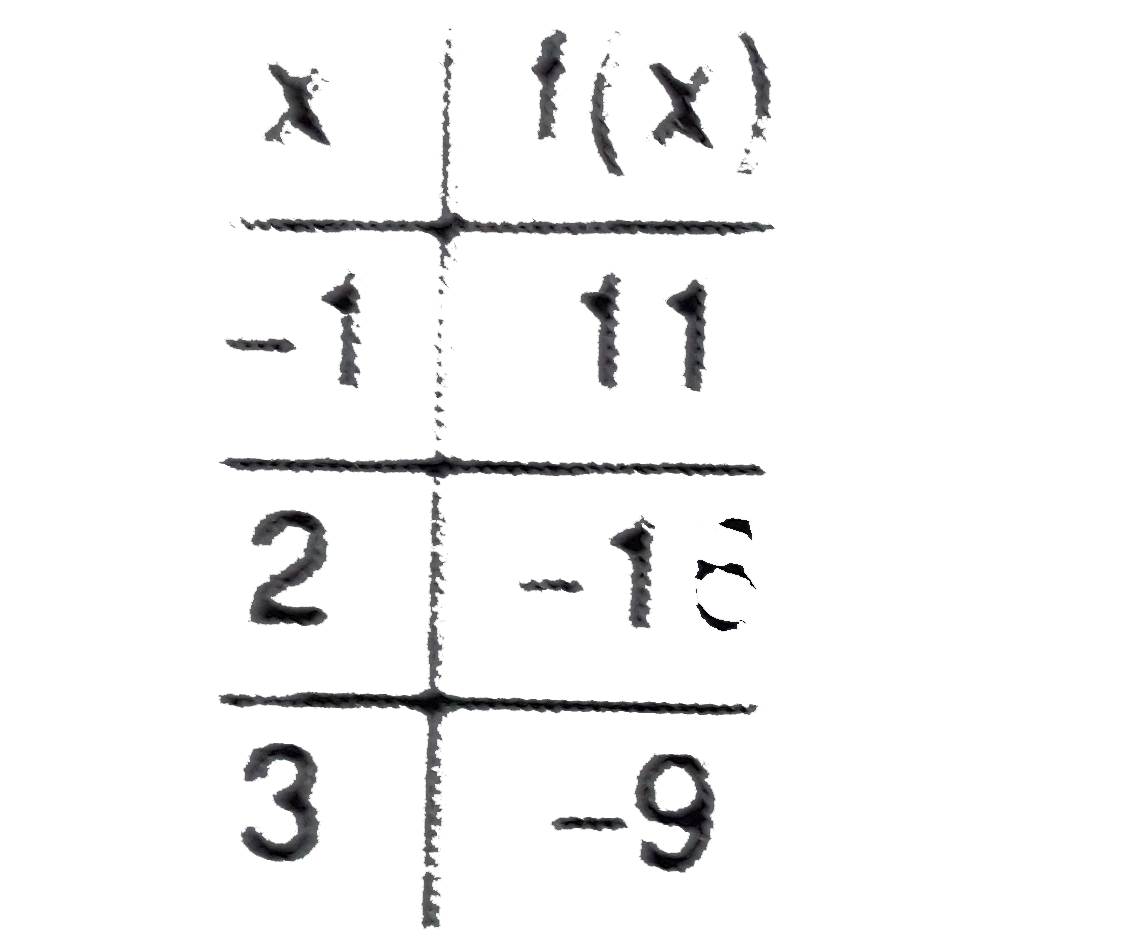
∴ Minimum f(x) =- 16 & Maximum f(x) =11.
Topper's Solved these Questions
- APPLICATION OF DERIVATIVESBook:RESONANCEChapter:APPLICATION OF DERIVATIVESExercise:Self Practice Problems
Explore 34 Videos
- APPLICATION OF DERIVATIVESBook:RESONANCEChapter:APPLICATION OF DERIVATIVESExercise:Exersise -1A
Explore 5 Videos
- COMBINATORICSBook:RESONANCEChapter:COMBINATORICSExercise:Exercise-2 (Part-II: Previously Asked Question of RMO)
Explore 8 Videos
Similar Questions
Find the greatest and least values of f(x)=x2logx in [1,e]
View SolutionFind the greatest and least value of x+22x2+3x+6
View SolutionLet f(x)=x+√x . Find the greatest and least value of f(x) for x∈(0,4) .
View SolutionFind greatest and least values of f(x)=(a+x)(b+x)(c+x), x≻c
View SolutionFind the greatest and least value of (sin−1x)3+(cos−1x)3.
View SolutionFind greatest and least values of f(x)=x−sin2x+13sin3x, x ∈[0, π]
View SolutionFind the greatest and least values of function f(x)=3x4−8x3−18x2+1.
View SolutionFind the greatest and least value of y=x3−3x2+6x−2 on [−1,1] y is differentiable function of x and y'(x)=3x2−6x+6=3(x2−2x+2)=3(x−1)2>0.
View SolutionFind least and greatest value of f(x)=ex^(2−4x+3) in [− 5,5]
View SolutionThe difference between the greatest and least values of f(x)=∫x0(t+1)dt on [2,3] is
View Solution