Find critical points of f(x) =max (sinx cosx) ∀,X∈(0,2π).
Video Solution
Text Solution
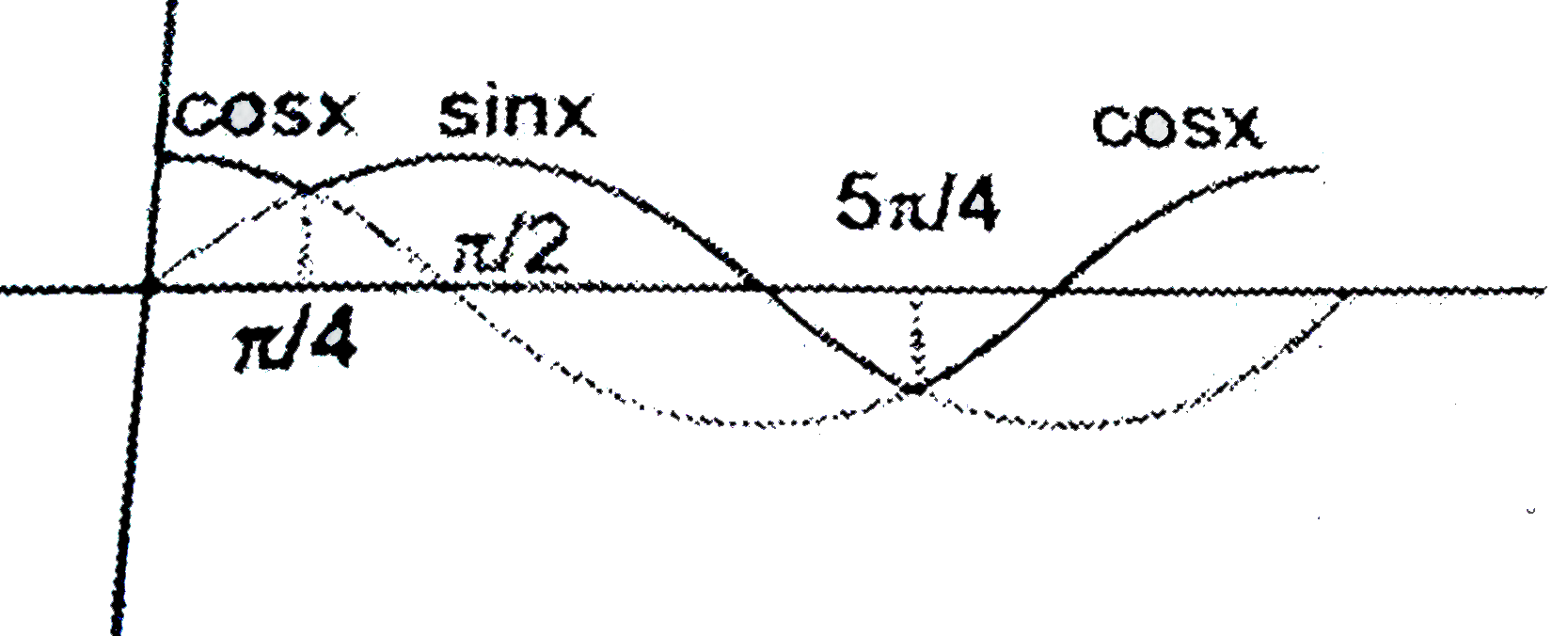
From the figure it is clear that f(x) has three critical points X=π4,π2,5π4,
|
Topper's Solved these Questions
- APPLICATION OF DERIVATIVESBOOK - RESONANCECHAPTER - APPLICATION OF DERIVATIVESEXERCISE - Self Practice Problems
34 Videos
- APPLICATION OF DERIVATIVESBOOK - RESONANCECHAPTER - APPLICATION OF DERIVATIVESEXERCISE - Exersise -1A
5 Videos
- COMBINATORICSBOOK - RESONANCECHAPTER - COMBINATORICSEXERCISE - Exercise-2 (Part-II: Previously Asked Question of RMO)
8 Videos
Similar Questions
Knowledge Check
The number of critical points of f(x)=max{sinx,cosx},∀x∈(−2π,2π), is
A5B6C7D8The critical points of the function f(x)=2sinx,x∈[0,2π] are :
A0,π2,3π2B0,π,2πCπ2,3π2Dπ,2π
Sketch the graph of y=max(sinx,cosx),∀x∈(−π,3π2).
View SolutionThe number of points where f(x) = max {|sin x| , | cos x|} , x∈(−2π,2π) is not differentiable is ______
View SolutionFind all the points of local maxima and minima of the function f given by f(x)=sinx−cosx,x∈[0,2π]
View SolutionFind the values of x, if any, for which f(x) has local maximum and local minimum when
f(x)=sinx+cosx,x∈[0,2π]
View SolutionFind the range of
f(x)=|sinx|+|cosx|0≤x≤π
View SolutionLet f(x) = sin x (1+cos x) ,x∈(0,2π). Find the number of critical points of f(x) . Also identify which of these critical points are points of Maxima/Minima.
View Solution
