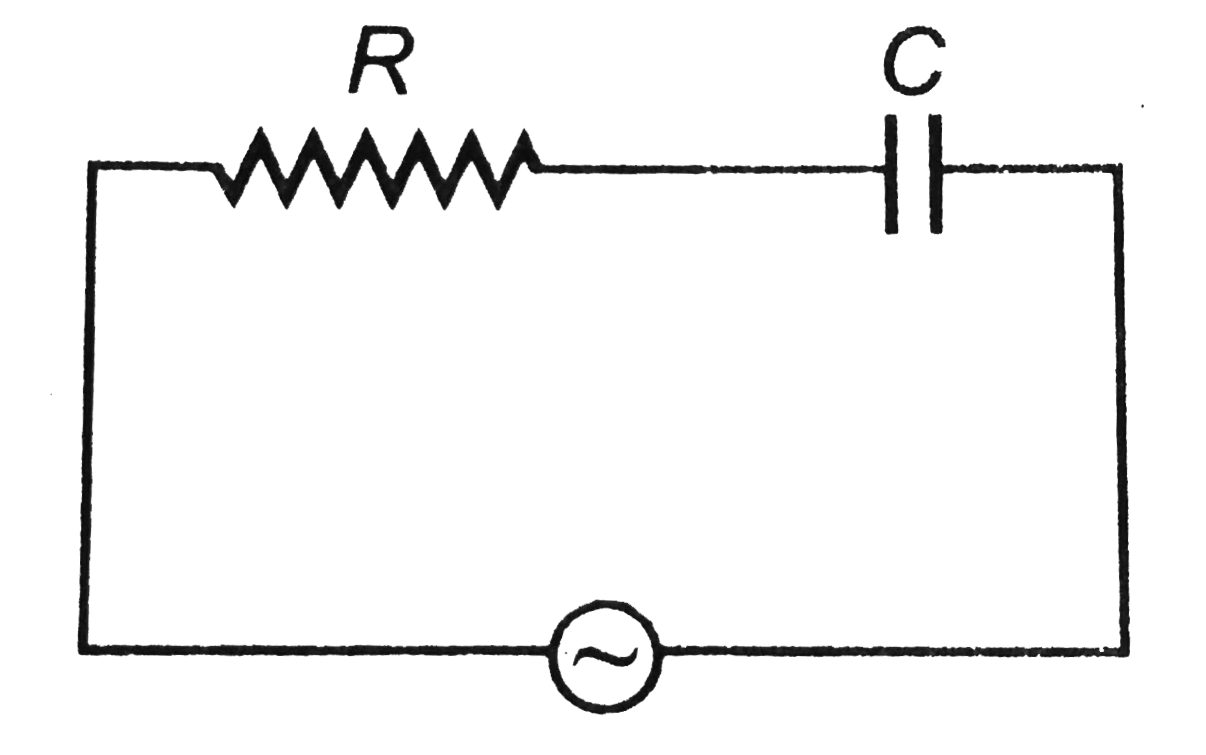
In an RC series circuit(in fig), the rms voltage of source is 200V and its frequency is 50 Hz, if $R = 100 Ω and C = \frac{100}{p o} (μ) F$ , find
(a) inpedance of the circuit.
(b) power factor angle,
(c ) power factor,
(d) current,
(e) maximum current
(f) voltage across R,
(g) voltage across C,
(h) maximum voltage across R,
(i) maximum voltage across C,
(j) ltPgt,
(k) $> P_{C} <$ .
$(m) i (t)$ , $V_{R} (t), and V_{C} (t)$ .

Video Solution

Text Solution

Verified by Experts

$X_{C} = \frac{10^{6}}{\frac{100}{π} (2 π 50)} = 100 Ω$
(i) $Z = \sqrt{R^{2} + X c^{2}} = \sqrt{100^{2} + {(100)}^{2}} = 100 \sqrt{2} Ω$
(ii) $tan ϕ = \frac{X c}{R} = 1 ∴ ϕ = 45^{\circ}$
(iii)Power factor = $cos θ = \frac{1}{\sqrt{2}}$ ltbtgt (iv)Current $I_{r m a} = \frac{V_{r m a}}{Z} = \frac{200}{100 \sqrt{2}} = \sqrt{2} A$
(v)Maximum current = $I_{r m a \sqrt{2} = 2 A}$
(vi)voltage across $R = V_{R m s} = I_{r m s} R = \sqrt{2} \times 100$ volt
(vii)voltage across $C = V_{C m s} = I_{r m s} X_{C} = \sqrt{2} \times 100$ volt
(viii)max voltage across $R = \sqrt{2} V_{R m s} = 200$ volt
(ix)max voltage across $C = \sqrt{2} V_{C . r m s} = 200$ volt
(x) $< P > = V_{r m s} I_{r m s} cos ϕ = 200 \times \sqrt{2} \times \frac{1}{\sqrt{2}} = 200$ volt
(xi) $< P_{R} \geq I_{r m s}^{2} R = 200 W$
(xii) $< P_{C} \geq 0$

Updated on:21/07/2023

In series LCR circuit, the phase difference between voltage across L and voltage across C is
AZero
B $π$
C $\frac{π}{2}$
D $2 π$
A $50 H z A C$ source of $20 V$ is connected across $R$ and $C$ as shown in figureure.

The voltage across $R$ is $12 V$ . The voltage across $C$ is
A $8 V$
B $16 V$
C $10 V$
Dnot possible to determine unless value of $R$ and $C$ are given
In an L-C-R circuit, if V is the effective value of the applied voltage $V_{R}$ is the voltage across R, $V_{L}$ is the effective voltage across L, $V_{C}$ is the effective voltage across C, then
A $V = V_{R} + V_{L} + V_{C}$
B $V^{2} = V_{R}^{2} + V_{L}^{2} + V_{C}^{2}$
C $V^{2} = V_{R}^{2} + {(V_{L} - V_{C})}_{L}^{2}$
D $V^{2} = V_{L}^{2} + {(V_{R} - V_{C})}_{R}^{2}$