A cuboidal piece of wood has dimensions a, b and c. its relatively density is d. it is floating in a large body of water such that side a is vertical. It is pushed down a bit and released. The time period of SHM executed by it is
2π√abcg
2π√gda
2π√bcdg
2π√dag
The correct Answer is:D
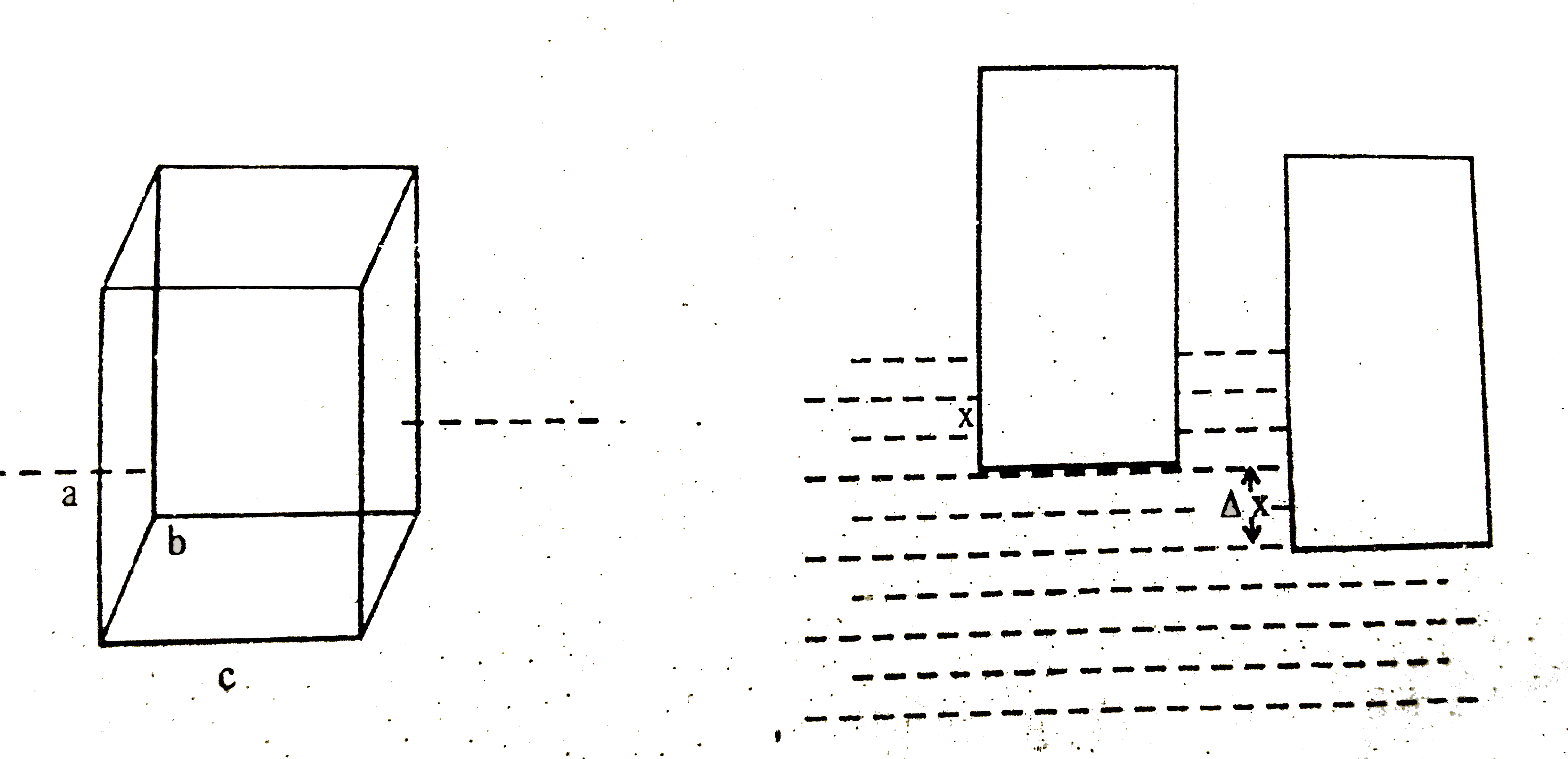
W=B
abcdg=xbc×1×g ⇒x=ad
FR=−Δxbcg ⇒FR=−bcgΔξmplies K=bcg
T=2π√mK ⇒T=2π√abcdbcg⇒ T=2π√adg
Topper's Solved these Questions
Similar Questions
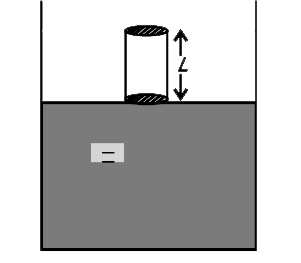
A glass tube is floating in a liquid with 30 cm of its length immersed in the liquid. If the glass tube is pushed down and released, then calculate the time period of vibration of glass tube.
View Solution(i) A cylindrical container has area of cross section equal to 4A and it contains a non viscous liquid of density 2ρ. A wooden cylinder of cross sectional area A and length L has density ρ. It is held vertically with its lower surface touching the liquid. It is released from this position. Assume that the depth of the container is sufficient and the cylinder does not touch the bottom.
(a) Find amplitude of oscillation of the wooden cylinder.
(b) Find time period of its oscillation.
Two cubical blocks of side length a and 2a are stuck symmetrically as shown in the figure. The combined block is floating in water with the bigger block just submerged completely. The block is pushed down a little and released. Find the time period of its oscillation. Neglect viscostiy.
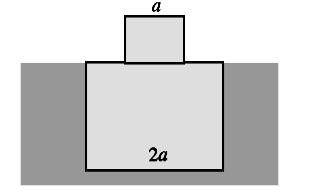
View Solution
Knowledge Check
A piece of wood has dimensions 'a', 'b' and 'c'. Its relative density is d. It is floating in water such that the side 'a' is vertical. It is now pushed down gently and relesed. The time-period of S.H.M. is
AT=2π√abcgBT=2π√bcdgCT=2π√gdaDT=2π√dagA pole is floating in a liquid with 80 cm of its length immersed. It is pushed doun a certain distance and then released. Time period of vertical oscillation is
A4π7sB3π7sC2π7sDπ7sA cylinderical block of wood of mass m and area cross-section A is floating in water (density = ρ) when its axis vertical. When dressed a little and the released the block starts oscillating. The period oscillations is
A(2π√mρbgB2π√mgρAC2π√ρAgmD2π√ρAmg)
A thin vertical tube of weight 12 g and external diameter 1 cm is made to float vertically in water by placing 12 g of mercury at its bottom. If the tube is depressed slightly and then released, determine the time period of S.H.M.
Take g=10m/s2
View SolutionA block of wood weight 4kg and has a relative density of 0.6 it is to be loaded with lead so that it will float in eater with 0.9 of its volume immersed. What weight of lead will be needed (a) if the is on top of the wood, (b) if the lead is attached below the wood ? (density of lead =11,340kgm−3)
View SolutionA cube made of wood having specific gravity 0.4 and side length a is floated in a large tanck full of water.
If the cube is depressed slighly, it executes SHM from it's position. What is it's time period?
View SolutionA cube made of wood having specific gravity 0.4 and side length a is floated in a large tank full of water.
 If the cube is depressed slightly, it excutes SHM from It's position. What is it's time period?
If the cube is depressed slightly, it excutes SHM from It's position. What is it's time period?
View SolutionA brass cube of side a and density σis floating in mercury of density ρ. If the cube is displaced a bit vertically, it executes S.H.M. Its time period will be
View Solution
