Solve for x: (i) 10x2−27x+5=0 (ii) pqx2−(p2+q2)x+pq=0
The correct Answer is:(i) 52,15 (ii) Pq,qP
To solve the given equations step by step, we will tackle each equation one at a time.
(i) Solve for x: 10x2−27x+5=0
Step 1: Rewrite the equation
We start with the equation:
10x2−27x+5=0
Step 2: Factor the quadratic
We need to factor the quadratic expression. To do this, we can look for two numbers that multiply to 10×5=50 and add up to −27. We can rewrite the middle term:
10x2−25x−2x+5=0
Step 3: Group the terms
Now, we group the terms:
(10x2−25x)+(−2x+5)=0
Step 4: Factor by grouping
Factoring out common terms from each group:
5x(2x−5)−1(2x−5)=0
Now, we can factor out (2x−5):
(2x−5)(5x−1)=0
Step 5: Set each factor to zero
Now we set each factor to zero:
1. 2x−5=0
2. 5x−1=0
Step 6: Solve for x
From 2x−5=0:
2x=5⟹x=52
From 5x−1=0:
5x=1⟹x=15
Final Solutions:
The solutions for the equation 10x2−27x+5=0 are:
x=52andx=15
---
(ii) Solve for x: pqx2−(p2+q2)x+pq=0
Step 1: Rewrite the equation
We start with the equation:
pqx2−(p2+q2)x+pq=0
Step 2: Divide by pq
To simplify, we can divide the entire equation by pq (assuming pq≠0):
x2−(p2+q2pq)x+1=0
Step 3: Identify coefficients
In the standard form ax2+bx+c=0, we have:
- a=1
- b=−(p2+q2pq)
- c=1
Step 4: Use the quadratic formula
We can use the quadratic formula x=−b±√b2−4ac2a:
x=(p2+q2pq)±√(p2+q2pq)2−4⋅1⋅12⋅1
Step 5: Simplify the expression
Calculating the discriminant:
(p2+q2pq)2−4=(p2+q2)2p2q2−4
This can be rewritten as:
(p2+q2)2−4p2q2p2q2
Step 6: Find the roots
Now we can express the roots:
x=p2+q2pq±√(p2+q2)2−4p2q2p2q22
This simplifies to:
x=p2+q2±√(p2−q2)22pq
Thus, the roots are:
x=p2+q2+(p2−q2)2pq=2p22pq=pqandx=p2+q2−(p2−q2)2pq=2q22pq=qp
Final Solutions:
The solutions for the equation pqx2−(p2+q2)x+pq=0 are:
x=pqandx=qp
---
We start with the equation:
10x2−27x+5=0
We need to factor the quadratic expression. To do this, we can look for two numbers that multiply to 10×5=50 and add up to −27. We can rewrite the middle term:
10x2−25x−2x+5=0
Now, we group the terms:
(10x2−25x)+(−2x+5)=0
Factoring out common terms from each group:
5x(2x−5)−1(2x−5)=0
Now, we can factor out (2x−5):
(2x−5)(5x−1)=0
Now we set each factor to zero:
1. 2x−5=0
2. 5x−1=0
From 2x−5=0:
2x=5⟹x=52
From 5x−1=0:
5x=1⟹x=15
The solutions for the equation 10x2−27x+5=0 are:
x=52andx=15
We start with the equation:
pqx2−(p2+q2)x+pq=0
To simplify, we can divide the entire equation by pq (assuming pq≠0):
x2−(p2+q2pq)x+1=0
In the standard form ax2+bx+c=0, we have:
- a=1
- b=−(p2+q2pq)
- c=1
We can use the quadratic formula x=−b±√b2−4ac2a:
x=(p2+q2pq)±√(p2+q2pq)2−4⋅1⋅12⋅1
Calculating the discriminant:
(p2+q2pq)2−4=(p2+q2)2p2q2−4
This can be rewritten as:
(p2+q2)2−4p2q2p2q2
Now we can express the roots:
x=p2+q2pq±√(p2+q2)2−4p2q2p2q22
This simplifies to:
x=p2+q2±√(p2−q2)22pq
Thus, the roots are:
x=p2+q2+(p2−q2)2pq=2p22pq=pqandx=p2+q2−(p2−q2)2pq=2q22pq=qp
The solutions for the equation pqx2−(p2+q2)x+pq=0 are:
x=pqandx=qp
Topper's Solved these Questions
- BASIC MATHSBOOK - ALLENCHAPTER - BASIC MATHSEXERCISE - EXERCISE-1
128 Videos
- BASIC MATHSBOOK - ALLENCHAPTER - BASIC MATHSEXERCISE - EXERCISE-2
80 Videos
- AIIMS 2019BOOK - ALLENCHAPTER - AIIMS 2019EXERCISE - PHYSICS
40 Videos
- CIRCULAR MOTIONBOOK - ALLENCHAPTER - CIRCULAR MOTIONEXERCISE - EXERCISE (J-A)
6 Videos
Similar Questions
Knowledge Check
(i) x2+6x+5=0
(ii) y2−10y+21=0Ax = y or no relation can be established between x and y.Bx≤yCx≥yDx<y(i)x2−10x+21=0
(ii) y2−11y+18=0Ax<yBx =y or no relation can be established between x and y.Cx≥yDx>y
Solve: (i) 27x2−10x+1=0.
(ii) 21x2−28x+10=0.
View SolutionSolve: (i) 3x2−4x+203=0.
(ii) x2−2x+23=0.
View SolutionSolve using formula :
(i) x2−4x−5=0 (ii) x2−7x−3=0
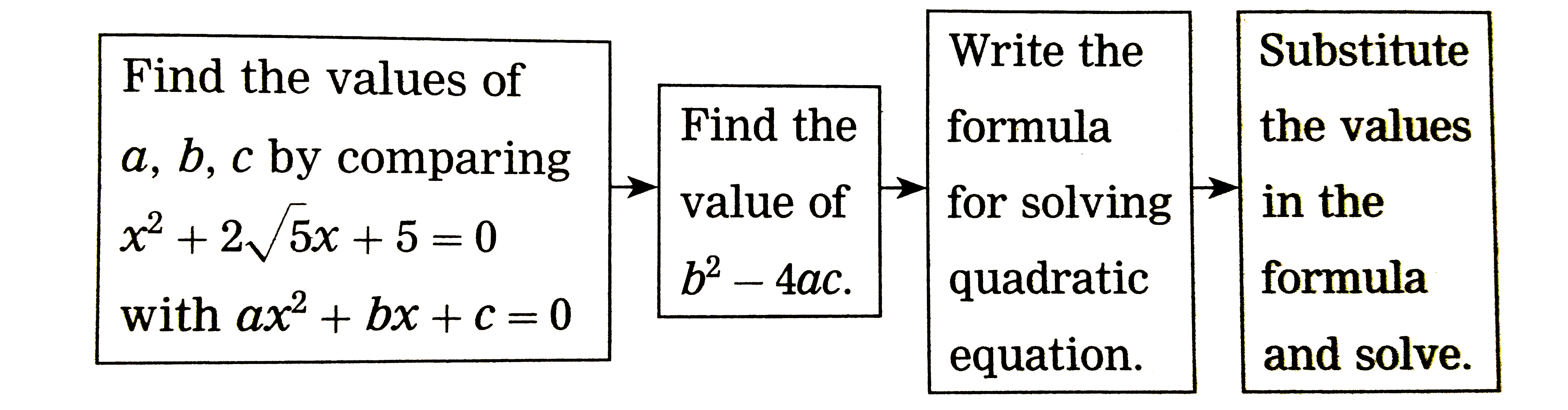
(iii) With the help of flow chart given below solve the equation x2+2√5x+5=0 using the formula :
View Solutiona2p2x2−q2=0,x=
View SolutionSolve log10(x2−2x−2)≤0.
View SolutionIf α,β are the roots of the equation px2−qx+r=0, then the equation whose roots are α2+rpandβ2+rp is (i) p3x2+pq2x+r=0 (ii) px2−qx+r=0 (iii) p3x2−pq2x+q2r=0 (iv) px2+qx−r=0
View Solution
