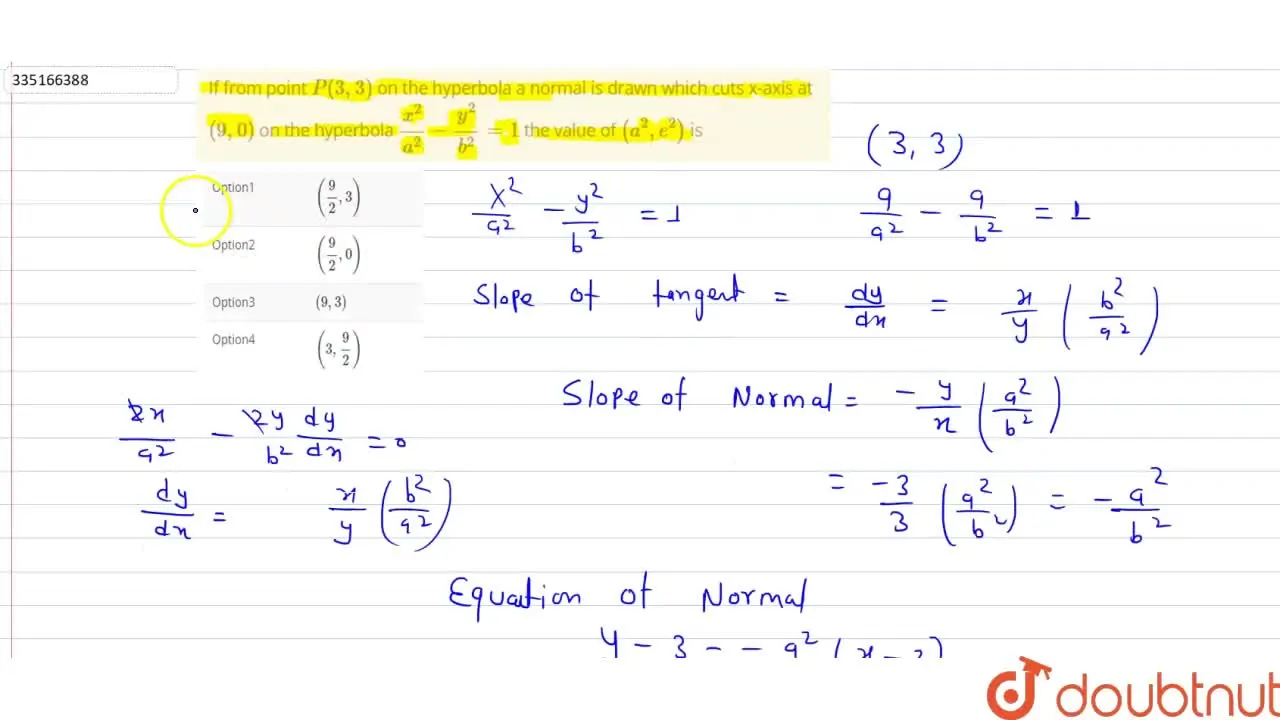
If from point $P (3, 3)$ on the hyperbola a normal is drawn which cuts x-axis at $(9, 0)$ on the hyperbola $\frac{x^{2}}{a^{2}} - \frac{y^{2}}{b^{2}} = 1$ the value of $(a^{2}, e^{2})$ is

A

$(\frac{9}{2}, 3)$

B

$(\frac{3}{2}, 2)$

C

$(\frac{9}{2}, 2)$

D

(9,3)

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A

|

Answer

Step by step video, text & image solution for If from point P(3,3) on the hyperbola a normal is drawn which cuts x-axis at (9,0) on the hyperbola x^2/a^2-y^2/b^2=1 the value of (a^2,e^2) is by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.

Updated on:21/07/2023

Class 12 MATHS QUESTION BANK

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
The distance of the origin from the normal drawn at the point $(1, - 1)$ on the hyperbola $4 x^{2} - 3 y^{2} = 1$ is
A $\frac{3}{5}$
B $\frac{4}{5}$
C $\frac{1}{5}$
D $\frac{7}{5}$
Question 2 - Select One
If the normal at an end of latus rectum of the hyperbola $\frac{x^{2}}{a^{2}} - \frac{y^{2}}{b^{2}} = 1$ passes through the point $(0, 2 b)$ then
Aeccentricity of the hyperbola is $\frac{1 + \sqrt{2}}{2}$
Beccentricity of the hyperbola is $\sqrt{2}$
Csquare of the eccentricity is 2 times the ratio of transverse and conjugate axis
Dratio of transverse and conjugate axis is unity
Question 3 - Select One
From any point on the hyperbola $H_{1} : (x^{2} / a^{2}) - (y^{2} / b^{2}) = 1$ tangents are drawn to the hyperbola $H_{2} : (x^{2} / a^{2}) - (y^{2} / b^{2}) = 2$ .The area cut-off by the chord of contact on the asymp- totes of $H_{2}$ is equal to
A $a b / 2$
B $a b$
C $2 a b$
D $4 a b$

JEE MAINS PREVIOUS YEAR-JEE MAINS 2020-MATHEMATICS

Home
Ask Doubt
Profile