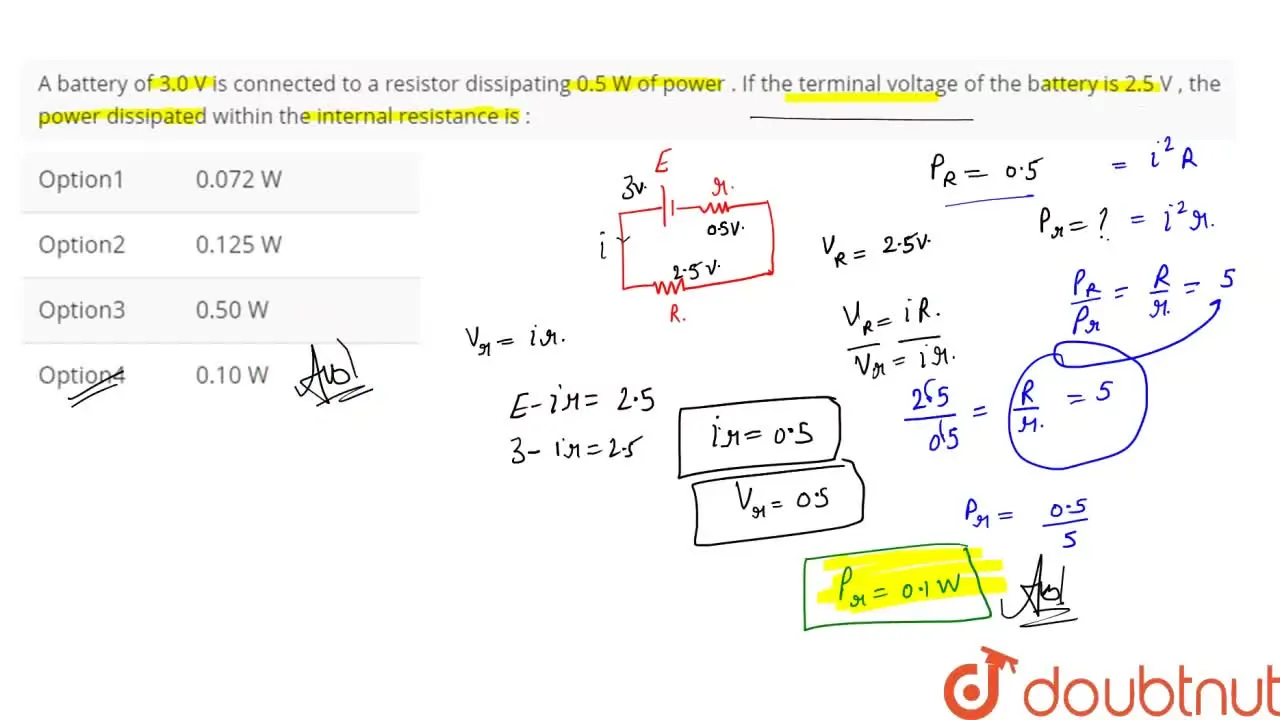
A battery of 3.0 V is connected to a resistor dissipating 0.5 W of power . If the terminal voltage of the battery is 2.5 V , the power dissipated within the internal resistance is :
0.072 W
0.125 W
0.50 W
0.10 W
Step by step video & image solution for A battery of 3.0 V is connected to a resistor dissipating 0.5 W of power . If the terminal voltage of the battery is 2.5 V , the power dissipated within the internal resistance is : by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Related Playlists
Similar Questions
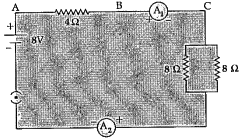
Power dissipated in 4Ω resistor is :View SolutionThe power dissipated 6Ω resistor is
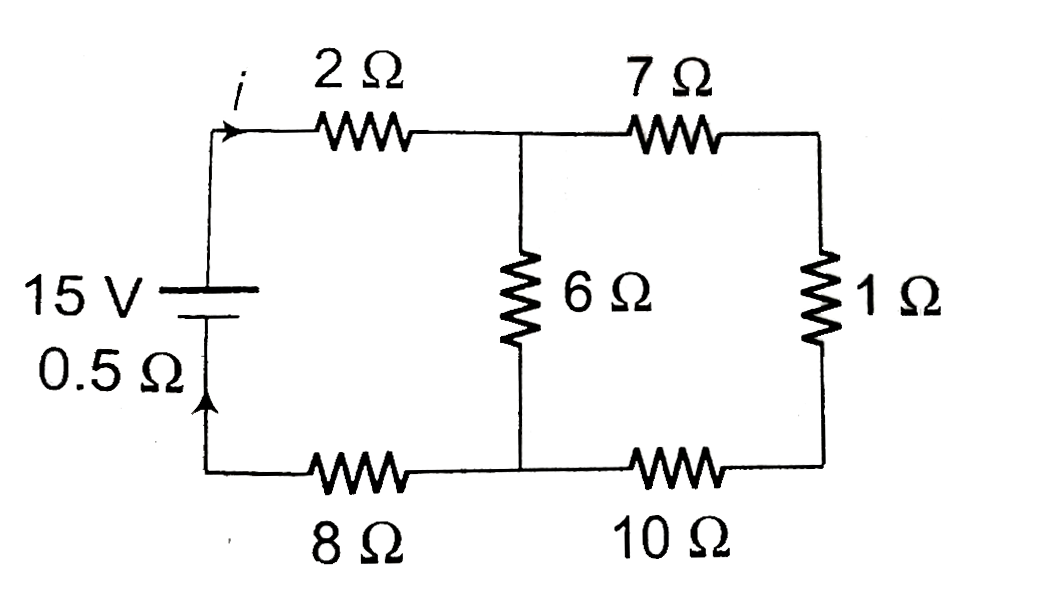 View Solution
View SolutionA 50V battery is connected across a 10 ohm resistor. The current is 4.5 amperes. The internal resistance of the battery is
View SolutionFive equal resistors when connected in series dissipated 5 W power. If they are connected in paralle, the power dissipated will be
View SolutionOn giving 220 V to a resistor the power dissipated is 40 W then value of resistance is
View SolutionA battery of emf 10 V and internal resistance 3Ωis connected to a resistor. The current in the circuit is 0.5 A . The terminal voltage of the battery when the circuit is closed is
View SolutionIf the power dissipated in 5Ω is 20W then power dissipated in 4Ω is:-
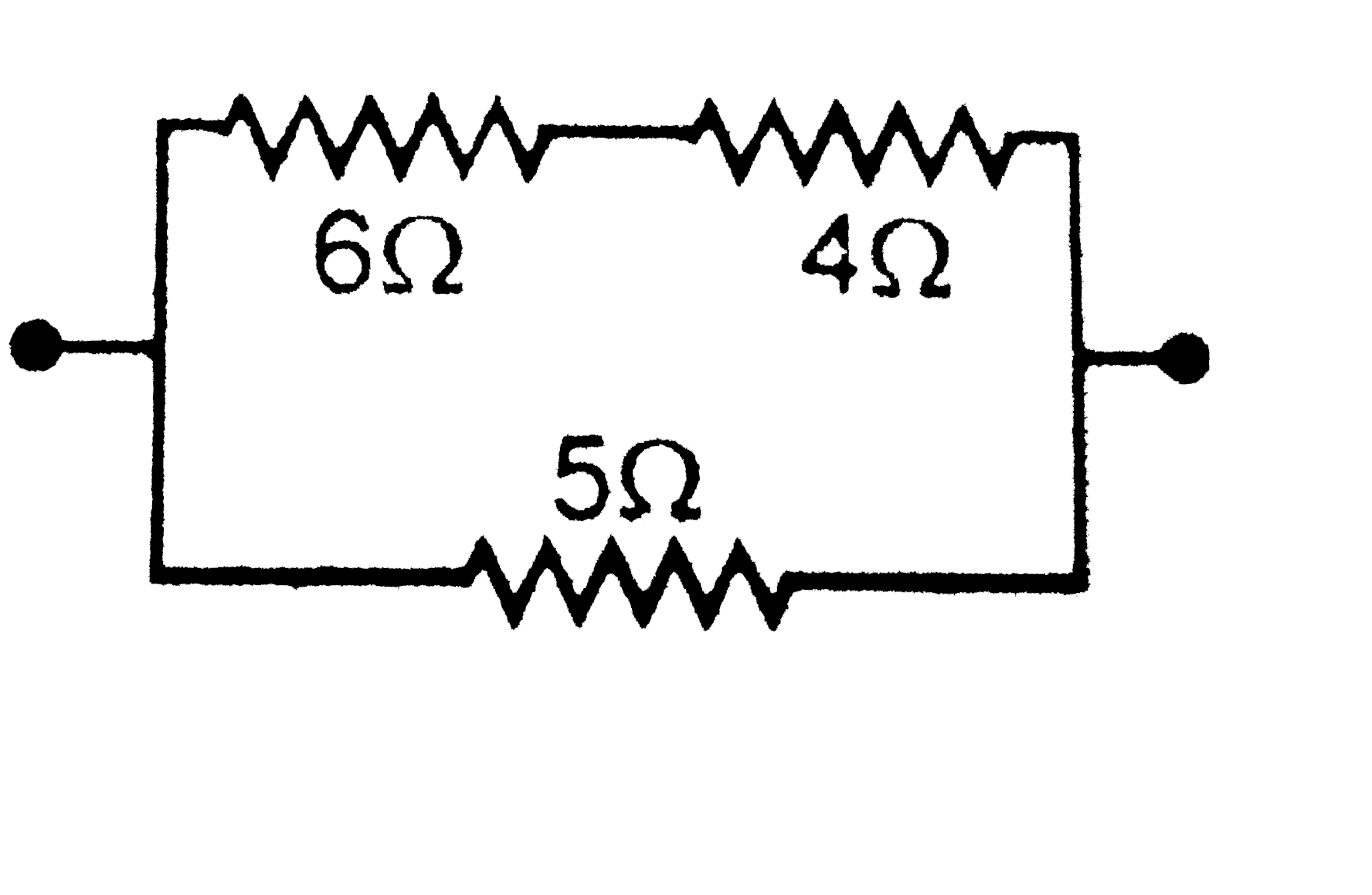 View Solution
View SolutionA battery when connected by resistance of 16Ω gives a terminal voltage of 12 V. and when connected by a resistance of 10Ω gives a terminal voltage of 11V. Then the emf of the battery ad its internal resistance
View SolutionA battery of e mf 10 V and internal resistance 3Ω is connected to a resistor as shown in the figure. If the current in the circuit is 0.5 A . then the resistance of the resistor will be
 View Solution
View SolutionFind the power dissipated in 3Ω resistance of the network of resistors.
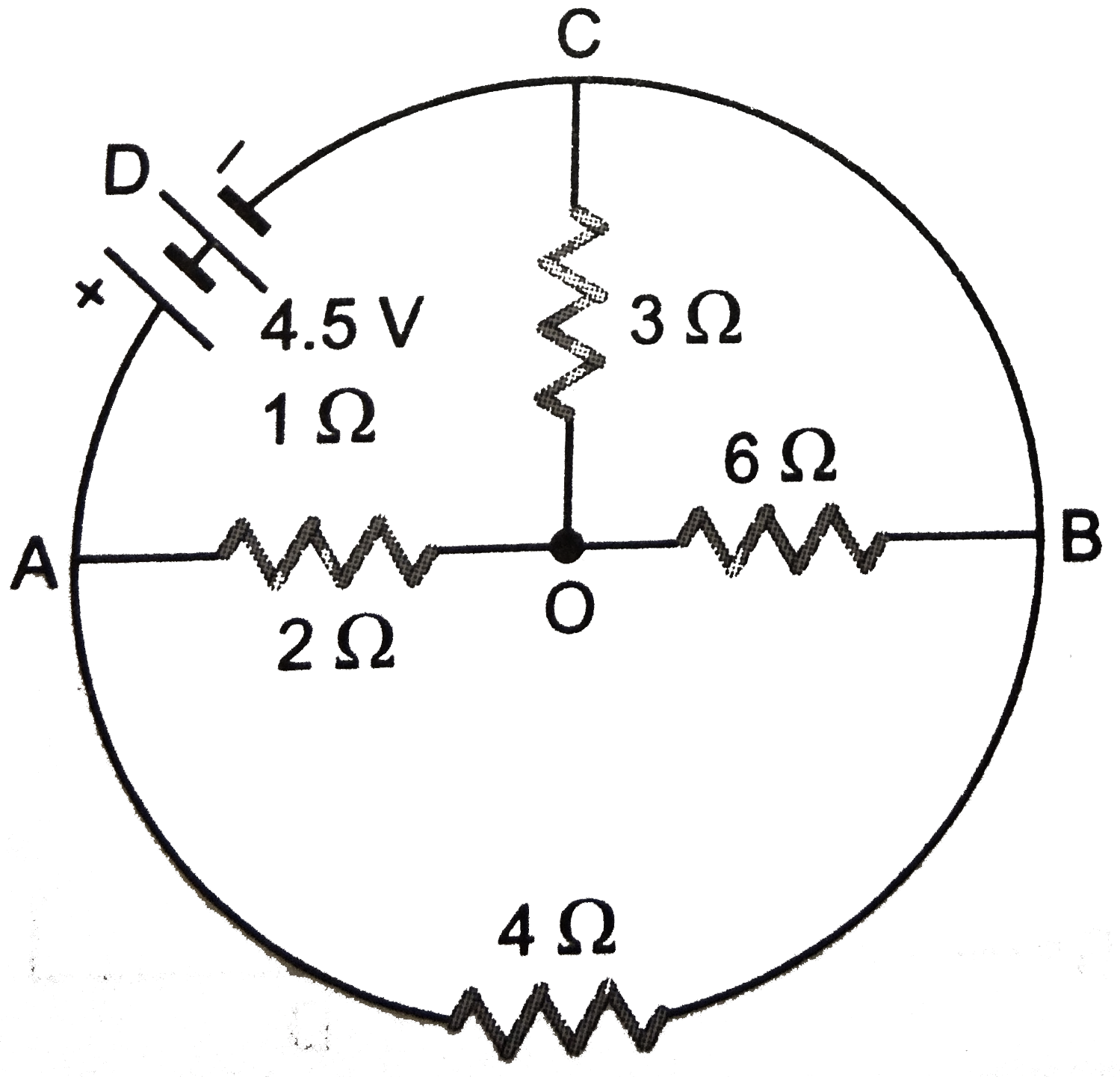 View Solution
View Solution