The ratio in which the plane →r.(→i−2→j+3→k)=17 divides the line joining the points −2→i+4→j+7→kand→3i−5→j+8→k is
1:5
1:10
3:5
3:10
The correct Answer is:d
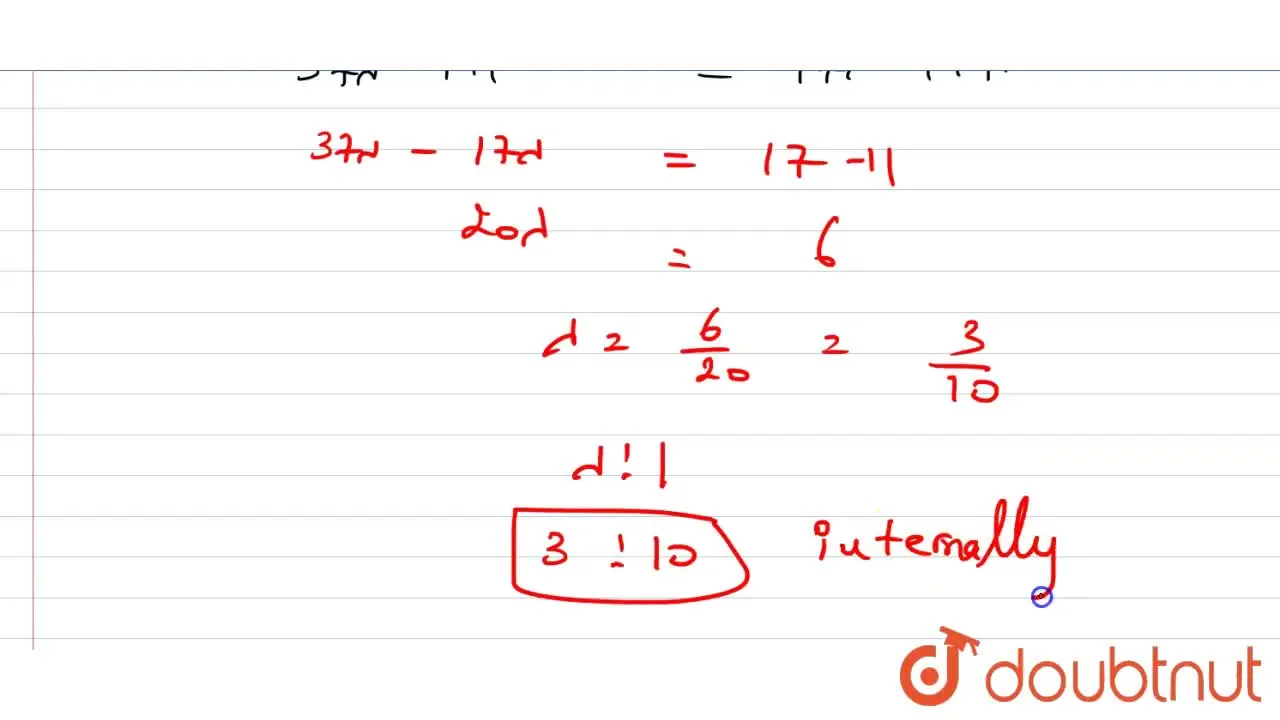
Let the plane →r.(→i−2→j+3→k)=17 divide the line
joining the points −2→i+4→j+7→kand3→i−5→j+8→k in the ration t : 1 at point P is
Therefore, point P is
3t−2t+1→i+−5t+4t+1→j+8t+7t+1→k
This lies on the given plane
∵3t−2t+1.(1)+−5t+4t+1(−2)+8t+7t+1(3)=17
Solving, we get
t=310
Related Playlists
- BOOK - CENGAGECHAPTER - THREE-DIMENSIONAL GEOMETRY EXERCISE - Exercise (Multiple)17videos
THREE-DIMENSIONAL GEOMETRY
- BOOK - CENGAGECHAPTER - THREE-DIMENSIONAL GEOMETRY EXERCISE - Exercise (Reasoning Questions)10videos
THREE-DIMENSIONAL GEOMETRY
- BOOK - CENGAGECHAPTER - THREE-DIMENSIONAL GEOMETRY EXERCISE - Exercise (Subjective)16videos
THREE-DIMENSIONAL GEOMETRY
- BOOK - CENGAGECHAPTER - THREE DIMENSIONAL GEOMETRYEXERCISE - Question Bank20videos
THREE DIMENSIONAL GEOMETRY
- BOOK - CENGAGECHAPTER - TRIGNOMETRIC RATIOS IDENTITIES AND TRIGNOMETRIC EQUATIONSEXERCISE - Question Bank34videos
TRIGNOMETRIC RATIOS IDENTITIES AND TRIGNOMETRIC EQUATIONS
Similar Questions
Find the equation of the plane through the origin and the points 4→jand2→i+→k. Find also the point in which this plane is cut by the line joining points →i−2→j+→kand3→k−2→j.
View SolutionFind the value of (→i−2j+→k)×[(2→i+→j+→k)×(→i+2→j−→k)]
View SolutionFind the angle between →a=3→i+3→j−3→k and →b=2→i+→j+3→k.
View SolutionWhat is the angle between two vectors 2→i+3→j+→kand−3→i+6→k ?
View SolutionFind the vector equation of the through the points 2→i+→j−3→k and parallel to vector →i+2→j+→k
View SolutionFind the angle between the line →r=(→i+2→j−→k)+λ(→i−→j+→k) and the normal to the plane →r⋅(2→i−→j+→k)=4.
View SolutionIf a1 and a2 are two values of a for which the unit vector a→i+b→j+12→k is linearly dependent with →i+2→j and →j−2→k, then 1a1+1a2 is equal to
View SolutionThe torque about the point 3→i−→j+3→k of a force 4→i+2→j+→k through the point 5→i+2→j+4→k is
View SolutionFind the angle between planes →r.(→i+→j)=1and→r.(→i+→k)=3.
View SolutionFind the equation of the plane through the point 2→i+3→j−→k and perpendicular to the vector 3→i−4→j+7→k.
View Solution