The perpendicular distance of a corner of uni cube from a diagonal not passing through it is
The correct Answer is:√23
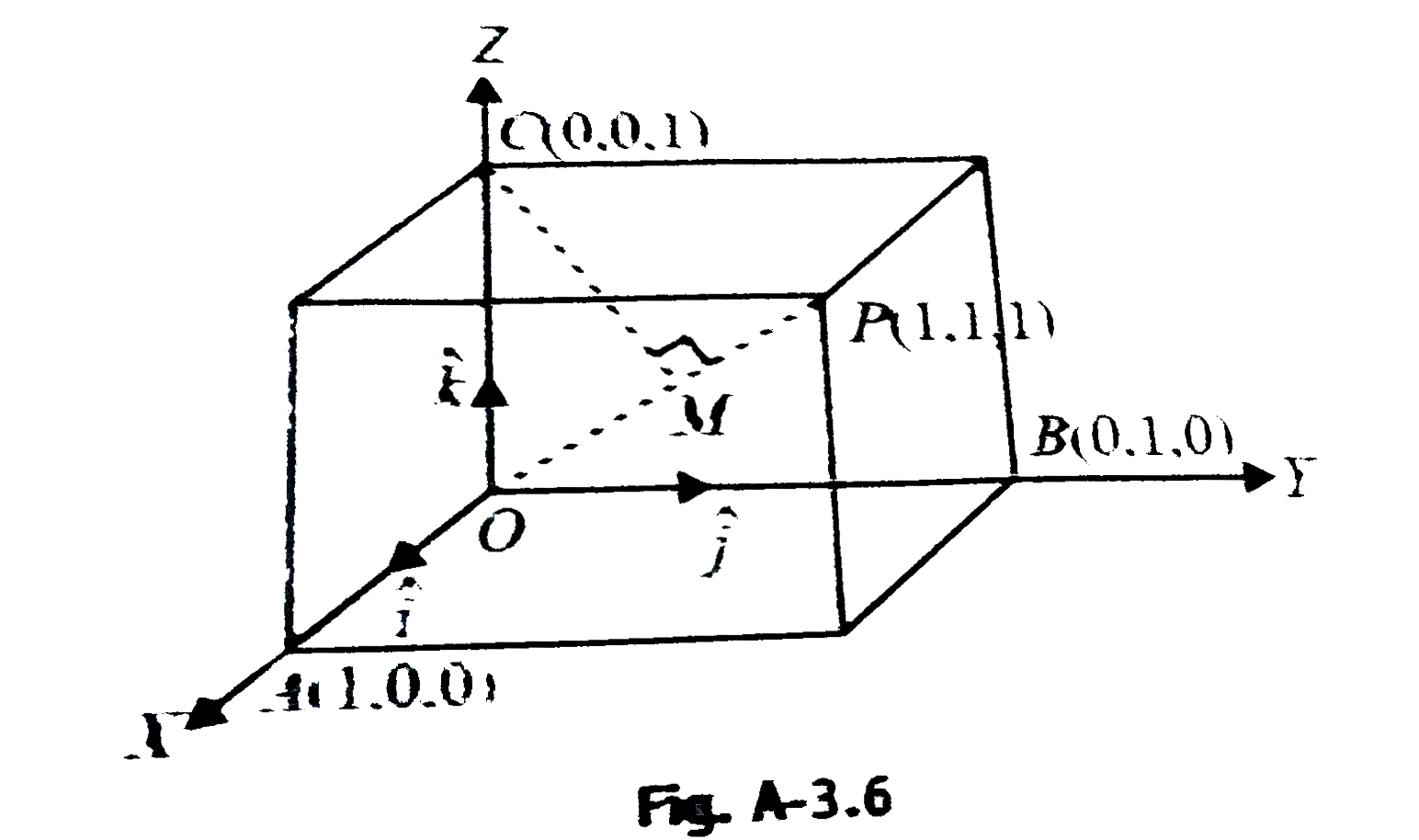
Let the edges OA, OB and OC of the unit cube be along OX, OY and OZ, respectively.
Since OA=OB=OC=1 unit,
−−→OA=ˆi,−−→OB=ˆjand−−→OC=ˆk
Let CM be perpendicular from the corner C on the diagonal OP. The vector equation of OP is
→r=λ(ˆi+ˆj+ˆk)
OM=projection of −−→OC on −−→OP=−−→OC.−−→OP∣∣∣−−→OP∣∣∣
=ˆk.(ˆi+ˆj+ˆk)√3=1√3
Now OC2=OM2+CM2
or CM2=OC2=OM2=1−13=23
or CM=√23
Topper's Solved these Questions
- THREE-DIMENSIONAL GEOMETRY BOOK - CENGAGECHAPTER - THREE-DIMENSIONAL GEOMETRY EXERCISE - Exercise (Single)
86 Videos
- THREE-DIMENSIONAL GEOMETRY BOOK - CENGAGECHAPTER - THREE-DIMENSIONAL GEOMETRY EXERCISE - Exercise (Multiple)
17 Videos
- THREE-DIMENSIONAL GEOMETRY BOOK - CENGAGECHAPTER - THREE-DIMENSIONAL GEOMETRY EXERCISE - Exercise 3.4
5 Videos
- THREE DIMENSIONAL GEOMETRYBOOK - CENGAGECHAPTER - THREE DIMENSIONAL GEOMETRYEXERCISE - Question Bank
20 Videos
- TRIGNOMETRIC RATIOS IDENTITIES AND TRIGNOMETRIC EQUATIONSBOOK - CENGAGECHAPTER - TRIGNOMETRIC RATIOS IDENTITIES AND TRIGNOMETRIC EQUATIONSEXERCISE - Question Bank
34 Videos
Similar Questions
Knowledge Check
A curve passing through (1,1) is such that the perpendicular distance of origin from normal to the curve at any point P on it is equal to the distance of P from the X-axis, then the differential equation representing the curve is
Adydx=x2−y22xyBdydx=x2+y22xyC2xydy=(y2−x2)dxDdydx=2xyx2+y2The minimum distance of origin from the plane passing through the point with position vector P and perpendicular to the lineL2 is:
A√14B7√14C11√14DNone of theseOne diagonal of a parallelogram is 40 cm and the perpendicular distance of this diagonal from either of the outlying vertices is 19 cm. The area of the parallelogram (in sq cm) is
A700 cm2B380 cm2C760 cm2D1140 cm2
Find the equation of a curve passing through the point (1.1) if the perpendicular distance of the origin from the normal at any point P(x, y) of the curve is equal to the distance of P from the x-axis.
View SolutionA curve passing through the point (1,2) has the property that the perpendicular distance of the origin from normal at any point P of the curve is equal to the distance of P from the x-axis is a circle with radius.
View SolutionThe algebraic sum of the perpendicular distances from the vertices of a triangle to a variable line is ‘O', then the line passes through the ------ of the triangle
View SolutionIf the diagonals of a cyclic quadrilateral are perpendicular to each other, show that the line passing through the point of intersection of diagonals and midpoint of a side is perpendicular to the opposite side.
View SolutionIf each line of a pair of lines passing through origin is at a perpendicular distance of 4 units from the point (3,4), then the equation of the pair of lines is
View Solution
