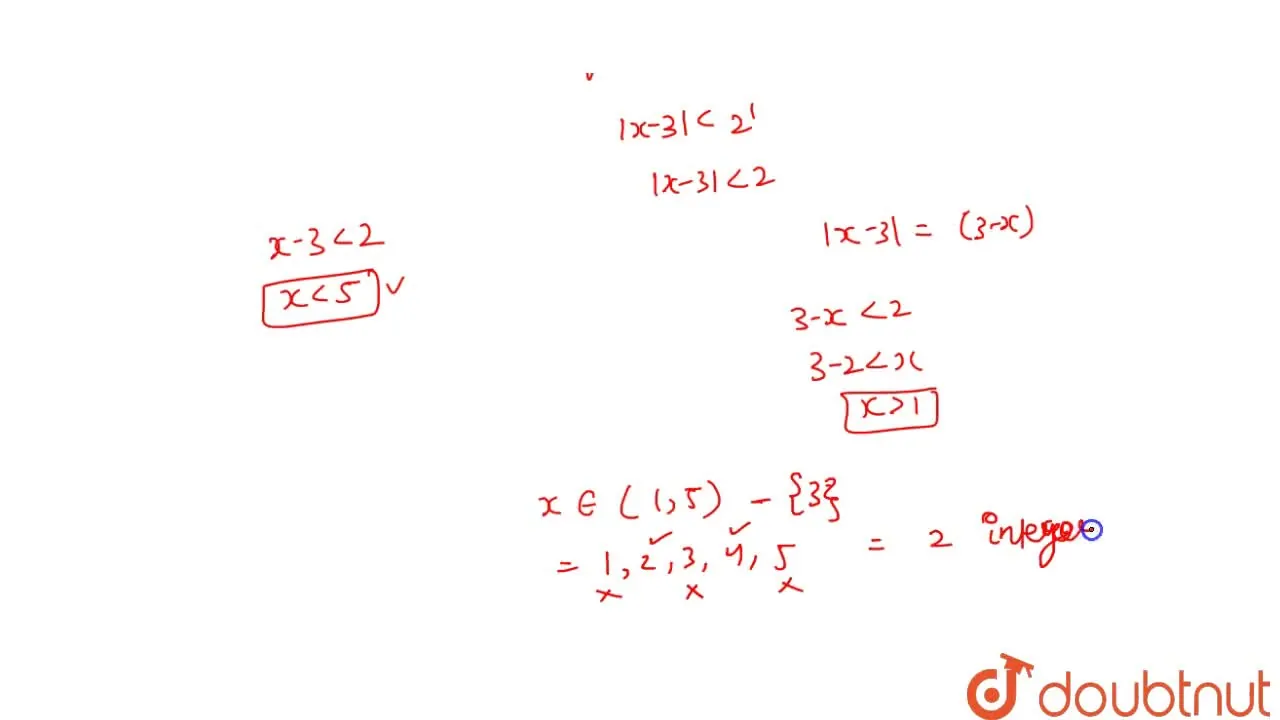Number of integers satisfying the inequality log1/2|x−3|>−1 is ________.
The correct Answer is:2
log1/2|x−3|>−1
⇒0<|x−3|<2
⇒−2<x−3<2,x≠3
⇒1<x<5,x≠3
∴x∈{2,4}
Related Playlists
- BOOK - CENGAGECHAPTER - LOGARITHM AND ITS PROPERTIESEXERCISE - JEE Previous Year5videos
LOGARITHM AND ITS PROPERTIES
- BOOK - CENGAGECHAPTER - LOGARITHM AND ITS PROPERTIESEXERCISE - Exercise (Matrix)3videos
LOGARITHM AND ITS PROPERTIES
- BOOK - CENGAGECHAPTER - LOGARITHM AND ITS APPLICATIONS EXERCISE - Subjective Type9videos
LOGARITHM AND ITS APPLICATIONS
- BOOK - CENGAGECHAPTER - MATHMETICAL REASONINGEXERCISE - JEE Previous Year10videos
MATHMETICAL REASONING
Similar Questions
Number of integers satisfying the inequality (log)12|x−3|≻1 is.....
View SolutionNumber of integers satisfying the inequality log(x+3)(x2−x)<1 is
View SolutionNumber of integers satisfying the inequality
log2√x−2log21/4x+1>0,isView SolutionNumber of integers satisfying the inequality
(13)|x+2|2−|x|<9 isView SolutionNumber of integers ≤10 satisfying the inequality 2log1/2(x−1)≤13−1logx2−x8 is ________.
View SolutionNumber of integers satisfying the inequality (13)|x+2|2−|x|>9 is
View SolutionNumber of integers satisfying the inequality log(x+3)/(x−3)4<2[log1/2(x−3)−log√2/2√x+3] is greater than (A)6 (B)5 (C)4 (D) 3
View SolutionNumber of integers ≤10 satisfying the inequality 2(log)12(x−1)≤13−1(log)x2−x8 is............
View SolutionNumber of integers ≤ 10 satisfying the inequality 2log12(x−1)≤13−1logx2−x8 is :
View SolutionThe number of integers satisfying the inequality is xx+6≤1x
View Solution