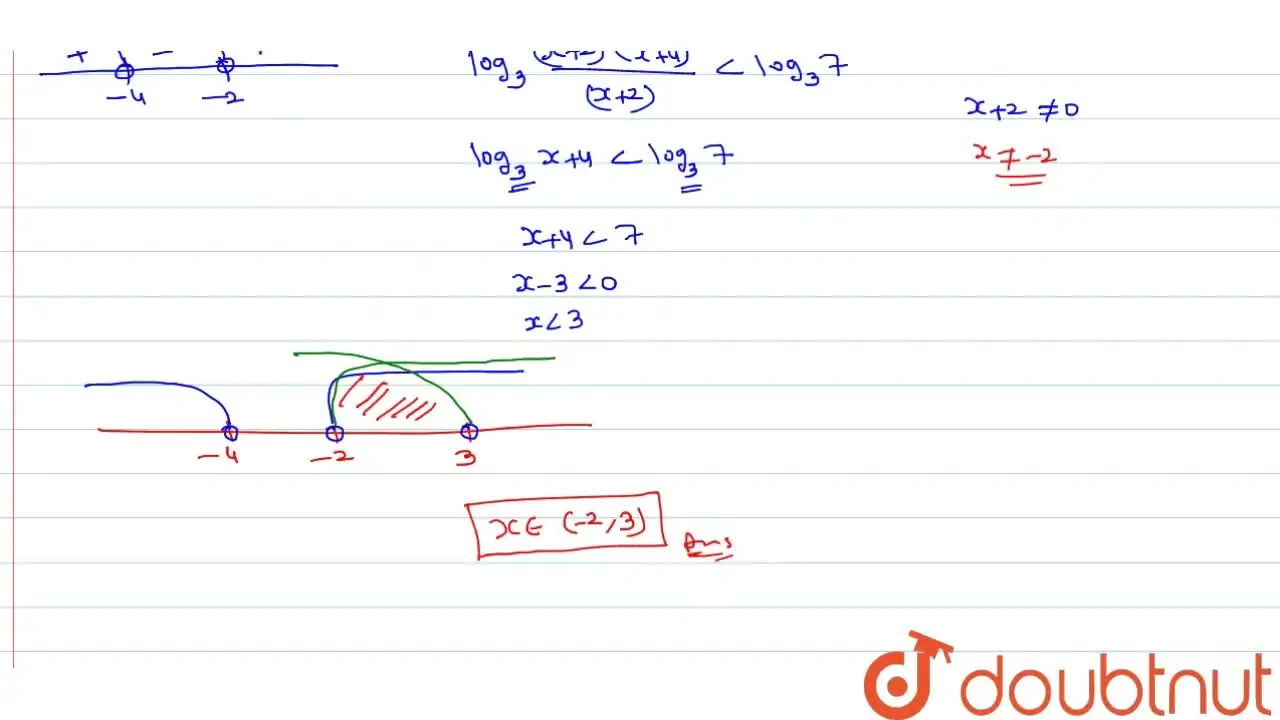
Solve log3(x+2)(x+4)+log1/3(x+2)<12log√37.
Video Solution
Text Solution
The correct Answer is:x∈(−2,3)
(x+2)(x+4)>0,x+2>0
⇒x>−2
Given inequality is
log3(x+2)(x+4)−log3(x+2)<(log7)/2(log3)/2
⇒log3(x+4)<log37
⇒x+4<7orx<3
⇒x∈(−2,3)
|
Updated on:21/07/2023
Related Playlists
- BOOK - CENGAGECHAPTER - LOGARITHM AND ITS PROPERTIESEXERCISE - Exercise 1.66videos
LOGARITHM AND ITS PROPERTIES
- BOOK - CENGAGECHAPTER - LOGARITHM AND ITS PROPERTIESEXERCISE - Exercise (Single)50videos
LOGARITHM AND ITS PROPERTIES
- BOOK - CENGAGECHAPTER - LOGARITHM AND ITS PROPERTIESEXERCISE - Exercise 1.412videos
LOGARITHM AND ITS PROPERTIES
- BOOK - CENGAGECHAPTER - LOGARITHM AND ITS APPLICATIONS EXERCISE - Subjective Type9videos
LOGARITHM AND ITS APPLICATIONS
- BOOK - CENGAGECHAPTER - MATHMETICAL REASONINGEXERCISE - JEE Previous Year10videos
MATHMETICAL REASONING
Similar Questions
Solve: log13(x−1)>log134
View SolutionSolve logx+3(x2−x)<1.
View SolutionSolve (log)2(3x−2)=(log)12x
View SolutionSolve log2.x−42x+5<1.
View SolutionSolve log1/3(x2−3x+5)<−1
View SolutionSolve log4(x−1)=log2(x−3).
View SolutionSolve log2(2√17−2x)=1−log1/2(x−1).
View SolutionSolve : 2(log)3x−4(log)x27≤5 (x>1)
View SolutionSolve for x:(a)log0.3(x2+8)>log0.3(9x), b) log7(2x−62x−1)>0
View SolutionSolve logx−2(3x2−x−1)>0.
View Solution