Consider the set of all lines px+qy+r=0 such that 3p+2q+4r=0. Which one of the following statements is true ?
The lines are all parallel.
Each line passes through the origin.
The lines are not concurrent.
The lines are concurrent at the point (34,12).
The correct Answer is:D
To solve the problem, we need to analyze the given equations and determine the relationship between the lines defined by px+qy+r=0 and the condition 3p+2q+4r=0.
1. Understanding the Equations:
We have the equation of a line given by:
px+qy+r=0
and a condition on the coefficients:
3p+2q+4r=0
2. Expressing r:
From the condition 3p+2q+4r=0, we can express r in terms of p and q:
4r=−3p−2q⟹r=−34p−12q
3. Substituting r into the Line Equation:
Substitute r back into the line equation:
px+qy−(34p+12q)=0
This simplifies to:
px+qy−34p−12q=0
Rearranging gives:
px+qy=34p+12q
4. Finding the Points of Intersection:
To find the points where these lines intersect, we can set the coefficients of p and q to zero:
4x−3=0and2y−1=0
5. Solving for x and y:
From 4x−3=0:
x=34
From 2y−1=0:
y=12
6. Conclusion:
The lines px+qy+r=0 defined by the condition 3p+2q+4r=0 are concurrent at the point:
(34,12)
Thus, the correct statement is that the lines are concurrent at the point (34,12).
Final Answer:
The lines are concurrent at the point (34,12).
---
We have the equation of a line given by:
px+qy+r=0
and a condition on the coefficients:
3p+2q+4r=0
From the condition 3p+2q+4r=0, we can express r in terms of p and q:
4r=−3p−2q⟹r=−34p−12q
Substitute r back into the line equation:
px+qy−(34p+12q)=0
This simplifies to:
px+qy−34p−12q=0
Rearranging gives:
px+qy=34p+12q
To find the points where these lines intersect, we can set the coefficients of p and q to zero:
4x−3=0and2y−1=0
From 4x−3=0:
x=34
From 2y−1=0:
y=12
The lines px+qy+r=0 defined by the condition 3p+2q+4r=0 are concurrent at the point:
(34,12)
Thus, the correct statement is that the lines are concurrent at the point (34,12).
The lines are concurrent at the point (34,12).
Topper's Solved these Questions
- JEE 2019BOOK - CENGAGECHAPTER - JEE 2019EXERCISE - Chapter 4 (Circle)
10 Videos
- JEE 2019BOOK - CENGAGECHAPTER - JEE 2019EXERCISE - Chapter 5 (Parabola)
8 Videos
- JEE 2019BOOK - CENGAGECHAPTER - JEE 2019EXERCISE - Chapter 10
9 Videos
- INVERSE TRIGONOMETRIC FUNCTIONSBOOK - CENGAGECHAPTER - INVERSE TRIGONOMETRIC FUNCTIONSEXERCISE - Question Bank
24 Videos
- LIMITSBOOK - CENGAGECHAPTER - LIMITSEXERCISE - Question Bank
30 Videos
Similar Questions
Using the properties of determinats, prove that
∣∣ ∣ ∣ ∣ ∣∣(a+b)2ccca(b+c)2aabb(c+a)2b∣∣ ∣ ∣ ∣ ∣∣=2(a+b+c)3.
Or, If p≠0,q≠0and∣∣ ∣ ∣∣pqpα+qqrqα+rpα+qqα+r0∣∣ ∣ ∣∣=0 then using the propetries of eterminants, prove that at least one of the following statements is true:
(a) p,q,r are in GP (b) α is a root of the equation px2+2qx+r=0.
View SolutionConsider the cubic equation x3+px2+qx+r=0, where p, q, r are real numbers, which of the following statement is correct?
View Solution
Knowledge Check
Given that P+Q+R=0. Which of the following statement is true?
A|P|+|Q|=|R|B|P+Q|=|R|C|P|−|Q|+|R|D|P−Q|=|R|If p, q are true and r is false statement then which of the following is true statement?
A(p∧q)∨ r is FB(p∧q)∧r is TC(p∨q)∧(p∨r) is TD(p∧q)∨(p∧r)is FConsider the following statements in respect of the quadratic equation 4(x−p)(x−q)−r2=0 where p.q.r are real numbers
1. The roots are real
2. The roots are equal if p = q and r = 0 Which of the above statements is/are correct ?A1 onlyB2 onlyCBoth 1 and 2DNeither 1 nor 2
Consider a triangle PQR having sides of lengths p,q,r opposite to the angles P,Q,R respectively. Then which of the following statements is (are) true?
View SolutionConsider the given question and decide which of the following statements is sufficient to answer the question. What are the values of p, q and r? Statements: 1. (p+q)2=16 2. p−q=4,r=2p
View SolutionIf p, q are true and r is a false statement, then which of the following is a true statement?
View SolutionShown below are the graphs of the lines y−2x=0,x+y=6andpx+qy=r
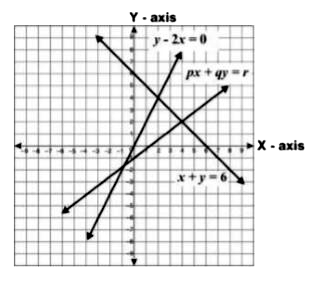
Which of these is the solution for the pair of equations x+y=6 and px+qy=r
View SolutionIf one root of the quadratic equation x2+px+q=0 is 2−√3 : where P,Q⊂Q.Then which of the following is true ?
View Solution
