PARAGRAPH A There are five students S1, S2, S3, S4 and S5 in a music class and for them there are five seats R1, R2, R3, R4 and R5 arranged in a row, where initially the seat Ri is allotted to the student Si, i=1, 2, 3, 4, 5 . But, on the examination day, the five students are randomly allotted five seats. (For Ques. No. 17 and 18) The probability that, on the examination day, the student S1 gets the previously allotted seat R1 , and NONE of the remaining students gets the seat previously allotted to him/her is 340 (b) 18 (c) 740 (d) 15
340
18
740
15
The correct Answer is:A
Total number of cases = 5!
Since S1 gets seat R1 and none of the other gets previously allotted seat, we have derangement of 4 students. So, required probability
=4!(1−11!+12!−13!+14!)5!=9120=340
Topper's Solved these Questions
Similar Questions
There are five students S1, S2, S3, S4 and S5 in a music class and for them there are five seats R1, R2, R3, R4 and R5 arranged in a row, where initially the seat Ri is allotted to the student Si, i=1, 2, 3, 4, 5 . But, on the examination day, the five students are randomly allotted five seats. The probability that, on the examination day, the student S1 gets the previously allotted seat R1 , and NONE of the remaining students gets the seat previously allotted to him/her is 340 (b) 18 (c) 740 (d) 15
View SolutionPARAGRAPH A There are five students S1, S2, S3, S4 and S5 in a music class and for them there are five seats R1, R2, R3, R4 and R5 arranged in a row, where initially the seat Ri is allotted to the student Si, i=1, 2, 3, 4, 5 . But, on the examination day, the five students are randomly allotted five seats. For i=1, 2, 3, 4, let Ti denote the event that the students Si and Si+1 do NOT sit adjacent to each other on the day of the examination. Then, the probability of the event T1∩T2∩T3∩T4 is 115 (b) 110 (c) 760 (d) 15
View Solution
Knowledge Check
There are five students S1,S2,S3,S4 and R5 arranged in a row, where initially the seat R1is allotted to the students are randomly allotted the five seats R1,R2,R3,R4 and R6 arranged in a row, where initially the seat Ri is allotted to the student Sii,=1,2,3,4,5.But,ontheexaminationday,thefivestudentsarerandomlyalloedthefiveseats.<br>(TherearetwoquestionsbasedonParagraph,thequestiongivenbelowiso≠ofthem)<br>Theprobabilitytˆ,ontheexaminationday,thestudentS_(1)≥tsthepreviouslyalloedseatR_(1), and NONE of the remaining students gets the seat previously allotted to him/her is
A340B18C740D15There are five students S1,S2,S3,S4 and R5 arranged in a row, where initially the seat R1is allotted to the students are randomly allotted the five seats R1,R2,R3,R4 and R6 arranged in a row, where initially the seat Ri is allotted to the student Sii,=1,2,3,4,5.But,ontheexaminationday,thefivestudentsarerandomlyalloedthefiveseats.<br>(TherearetwoquestionsbasedonParagraph,thequestiongivenbelowiso≠ofthem)<br>Fori=1,2,3,4.≤tT_(i)de¬etheeventtˆthestudentsS_(1)andS_(i+1)doNOTsitadjacent→eachotheronthedayoftheexamination.Then,theprobabilityoftheeventT_(1) cap T_(2) cap T_(3) cap T_(4) is
A115B110C760D16Five students are sitting in a row. S is on the right of L, P is on the left of L but is on the right of K, S is on the left of Q. The first student in the row from the left is
AKBLCpDS
Ten students are seated at random in a row. The probability that two particular students are not seated side by side is 4/5.
View SolutionThe following pie-chart shows the percentage-wise distribution of the number of students in five different schools P, Q, R, S and T. The total number of students in all five schools together is 8400.
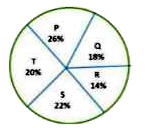
Find the average number of students in schools R and S together.
View SolutionThere are five students - P, Q, R, S and T having different heights in a class. P's height is more than only one student. Q's height is more than S and P but not more than R. S's height is more than P. R is not the smallest. Who is having the maximum height in the class?
View SolutionFive particles have speeds 1, 2, 3, 4, 5 m/s. The average velocity of the particles is (in m/s)
View SolutionP, Q, R, S, and T are five numbers in ascending order. Their median is M. Find the median of Q/3, R/3, and S/3.
View Solution
