Two bodies of masses m1andm2(m2>m1) are connected by a light inextensible string which passes through a smooth fixed pulley. The instantaneous power delivered by an external agent to pull m1 with constant velocity v is :
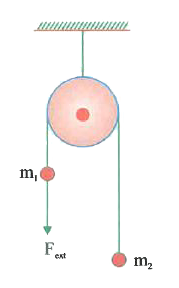
(m2−m1)gv
(m2−m1)vg
(m2−m1)gv
(m1−m2)gv
The correct Answer is:(3)
Fext+m1g=T&T=m2g
⇒Fext=T−m1g
⇒Fext=(m2−m1)g
∴P=FextV=(m2−m1)gV
Topper's Solved these Questions
- WORK , ENERGY & POWER BOOK - NARAYNACHAPTER - WORK , ENERGY & POWER EXERCISE - EXERCISE -III
54 Videos
- WORK , ENERGY & POWER BOOK - NARAYNACHAPTER - WORK , ENERGY & POWER EXERCISE - EXERCISE IV
43 Videos
- WORK , ENERGY & POWER BOOK - NARAYNACHAPTER - WORK , ENERGY & POWER EXERCISE - EXERCISE -II (C.W)
75 Videos
- WAVESBOOK - NARAYNACHAPTER - WAVESEXERCISE - Exercise-IV
56 Videos
- WORK POWER AND ENERGYBOOK - NARAYNACHAPTER - WORK POWER AND ENERGYEXERCISE - Level-VI (Integer)
12 Videos
Similar Questions
(i) Two bodies of masses m1(m2>m1) are connected by a light inextensible string which passes through a smooth fixed pulley. What is the instantaneous power delivered by an external agent to pull m1 with constant velocity →V?

(ii) A small body of mass m is located on a horizontal plane at any point O. The body acquires a horizontal velocity v0 . Find the mean power developed by the friction force during the motion, if the coefficient of friction μ=0.27,m=1.0 kg and v0=1.5m/s.
View SolutionTwo blocks of masses m1andm2(m1>m2) connected by a massless string passing over a frictionless pulley Magnitude of acceleration of blocks would be
View Solution
Knowledge Check
Two masses m1 and m2 are connected by light inextensible string passing over a smooth pulley P. If the pulley moves vertically upwards with an acceleration equal to g then
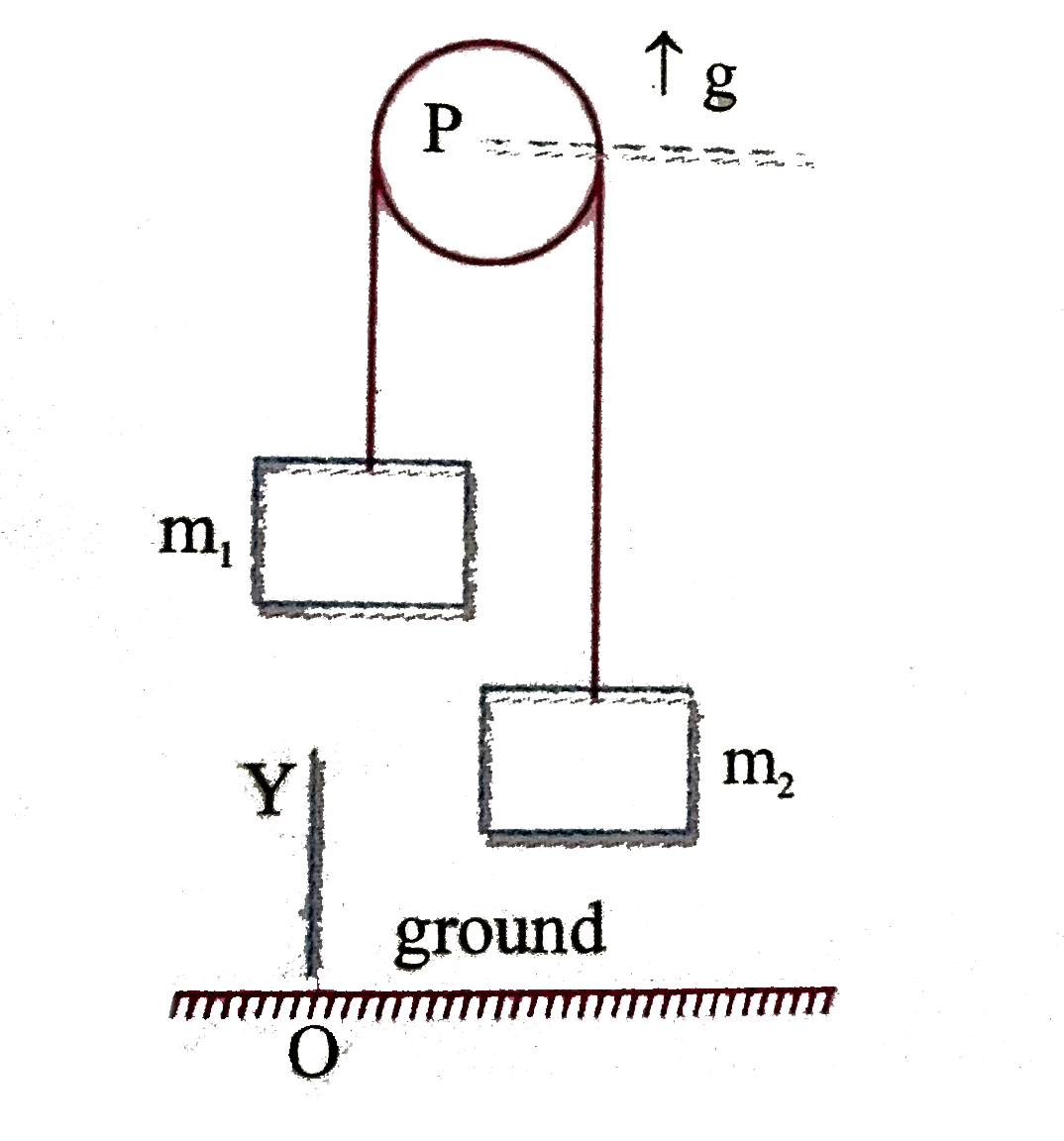 .ATension on the string is 4m1m2gm1+m2BTension on the string is 2m1m2gm1+m2CThe acceleration of mass m1 with respect to ground is 3m2−m1m1+m2g .DThe acceleration of mass m1 with respect to ground is 2(m2−m)m1+m2g
.ATension on the string is 4m1m2gm1+m2BTension on the string is 2m1m2gm1+m2CThe acceleration of mass m1 with respect to ground is 3m2−m1m1+m2g .DThe acceleration of mass m1 with respect to ground is 2(m2−m)m1+m2gTwo particles of mass m1 and m2(m1>m2) are attached to the ends of a light inextensible string passing over a smooth fixed pulley. When the level of m1 is higher than that of m2 by 49 cm and string is just taut m2 is released. 0.5 s after motion started the masses are at the same level. the ratio of m1 to m2 is (Take g=9.8ms−2)
A7:3B4:3C3:2D2:1Two blocks of mass m1andm2 are connected by light inextensible string passing over a smooth fixed pulleuy of negligible mass. The acceleration of the centre of mass of the system when blocks move under gravity is :
A(m2−m1m1+m2)gB(m1+m2m1−m2)gC(m1+m2m1−m2)gD(m1−m2m1+m2)2g
Two blocks of masses m1 = 3 kg and m2=1√3 kg are connected by a light inextensible string which passes over a smooth peg . The blocks rest on the inclined smooth planes of a wedge and the peg is fixed to the top of the wedge . The planes of the wedge supporting m1 and m2 are inclined at 30∘ and 60∘ respectively , with the horizontal. Calculate the acceleration of masses and the tension in the string .
View SolutionTwo particles of masses m1 and m2 are connected by a light and inextensible string which passes over a fixed pulley. Initially, the particle m1 moves with velocity v0 when the string is not taut. Neglecting friction in all contacting surface, find the velocities of the particles m1 and m2 just after the string is taut.

View SolutionTwo blocks of masses m1 and m2(m1>m2) are connected by a massless threads, that passes over a massless smooth pulley. The pulley is suspended from the ceiling of an elevator. Now the elevator moves up with uniform velocity v0 Now, select the correct options:
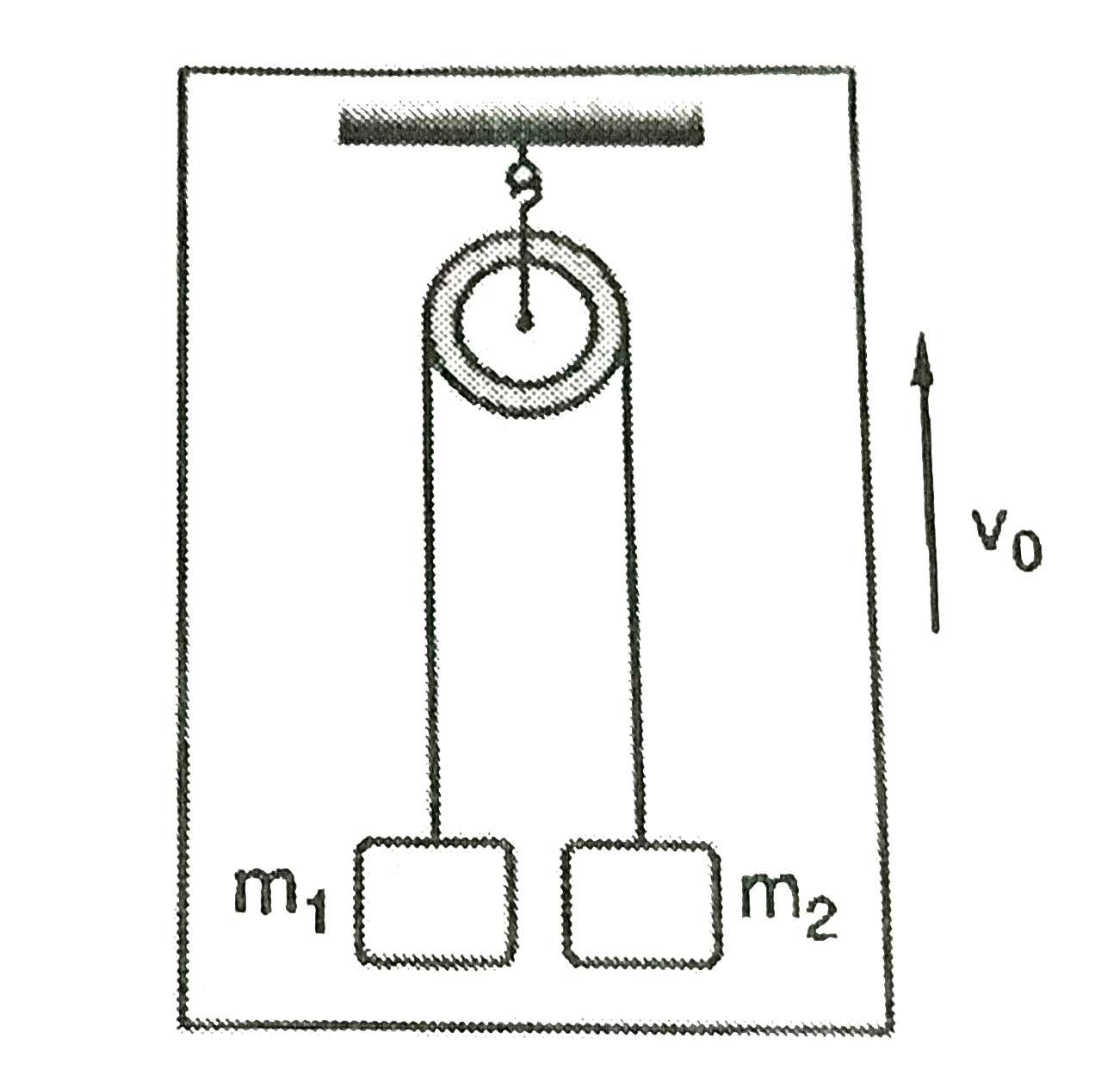
View SolutionTwo masses m and m (m>m) are connected by massless flexible and inextensible string passed over massless and frictionless pulley. The acceleration of centre of mass is
View SolutionTwo masses M and m(M>m) are joined by a light string passing over a smooth light pulley.

View Solution
