Question

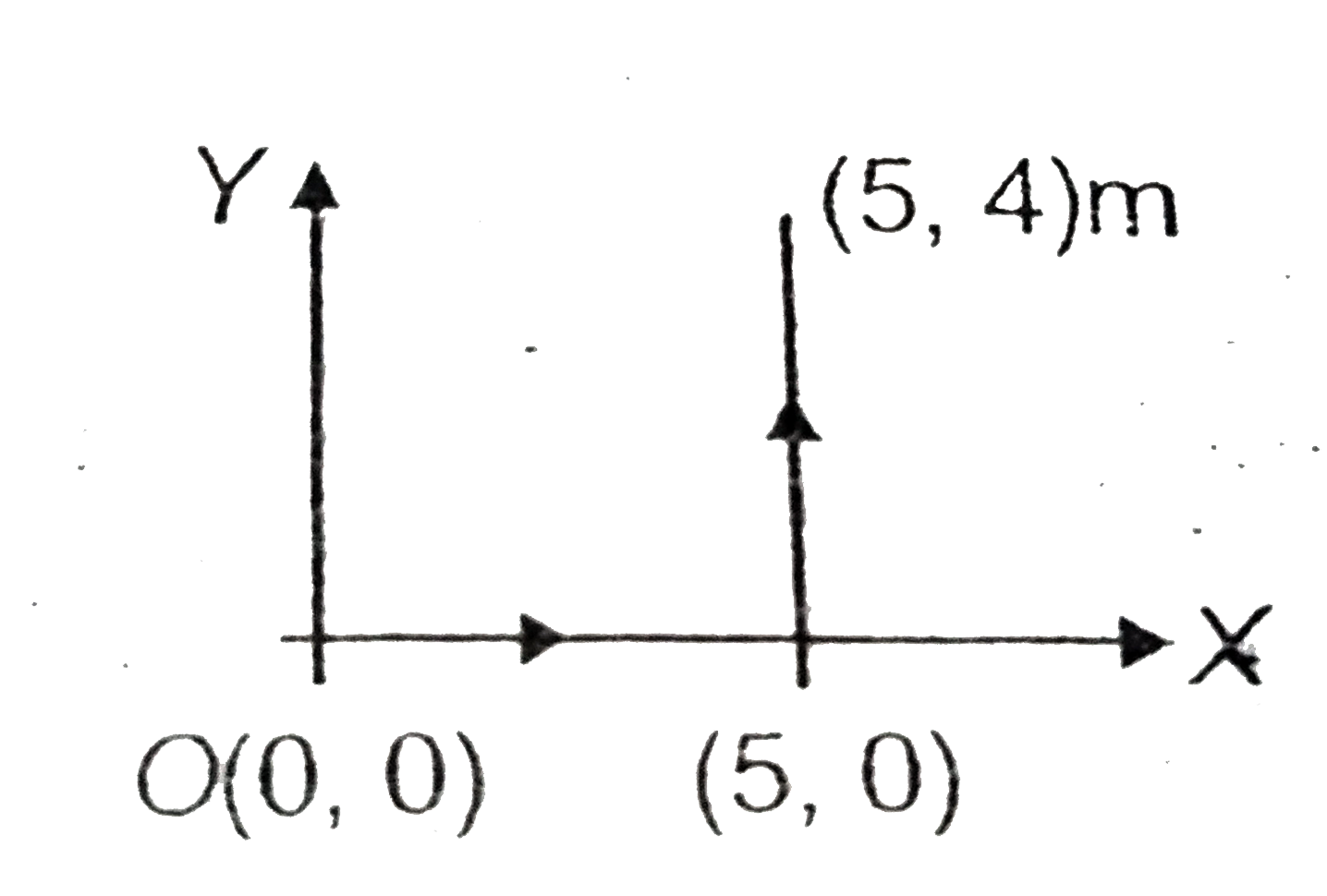
A force $\overrightarrow{F}=(3\overrightarrow{i}+4\overrightarrow{j})N$, acts on a particle moving in x-y plane. Starting from origin, the particle first goes along x-axis to thepoint (4,0)m and then parallel to the y -axis to the point(4, 3)m. The total work done by the force on the particle is
(##NAR_NEET_PHY_XI_P2_C06_E09_014_Q01.png" width="80%">

Options

A. `+12 J`
B. `-6 J`
C. `+24 J`
D. `-12 J`

Answer

`(3)`

Explanation

To solve the problem, we need to calculate the total work done by the force $$\overrightarrow{F}$$ on the particle as it moves from the origin to the point (4, 3) m. The force is given as $$\overrightarrow{F}=3\hat{i}+4\hat{j}$$ N.

1. Identify the Displacement Vector:
The particle moves from the origin (0, 0) to the point (4, 0) along the x-axis and then from (4, 0) to (4, 3) along the y-axis. The total displacement vector \(\vec{S}\) can be calculated as:
\( \vec{S} = (4 - 0)\hat{i} + (3 - 0)\hat{j} = 4\hat{i} + 3\hat{j} \)

2. Calculate the Work Done:
The work done \(W\) by a constant force is given by the dot product of the force vector \(\vec{F}\) and the displacement vector \(\vec{S}\):
\( W = \vec{F} \cdot \vec{S} \)
Substituting the values of \(\vec{F}\) and \(\vec{S}\):
\( W = (3\hat{i} + 4\hat{j}) \cdot (4\hat{i} + 3\hat{j}) \)

3. Calculate the Dot Product:
The dot product is calculated as follows:
\( W = (3 \cdot 4)(\hat{i} \cdot \hat{i}) + (4 \cdot 3)(\hat{j} \cdot \hat{j}) + (3 \cdot 3)(\hat{i} \cdot \hat{j}) + (4 \cdot 4)(\hat{j} \cdot \hat{i}) \)
Since \(\hat{i} \cdot \hat{j} = 0\) (the unit vectors are perpendicular), we have:
\( W = 12 + 12 + 0 + 0 = 24 \text{ Joules} \)

4. Conclusion:
The total work done by the force on the particle as it moves from the origin to the point (4, 3) m is:
\( W = 24 \text{ Joules} \)