A ball is dropped from the certain height on the surface of glass. It is collide elastically the comes back to initial position. If this process it repeated then velocity time graph is:-
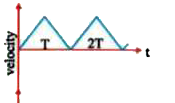
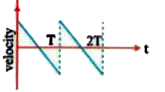
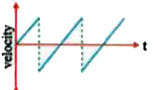

The correct Answer is:C
Step by step video & text solution for A ball is dropped from the certain height on the surface of glass. It is collide elastically the comes back to initial position. If this process it repeated then velocity time graph is:- by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Similar Questions
A ball is dropped from a certain height on a horizontal surface.It bounces up and down,finally comes to rest.If the time taken for first fall is 10s, then find the time taken to come to rest.(e=0.2)
View SolutionA body is dropped vertically from a certain height. Draw velocity time graph of the body.
View Solution
Knowledge Check
A ball is dropped from certain height on a glass floor so that it rebounds elastically to the same height. If the process continues, the velocity -time graph for such a motion would be -
 AIBiiCiiiDiv
AIBiiCiiiDivA body is dropped from a height equal to radius of the earth. The velocity acquired by it before touching the ground is
Adirectly proportional to the time of fall.Bdirectly proportional to the square of the time o fall.Cdirectly proportional to mass of the body.DBoth (a) and (c)A small ball is dropped from a certain height on the surface of a non-viscous liquid of density less than the density of ball. The motion of the ball is
ASHMBperiodic but not SHMCnot periodicDSHM for half a period and non-periodic for rest half of the period
A 10-kg ball is dropped from a height of 10 m. Find the initial potential of the ball.
View SolutionA ball of density d is dropped on to a horizontal solid surface. It bounces elastically from the surface and returns to its original position in a time t1. Next, the ball is released and it falls through the same height before striking the surface of a liquid of density of dL
(a) If d<dL, obtain an expression (in terms of d, t1 and dL) for the time t2 the ball takes to come back to the position from which it was released.
(b) Is the motion of the ball simple harmonic?
(c) If d=dL, how does the speed of the ball depend on its depth inside the liquid? Neglect all frictional and other dissipative forces. Assume the depth of the liquid to be large.
View SolutionA ball is dropped from a height h on a inclined plane, ball collides elastically with the first plane. Find the value of 'h' (in meter) so that it strikes perpendicular on the second inclined plane.
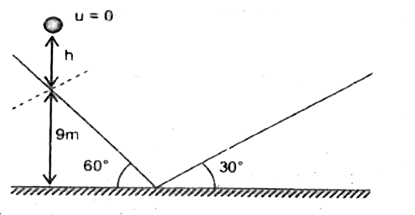
View SolutionWhen a ball is dropped from a certain height the speed of the ball goes on increasing due to
View SolutionA ball is dropped from height 'H' onto a horizontal surface. If the coefficient of restitution is 'e' then the total time after which it comes to rest is
View Solution
