The table that follows shows some measurements of the decay rate of a sample of $.^{128} I$ , a radio nuclide often used medically as a tracer to measure the rate at which iodine is absorbed by the thyroid gland.
$∣ ∣ ∣ ∣ ∣ ∣ ∣ ∣ \begin{matrix} Time(min) & A (c o u n t s / s) & Time(min) & A (c o u n t s) / s 4 & 392.2 & 132 & 10.9 36 & 161.4 & 164 & 4.56 68 & 65.5 & 196 & 1.86 10 & 26.8 & 218 & 1.00 \end{matrix} ∣ ∣ ∣ ∣ ∣ ∣ ∣ ∣$
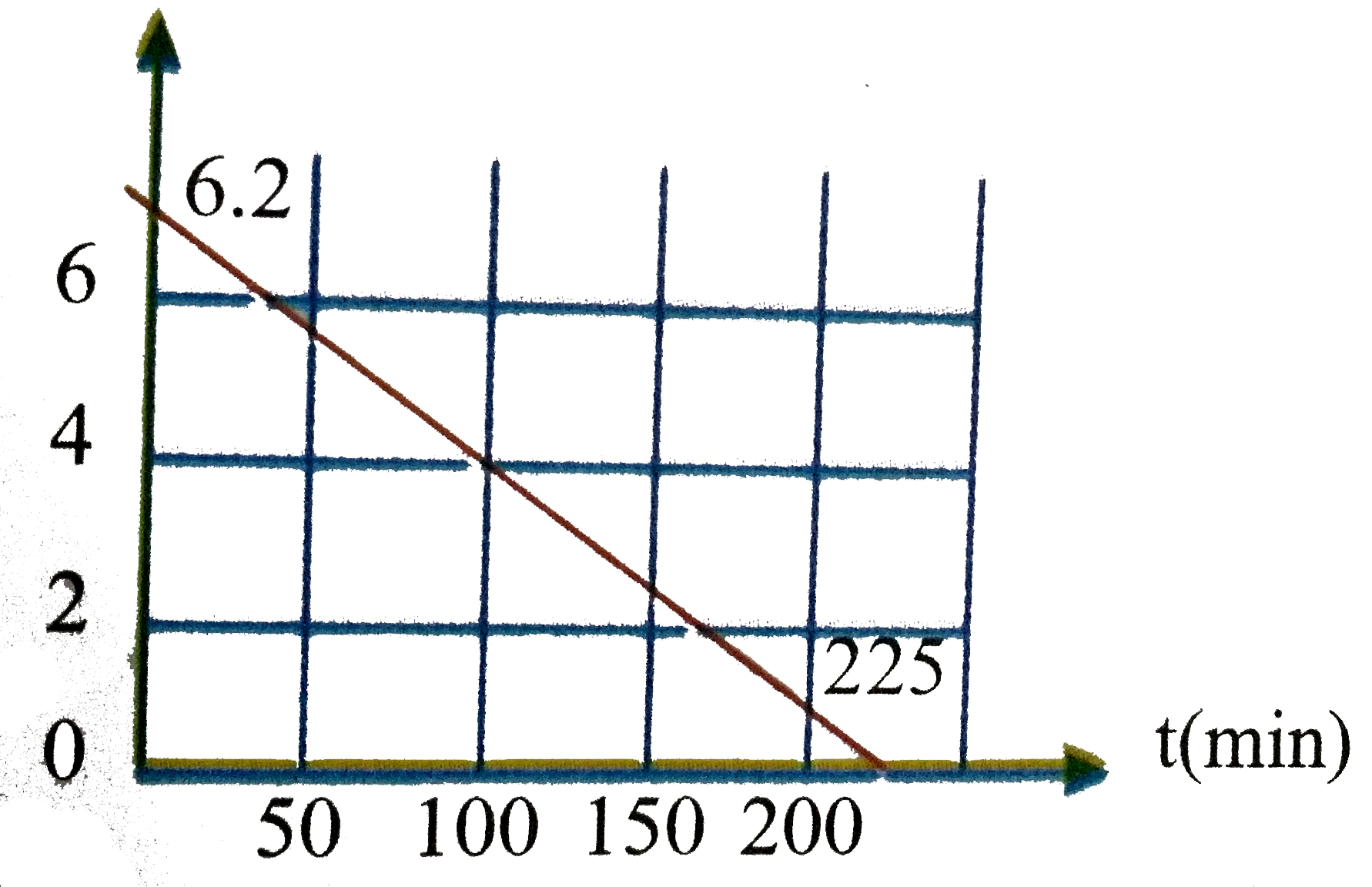
$The table that follows shows some measurements of the decay rate of a sample of .^(128)I, a radio nuclide often used medically as a tracer to measure the rate at which iodine is absorbed by the thyroid gland. |{:($
The half life $t_{1 / 2}$ for this radio nuclide.

25 min

50 min

$2.5$ min

5 min

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A

Updated on:21/07/2023

The half-life of a sample of a radioactive substance is $1$ hour. If $8 \times 10^{10}$ atoms are present at $t = 0$ , then the number of atoms decayed in the duration $t = 2$ hour to $t = 4$ hour will be
A $2 \times 10^{10}$
B $1.5 \times 10^{10}$
CZero
DInfinity
The half-life of a sample of a radioactive substance is $1$ hour. If $8 \times 10^{10}$ atoms are present at $t = 0$ , then the number of atoms decayed in the duration $t = 2$ hour to $t = 4$ hour will be
A $2 \times 10^{10}$
B $1.5 \times 10^{10}$
Czero
Dinfinity
A first order reaction is found to have a rate constant, $k = 4.2 \times 10^{- 12} s^{- 1}$ . Find the half-life of the reaction.
A $1.26 \times 10^{13} s$
B $1.65 \times 10^{11} s$
C $1.65 \times 10^{- 11} s$
D $1.26 \times 10^{- 13} s$