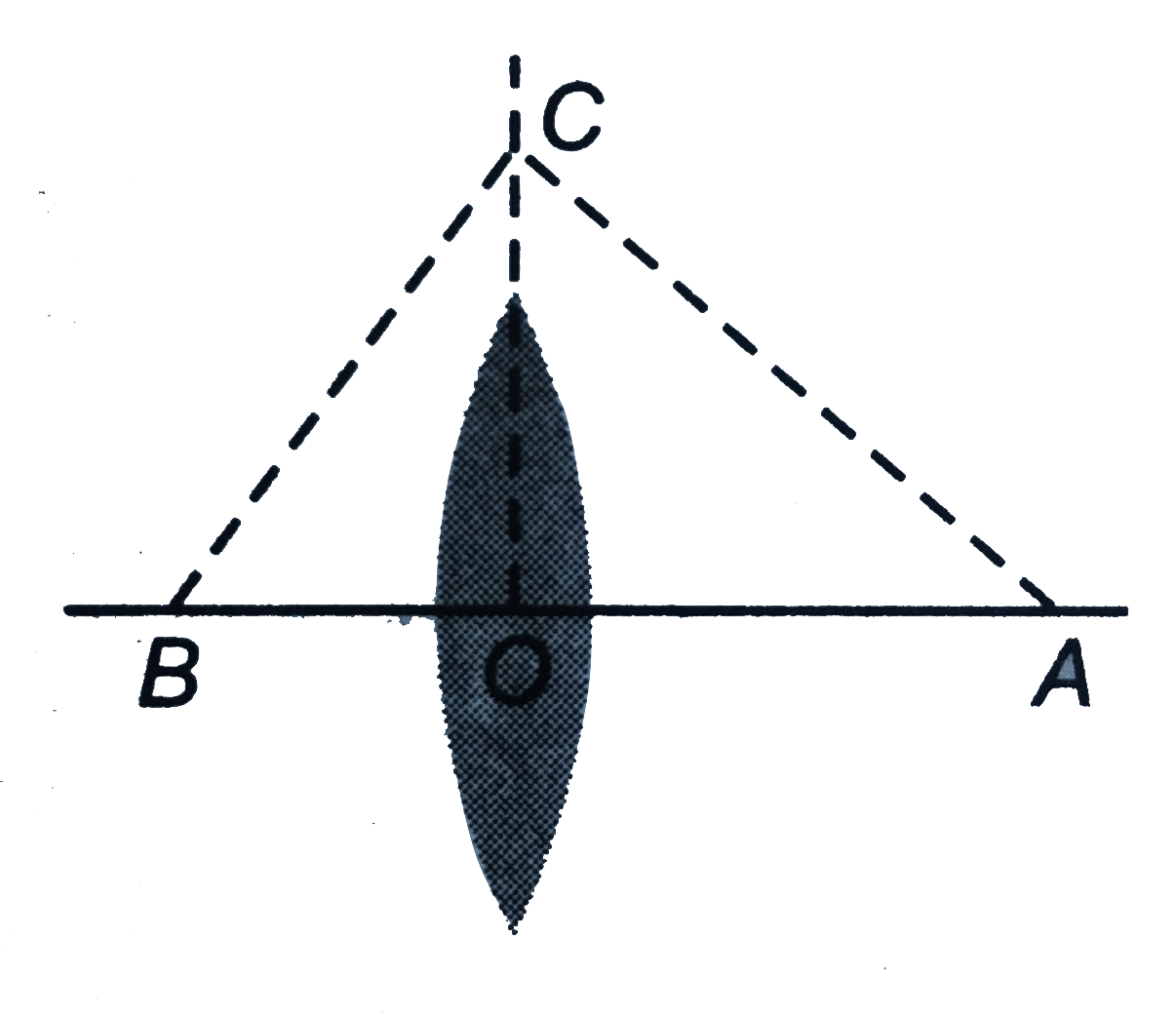
An object is placed at A(OA>f). Here, f is the focal length of the lens. The image is formed at B. A perpendicular is erected at O and C is chosen such that ∠BCA=90∘. Let OA = a, OB = b and OC = c. Then the value of f is
From 1.v−1u=1f we have 1b+1a=1f
or f=aba+b→(1)
further AC2+BC2=AB2
or (a2+c2)+(b2+c2)=(a+b)2
ab=c2
Substituting this in Eq. (1) we get f= c2a+b

Topper's Solved these Questions
- BOOK - NARAYNACHAPTER - RAY OPTICS AND OPTICAL INSTRAUMENTS EXERCISE - CUQ (REFLECTION)9videos
RAY OPTICS AND OPTICAL INSTRAUMENTS
- BOOK - NARAYNACHAPTER - RAY OPTICS AND OPTICAL INSTRAUMENTS EXERCISE - CUQ (REFRECTION)28videos
RAY OPTICS AND OPTICAL INSTRAUMENTS
- BOOK - NARAYNACHAPTER - NUCLEI EXERCISE - ASSERTION & REASON5videos
NUCLEI
- BOOK - NARAYNACHAPTER - SEMI CONDUCTOR DEVICESEXERCISE - Level-II (H.W)36videos
SEMI CONDUCTOR DEVICES
Similar Questions
Knowledge Check
- Question 1 - Select One
If an object is placed at A(OA>f), where f is the focal length of the lens, the image is formed at B. A perpendicular is erected at O and C is chosen on it such that the angle (∠BCA) is a right angle. Then, the value of f will be
 AABOC2B(AC)(BC)OCCOC2ABD(OC)(AB)AC+BC
AABOC2B(AC)(BC)OCCOC2ABD(OC)(AB)AC+BC - Question 2 - Select One
OABC is a parallelogram such that OA=a,OB=bandOC=c, then the value →OA.→BA is
Aa2+3b2+c22Ba2−b2+3c22Ca2+3b2−c22D3a2+b2−c22 - Question 3 - Select One
When an object is placed between O and F1 in front of a convex lens, the image formed
Aenlarged and erectBdiminished and erectCreal and enlargedDdiminished and inverted
When an object is placed between O and F1 in front of a convex lens, the image formed
View SolutionA object is placed 20 cm from the convex lens, with focal length 40 cm. The image formed is virtual and erect.
What will be power of the lens?View SolutionWhen an object is placed between F and 2F in front of a convex lens, the image formed is
View SolutionAn object is placed at f/2 away from first focus of a convex lens where f is the focal length of the lens. Its image is formed at a distance 3f/2 in a slab of refractive index 3/2 , from the face of the slab facing the lens. Find the distance of this face of the slab from the second focus of the lens.
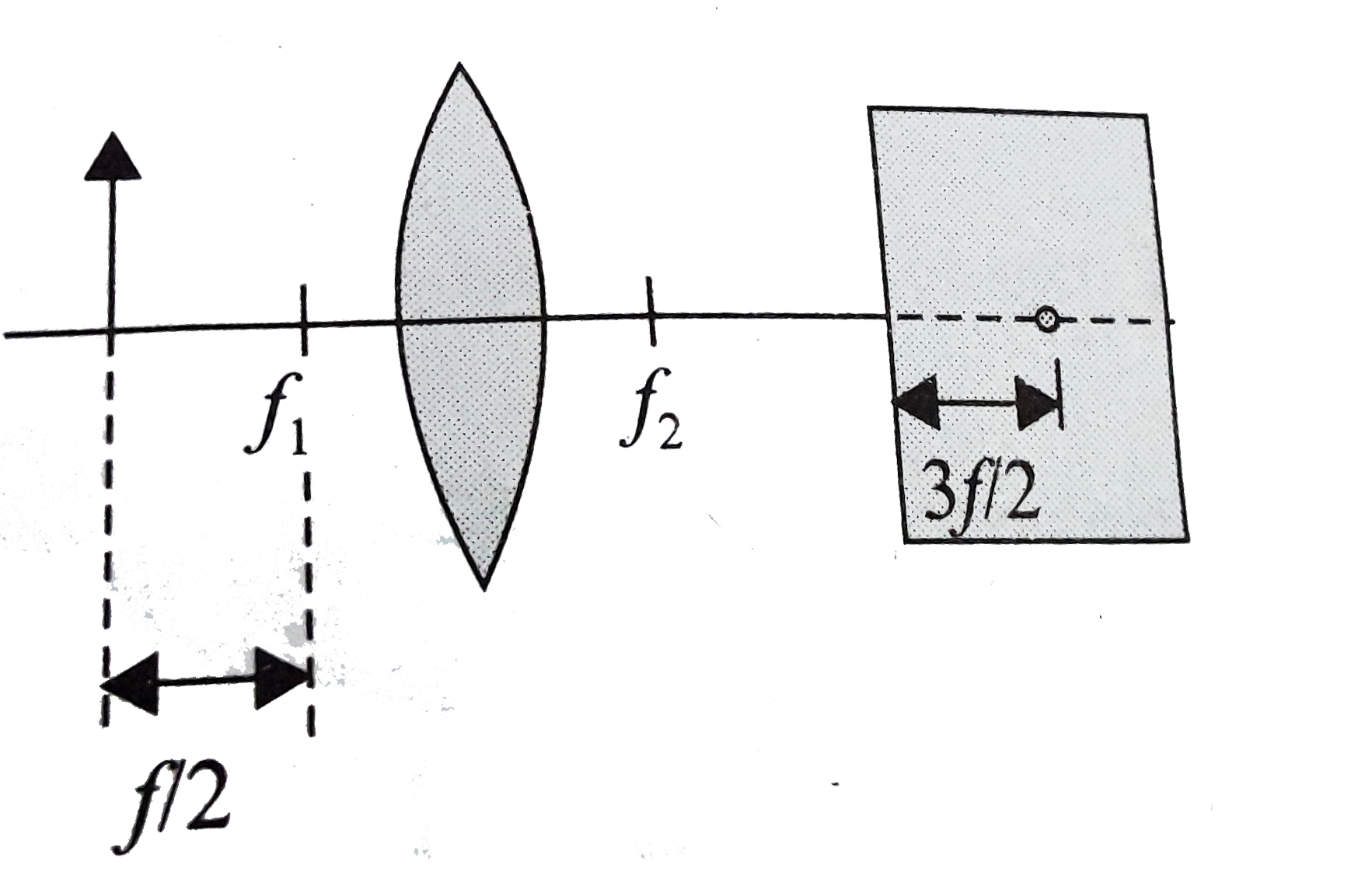 View Solution
View SolutionAn object is situated at a distance off/2from a convex lens of focal lengthfDistance of image will be-
View Solution

