A parallel sides glass slab of thickness 4 cm is made of a material of refractive index . When light is incident on one of the parallelfaces at an angle of , it emerges from the other parallel face. Find the lateral displacement of the emergent beam.
To find the lateral displacement of the emergent beam when light passes through a parallel-sided glass slab, we can follow these steps:
Step 1: Understand the Problem
We have a glass slab of thickness t=4cm and a refractive index μ=√3. Light is incident on one of the parallel faces at an angle of 60∘. We need to find the lateral displacement of the emergent beam.
Step 2: Use Snell's Law
According to Snell's Law:
μ1sini=μ2sinr
Here, μ1=1 (for air), μ2=√3, i=60∘, and r is the angle of refraction we need to find.
Step 3: Calculate the Angle of Refraction
Substituting the values into Snell's Law:
1⋅sin(60∘)=√3⋅sin(r)
sin(60∘)=√32
Thus,
√32=√3⋅sin(r)
Dividing both sides by √3:
12=sin(r)
Therefore,
r=sin−1(12)=30∘
Step 4: Calculate Lateral Displacement
The formula for lateral displacement D is given by:
D=tcosr⋅(sini−sinr)
Substituting the known values:
- t=4cm
- i=60∘
- r=30∘
Calculating cos(30∘):
cos(30∘)=√32
Now substituting into the formula:
D=4cos(30∘)⋅(sin(60∘)−sin(30∘))
Calculating sin(60∘) and sin(30∘):
- sin(60∘)=√32
- sin(30∘)=12
So,
D=4√32⋅(√32−12)
Calculating the difference:
√32−12=√3−12
Now substituting this back:
D=4⋅2√3⋅√3−12
Simplifying:
D=4(√3−1)√3
Calculating the final value:
D=4√3−4√3≈2.31cm
Final Answer
The lateral displacement of the emergent beam is approximately 2.31cm.
---
We have a glass slab of thickness t=4cm and a refractive index μ=√3. Light is incident on one of the parallel faces at an angle of 60∘. We need to find the lateral displacement of the emergent beam.
According to Snell's Law:
μ1sini=μ2sinr
Here, μ1=1 (for air), μ2=√3, i=60∘, and r is the angle of refraction we need to find.
Substituting the values into Snell's Law:
1⋅sin(60∘)=√3⋅sin(r)
sin(60∘)=√32
Thus,
√32=√3⋅sin(r)
Dividing both sides by √3:
12=sin(r)
Therefore,
r=sin−1(12)=30∘
The formula for lateral displacement D is given by:
D=tcosr⋅(sini−sinr)
Substituting the known values:
- t=4cm
- i=60∘
- r=30∘
cos(30∘)=√32
D=4cos(30∘)⋅(sin(60∘)−sin(30∘))
Calculating sin(60∘) and sin(30∘):
- sin(60∘)=√32
- sin(30∘)=12
D=4√32⋅(√32−12)
Calculating the difference:
√32−12=√3−12
D=4⋅2√3⋅√3−12
Simplifying:
D=4(√3−1)√3
Calculating the final value:
D=4√3−4√3≈2.31cm
The lateral displacement of the emergent beam is approximately 2.31cm.
Topper's Solved these Questions
- RAY OPTICS AND OPTICAL INSTRAUMENTS Book:NARAYNAChapter:RAY OPTICS AND OPTICAL INSTRAUMENTS Exercise:CUQ (REFLECTION)
Explore 9 Videos
- RAY OPTICS AND OPTICAL INSTRAUMENTS Book:NARAYNAChapter:RAY OPTICS AND OPTICAL INSTRAUMENTS Exercise:CUQ (REFRECTION)
Explore 28 Videos
- NUCLEI Book:NARAYNAChapter:NUCLEI Exercise:ASSERTION & REASON
Explore 5 Videos
- SEMI CONDUCTOR DEVICESBook:NARAYNAChapter:SEMI CONDUCTOR DEVICESExercise:Level-II (H.W)
Explore 36 Videos
Similar Questions
A ray of light incident normally on the first face of a glass prism of refractive index 1.6 just emerges from the other face. Find the angle of the prism.
View SolutionA ray of light is incident on one face of a prism (m=√3) at an angle of 60∘. The refracting angle of the prism is also 60∘. Find the angle of emergence .
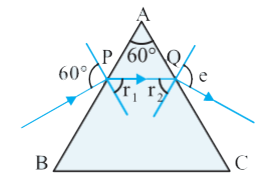
View Solution
Knowledge Check
A ray of light is incident on a parallel slab of thickness t and refractive index n. If the angle of incidence θ is small, than the lateral displacement in the incident and emergent ray will be
Atθ(n−1)nBtθnCtθnn−1Dtθ(n+1)nA ray of light is incident upon a parallel sided transparent slab of thickness 9 cm at an angle of incidence 60° • If the angle of refraction is 30°, the lateral displacement of the light ray is
A√3cmB3√3cmC3cmD2√3 cmA ray of light is incident at an angle of 60∘ on one face of a prism of angle 30∘. They ray emerges normally from the other face of the prism. The refractive index of the prism material is
A√2B√3C2D3
A right-angled isosceles glass prism is immersed in water of refractive index 4/3 .A ray of light falling on the prism at an angle of incidence of 60∘ is found to emerge from the other side at the same angle of60∘ .The refractive index of the material of the prism is: 1. √83 2. √38 3.√32 4. √43
View SolutionA glass slab made of a material of refractive index n1 is kept in a medium of refractive index n2.
A light ray is incident on the slab. Draw the path of the rays of light emerging from the glass slab, if n1=n2
View SolutionA glass slab made of a material of refractive index n1 is kept in a medium of refractive index n2.
A light ray is incident on the slab. Draw the path of the rays of light emerging from the glass slab, if n1<n2
View SolutionA glass slab made of a material of refractive index n1 is kept in a medium of refractive index n2.
A light ray is incident on the slab. Draw the path of the rays of light emerging from the glass slab, if n1>n2
View SolutionA ray of light is incident normally on one of the refracting surfaces of a prism of refracting angle A,. The emergent ray grazes the other refracting surface. Find the refractive index of the material ofprism.
View Solution
