Using three wires of resistances 1 ohm, 2 ohm and 3 ohm, then no.of different values of resistances that possible are
6
4
10
8
The correct Answer is:D
To solve the problem of finding the number of different resistance values possible using three wires with resistances of 1 ohm, 2 ohm, and 3 ohm, we can explore various combinations of these resistances in series and parallel configurations.
1. Understanding Series and Parallel Combinations:
- In a series combination, the total resistance Rs is the sum of the individual resistances:
Rs=R1+R2+R3
- In a parallel combination, the total resistance Rp is given by:
1Rp=1R1+1R2+1R3
2. Calculating All Possible Combinations:
- We can use the three resistors in different combinations:
- All three in series
- All three in parallel
- Any two in series and one in parallel
- Any two in parallel and one in series
3. Calculating Individual Combinations:
- All in Series:
Rseries=1+2+3=6 ohms
- All in Parallel:
1Rparallel=11+12+13=1+0.5+0.333=1.833⟹Rparallel≈0.545 ohms
4. Two in Series, One in Parallel:
- (1 ohm and 2 ohm in series, 3 ohm in parallel):
Rs1=1+2=3 ohms
1Rp1=13+13⟹Rp1=32=1.5 ohms
- (1 ohm and 3 ohm in series, 2 ohm in parallel):
Rs2=1+3=4 ohms
1Rp2=14+12⟹Rp2=43≈1.333 ohms
- (2 ohm and 3 ohm in series, 1 ohm in parallel):
Rs3=2+3=5 ohms
1Rp3=15+12⟹Rp3=107≈1.428 ohms
5. Two in Parallel, One in Series:
- (1 ohm and 2 ohm in parallel, 3 ohm in series):
1Rp4=11+12⟹Rp4=23≈0.667 ohms
Rtotal=Rp4+3=3.667 ohms
- (1 ohm and 3 ohm in parallel, 2 ohm in series):
1Rp5=11+13⟹Rp5=34=0.75 ohms
Rtotal=Rp5+2=2.75 ohms
- (2 ohm and 3 ohm in parallel, 1 ohm in series):
1Rp6=12+13⟹Rp6=65=1.2 ohms
Rtotal=Rp6+1=2.2 ohms
6. Listing All Unique Resistance Values:
- From the calculations, we have the following unique resistance values:
- 6 ohms (all in series)
- 0.545 ohms (all in parallel)
- 1.5 ohms (1 & 2 in series, 3 in parallel)
- 1.333 ohms (1 & 3 in series, 2 in parallel)
- 1.428 ohms (2 & 3 in series, 1 in parallel)
- 3.667 ohms (1 & 2 in parallel, 3 in series)
- 2.75 ohms (1 & 3 in parallel, 2 in series)
- 2.2 ohms (2 & 3 in parallel, 1 in series)
7. Counting Unique Values:
- The unique resistance values are: 0.545, 1.2, 1.333, 1.428, 1.5, 2.2, 2.75, 3.667, and 6.
- Total unique values = 9.
Final Answer:
The total number of different resistance values possible using the three wires is 9.
- In a series combination, the total resistance Rs is the sum of the individual resistances:
Rs=R1+R2+R3
- In a parallel combination, the total resistance Rp is given by:
1Rp=1R1+1R2+1R3
- We can use the three resistors in different combinations:
- All three in series
- All three in parallel
- Any two in series and one in parallel
- Any two in parallel and one in series
- All in Series:
Rseries=1+2+3=6 ohms
- All in Parallel:
1Rparallel=11+12+13=1+0.5+0.333=1.833⟹Rparallel≈0.545 ohms
- (1 ohm and 2 ohm in series, 3 ohm in parallel):
Rs1=1+2=3 ohms
1Rp1=13+13⟹Rp1=32=1.5 ohms
- (1 ohm and 3 ohm in series, 2 ohm in parallel):
Rs2=1+3=4 ohms
1Rp2=14+12⟹Rp2=43≈1.333 ohms
- (2 ohm and 3 ohm in series, 1 ohm in parallel):
Rs3=2+3=5 ohms
1Rp3=15+12⟹Rp3=107≈1.428 ohms
- (1 ohm and 2 ohm in parallel, 3 ohm in series):
1Rp4=11+12⟹Rp4=23≈0.667 ohms
Rtotal=Rp4+3=3.667 ohms
- (1 ohm and 3 ohm in parallel, 2 ohm in series):
1Rp5=11+13⟹Rp5=34=0.75 ohms
Rtotal=Rp5+2=2.75 ohms
- (2 ohm and 3 ohm in parallel, 1 ohm in series):
1Rp6=12+13⟹Rp6=65=1.2 ohms
Rtotal=Rp6+1=2.2 ohms
- From the calculations, we have the following unique resistance values:
- 6 ohms (all in series)
- 0.545 ohms (all in parallel)
- 1.5 ohms (1 & 2 in series, 3 in parallel)
- 1.333 ohms (1 & 3 in series, 2 in parallel)
- 1.428 ohms (2 & 3 in series, 1 in parallel)
- 3.667 ohms (1 & 2 in parallel, 3 in series)
- 2.75 ohms (1 & 3 in parallel, 2 in series)
- 2.2 ohms (2 & 3 in parallel, 1 in series)
- The unique resistance values are: 0.545, 1.2, 1.333, 1.428, 1.5, 2.2, 2.75, 3.667, and 6.
- Total unique values = 9.
The total number of different resistance values possible using the three wires is 9.
Similar Questions
How can three resistances of 2, 3 and 6 ohms be connected so as to give a total resistance of 1 ohm? Justify your answer.
View SolutionTwo batteries one of the emf 3V, internal resistance 1 ohm and the other of emf 15 V , internal resistance 2 ohm are connected in series with a resistance R as shown . If the potential difference between a and b is zero the resistance of R in ohm is
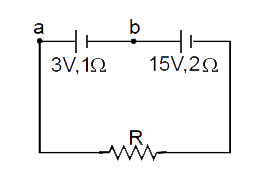
View Solution
Knowledge Check
Using three wires of resistances 1 ohm, 2ohm and 3 ohm then no. of different values of resistances that possible are
A6B4C10D8Two resistance wires on joining in parallel the resultant resistance is ohms 65 ohms. One of the wire breaks, the effective resistance is 2 ohms . The resistance of the broken wire is
A35 ohmB2 ohmC65 ohmD3 ohmA 10 m long wire of resistance 15 ohm is connectd in series with a battery of emf 2V (no internal resistance) and a resistance of 5 ohm. The potential gradient along the wire is
A0.15Vm−1B0.45Vm−1C1.5Vm−1D4.5Vm−1
If a current of 3 A flows through a wire of resistance 8 ohms, calculate the potential difference applied across its ends.
View SolutionA student has been given 3 resistances of 3 ohms each.
(a) How should he connect these resistances so as to obtain a resultant resistance of 1 ohm ?
(b) Draw a labelled diagram to show the combination of these resistances.
View SolutionHow should the two resistances of 2 ohms each be connected so as to produce an equivalent resistance of 1 ohm?
View SolutionA silver voltameter of resistance 2 ohm and a 3 ohm resistor are connected in series across a cell. If a resistance of 2 ohm is connected in parallel with the voltameter, then the rate of deposition of silver
View SolutionThe resistance of the four arms P,Q,R and S in a Wheatstone's bridge are 10ohm30ohm and 90ohm rerspectively. The e.m.f. and internal resistance of the cell are 7vo< and 5ohm respectively. If the galvanometer resistance is 50ohm, the current drawn for the cell will be
View Solution
