In case of a dipole field
intensity can be zero
potential can be zero
both can be zero
none of these
The correct Answer is:B
To solve the problem of finding the speed of each proton when they reach infinity due to their mutual repulsion, we can follow these steps:
1. Understand the System: We have two protons, each with charge q (where q=e=1.6×10−19C), separated by a distance r. They repel each other due to their like charges.
2. Calculate the Electrostatic Force: The electrostatic force F between the two protons can be calculated using Coulomb's law:
F=k⋅q1⋅q2r2=k⋅q2r2
where k is Coulomb's constant, k=14πϵ0.
3. Calculate the Initial Potential Energy: The potential energy U of the system when the protons are separated by a distance r is given by:
U=k⋅q2r
4. Energy Conservation Principle: As the protons move apart due to their mutual repulsion, the potential energy converts into kinetic energy. At infinity, the potential energy approaches zero, and all the initial potential energy becomes kinetic energy.
5. Kinetic Energy of Each Proton: Since both protons will have the same kinetic energy K when they reach infinity, we can express this as:
K=12mv2
where m is the mass of a proton and v is the speed of each proton.
6. Set Initial Potential Energy Equal to Total Kinetic Energy: At the initial position, all potential energy will convert into kinetic energy:
k⋅q2r=2⋅12mv2
Simplifying gives:
k⋅q2r=mv2
7. Solve for Speed v: Rearranging the equation to solve for v:
v2=k⋅q2m⋅r
v=√k⋅q2m⋅r
8. Substituting k: Substitute k=14πϵ0:
v=
⎷14πϵ0⋅q2m⋅r
9. Final Expression: Therefore, the speed of each proton when they reach infinity is:
v=√q24πϵ0mr
F=k⋅q1⋅q2r2=k⋅q2r2
where k is Coulomb's constant, k=14πϵ0.
U=k⋅q2r
K=12mv2
where m is the mass of a proton and v is the speed of each proton.
k⋅q2r=2⋅12mv2
Simplifying gives:
k⋅q2r=mv2
v2=k⋅q2m⋅r
v=√k⋅q2m⋅r
v=
⎷14πϵ0⋅q2m⋅r
v=√q24πϵ0mr
Topper's Solved these Questions
- ELECTROSTATIC POTENTIAL AND CAPACITANCEBook:NARAYNAChapter:ELECTROSTATIC POTENTIAL AND CAPACITANCEExercise:C.U.Q (Potential energy of system of charges )
Explore 6 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITANCEBook:NARAYNAChapter:ELECTROSTATIC POTENTIAL AND CAPACITANCEExercise:C.U.Q (Potential energy of dipole)
Explore 3 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITANCEBook:NARAYNAChapter:ELECTROSTATIC POTENTIAL AND CAPACITANCEExercise:Evaluate yourself-10
Explore 1 Video
- ELECTROMAGNETIC WAVES Book:NARAYNAChapter:ELECTROMAGNETIC WAVES Exercise:EXERCISE -4
Explore 15 Videos
- ELECTROSTATICS AND GAUSS LAWBook:NARAYNAChapter:ELECTROSTATICS AND GAUSS LAWExercise:Intergers type question
Explore 11 Videos
Similar Questions
Knowledge Check
In case of dipole-induced dipole forces, interaction energy is proportional to
A1r6,wherer=distancebetweentwomo≤ce–sB1r3,wherer=distancebetweentwomo≤ce–sC1r4,wherer=distancebetweentwomo≤ce–sD1r,wherer=distancebetweentwomo≤ce–sAssertion :- Ion-induced dipole interaction is stronger than dipole-induced dipole interaction. Reason :- Accumulated charges on the molecule in case of ion-induced dipole is more compared to that in case of dipole interaction.
AIf both Assertion & Reason are True & the Reason is a correct explanation of the Assertion.BIf both Assertion & Reason are True but Reason is not a correct explanation of the Assertion.CIf Assertion is True but the Reason is False.DIf both Assertion & Reason are False.Maximum torque on a dipole in electric field is given by:
ApEBPECEPD→P.→E
Potential Energy OF electric dipole||Force on a dipole in Non-uniform electric field||SHM in case OF dipole
View SolutionA dipole is placed in the field of a point charge, the distance between the dipole and the field source being much greater than the dipole separation. Find the force acting on the dipole and the torque, if the dipole is arranged:
In the direction of the line of force.
View Solution(i) State Maxwell's right-hand thumb rule.
(ii) PQ is a current carrying conductor in the plane of the paper as shown in the figure. Mention the direction of magnetic fields produced by it at points A and B. Given r1<r2, where will the strength of the magnetic field be larger?
Justify your answer in each case.
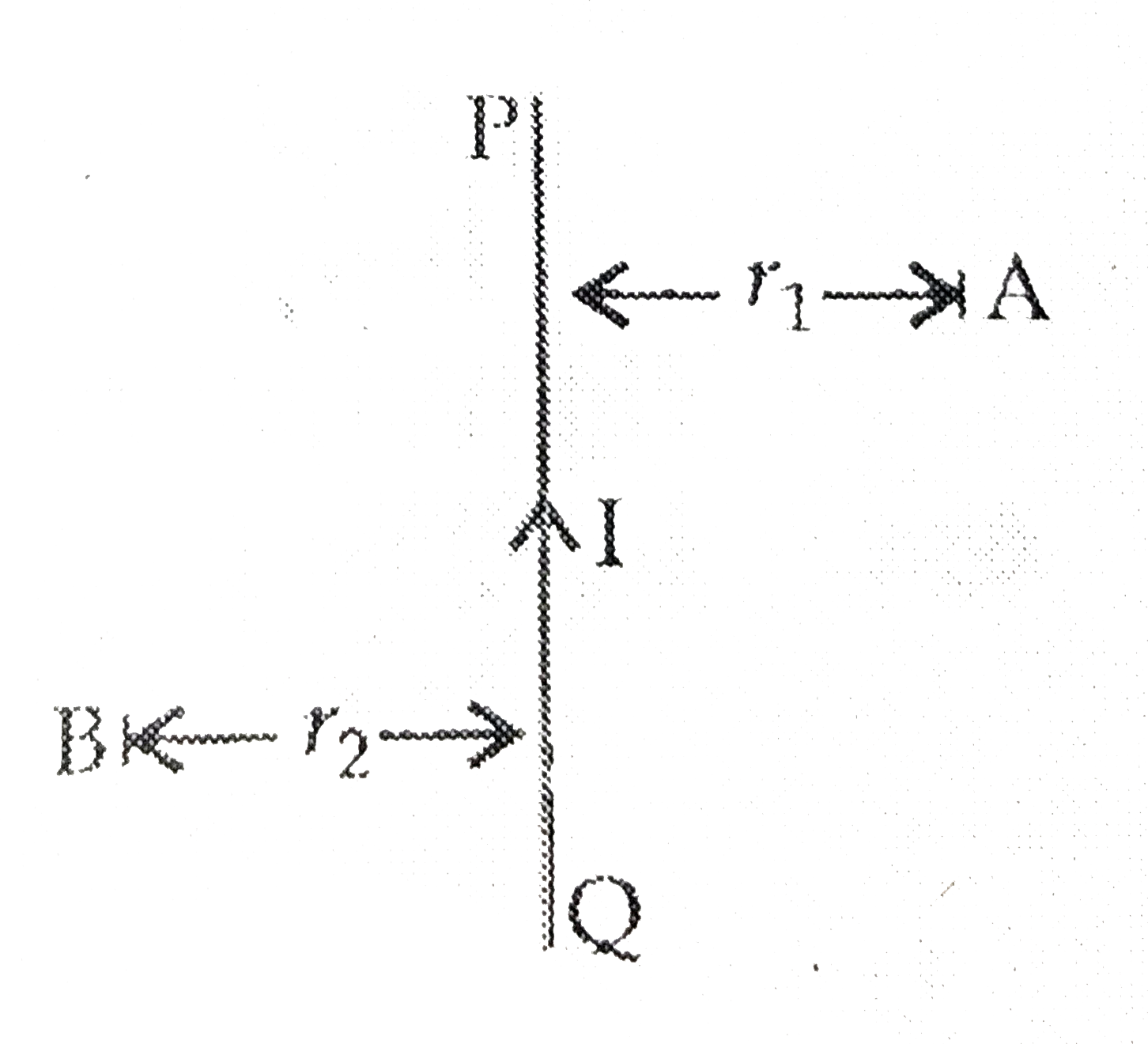
View SolutionCorrect plot of dτ/dθ.θ vs θ in case of an electric dipole placed in any uniform electric field is
View SolutionIn which of the following cases dipole moment will be highest ?
View Solution