A uniform rope of mass (m) and length (L) placed on frictionless horizontal ground is being pulled by two forces at its ends as shown in the figure. As a result, the rope accelerates toward the right.

Tension (T) at the mid point of the pore is
The correct Answer is:D
Step by step video, text & image solution for A uniform rope of mass (m) and length (L) placed on frictionless horizontal ground is being pulled by two forces F_(A) and F_(B) at its ends as shown in the figure. As a result, the rope accelerates toward the right. Tension (T) at the mid point of the pore is by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- NEWTON'S LAWS OF MOTION & FRICTIONBOOK - ALLENCHAPTER - NEWTON'S LAWS OF MOTION & FRICTIONEXERCISE - EXERCISE (O-2)
31 Videos
- NEWTON'S LAWS OF MOTION & FRICTIONBOOK - ALLENCHAPTER - NEWTON'S LAWS OF MOTION & FRICTIONEXERCISE - EXERCISE (JM)
6 Videos
- NEWTON'S LAWS OF MOTION & FRICTIONBOOK - ALLENCHAPTER - NEWTON'S LAWS OF MOTION & FRICTIONEXERCISE - EXERCISE (S-2)
9 Videos
- MOTION IN A PALNEBOOK - ALLENCHAPTER - MOTION IN A PALNEEXERCISE - SOLVED EXAMPLE
28 Videos
- NEWTONS LAWS OF MOTIONBOOK - ALLENCHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - EXERCISE-III
28 Videos
Similar Questions
A uniform rope of length L , resting on frictionless horizontal table is pulled at one end by a force F . What is the tension in the rope at a distance x from the end where the force is applied ?
[ Hint : Consider the motion of the entire rope and the motion of x length of rope using P = ma formula and third law of motion ]
View SolutionA block of mass M is being pulled with the help of a string of mass m and length L. the horizontal force applied by the man on the string is F.

Determine
(a) Find the force exerted by the string on the block and acceleration of system.
(b) Find the tension at the mid point of the string.
(c ) Find the tension at a distance x from the end at which force is applied.
Assume that the block is kept on a frictionless horizontal surface and the mass is uniformly distributed in the string.
View Solution
Knowledge Check
A uniform rope of mass (m) and length (L) placed on frictionless horizontal ground is being pulled by two forces FAandFB at its ends as shown in the figure. As a result, the rope accelerates toward the right.

Acceleration (A) of the rope isAzeroBa=FA+FBmCa=FA−FBmDa=FB−FAmA uniform rod of mass M and length L lies flat on a frictionless horizantal surface. Two forces F and 2F are applied along the length of the rod as shown. The tension in the rod at point P is
 AFB3FC5F4D7F4
AFB3FC5F4D7F4A rod of length L and mass M is acted on by two unequal forces F1 and F2(<F)as shown in the figure.
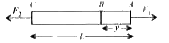
The tension in the rod at a distance y from the end A is given byAF1(1−yL)+F2(yL)BF2(1−yL)+F1(YL)C(F1−F2)yLDNone of these
A block of mass M is attached to a uniform rope of mass m. This arrangement is pulled by applying a force F at the rope as shown. Find the tension in the rope (a) at the junction of the rope and the block (b) at the mid point of the rope.
View SolutionAs shown in Fig. three masses m, 3m and 5m connected together lie on a frictionless horizontal surface and pulled to the left by a force F. The tension T1 in the first string is 24 N. Find (i) acceleration of the system, (ii) tension in the second string, and (iii) force F.
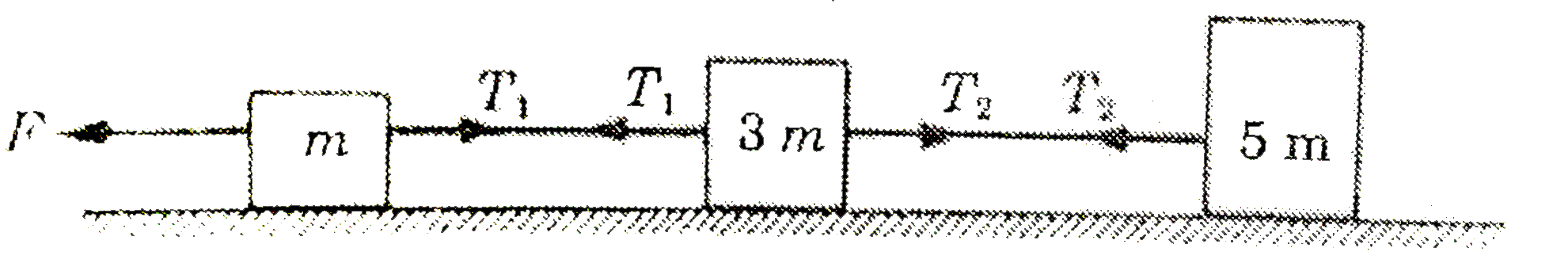
View SolutionA block of mass 10kg lying on a smooth horizontal surface is being pulled by means of a rope of mass 2kg .If a force of 36N is applied at the end of the rope,the tension at the midpoint of the rope is?
View SolutionA uniform rope of length 10m is on a smooth horizontal surface. It is being pulled by a horizontal force of 20N at one end. The ratio of tension at a distance of 4m from force end to tension at a distance of 4m from free end is
View SolutionTwo blocks of masses m and M are placed on a horizontal frictionless table connected by light spring as shown in the figure. Mass M is pulled to the right with a force F. If the acceleration of mass m is a, then the acceleration of mass M will be

View Solution
