A 40 kg boy climbs a rope that passes over an ideal pulley. The other end of the rope is attached to a 60 kg weight placed on the ground. What is the maximum upward acceleration the boy can have without lifting the weight ? If he climbs the rope with upward acceleration 2 g, with what acceleration the weight will rise up ?
The correct Answer is:0.5g,g
To solve the problem step-by-step, we will analyze the forces acting on both the boy and the weight, and use Newton's second law of motion.
Step 1: Identify the forces acting on the boy and the weight
- The weight of the boy (W_boy) = mass × gravity = 40 kg × g = 40g.
- The weight of the block (W_block) = mass × gravity = 60 kg × g = 60g.
- The tension in the rope (T) acts upwards on the boy and downwards on the block.
Step 2: Determine the maximum upward acceleration of the boy without lifting the weight
For the weight not to lift off the ground, the tension in the rope must not exceed the weight of the block. Therefore, we set up the equation:
T≤60g
For the boy climbing with an upward acceleration (a), the net force acting on him can be expressed as:
T−40g=40a
From this, we can express the tension (T):
T=40g+40a
Step 3: Set the maximum tension equal to the weight of the block
To find the maximum upward acceleration (a_max) of the boy without lifting the weight, we substitute T from the previous equation into the maximum tension condition:
40g+40amax≤60g
Step 4: Solve for a_max
Rearranging the equation gives:
40amax≤60g−40g
40amax≤20g
amax≤20g40
amax≤g2
Thus, the maximum upward acceleration of the boy without lifting the weight is:
amax=g2
Step 5: Analyze the scenario when the boy climbs with an upward acceleration of 2g
If the boy climbs with an upward acceleration of 2g, we substitute this value into the tension equation:
T=40g+40(2g)=40g+80g=120g
Step 6: Determine the acceleration of the weight
Now, we analyze the forces acting on the weight:
The net force acting on the weight is given by:
T−60g=60a
Substituting the value of T:
120g−60g=60a
60g=60a
a=g
Thus, when the boy climbs with an upward acceleration of 2g, the weight will rise with an acceleration of:
a=g
Summary of Results
1. The maximum upward acceleration the boy can have without lifting the weight is g2.
2. If the boy climbs the rope with an upward acceleration of 2g, the weight will rise with an acceleration of g.
---
- The weight of the boy (W_boy) = mass × gravity = 40 kg × g = 40g.
- The weight of the block (W_block) = mass × gravity = 60 kg × g = 60g.
- The tension in the rope (T) acts upwards on the boy and downwards on the block.
For the weight not to lift off the ground, the tension in the rope must not exceed the weight of the block. Therefore, we set up the equation:
To find the maximum upward acceleration (a_max) of the boy without lifting the weight, we substitute T from the previous equation into the maximum tension condition:
Rearranging the equation gives:
40amax≤20g
amax≤20g40
amax≤g2
If the boy climbs with an upward acceleration of 2g, we substitute this value into the tension equation:
Now, we analyze the forces acting on the weight:
60g=60a
a=g
1. The maximum upward acceleration the boy can have without lifting the weight is g2.
2. If the boy climbs the rope with an upward acceleration of 2g, the weight will rise with an acceleration of g.
Topper's Solved these Questions
- NEWTON'S LAWS OF MOTION & FRICTIONBOOK - ALLENCHAPTER - NEWTON'S LAWS OF MOTION & FRICTIONEXERCISE - EXERCISE (S-2)
9 Videos
- NEWTON'S LAWS OF MOTION & FRICTIONBOOK - ALLENCHAPTER - NEWTON'S LAWS OF MOTION & FRICTIONEXERCISE - EXERCISE (O-1)
51 Videos
- NEWTON'S LAWS OF MOTION & FRICTIONBOOK - ALLENCHAPTER - NEWTON'S LAWS OF MOTION & FRICTIONEXERCISE - EXERCISE (JA)
4 Videos
- MOTION IN A PALNEBOOK - ALLENCHAPTER - MOTION IN A PALNEEXERCISE - SOLVED EXAMPLE
28 Videos
- NEWTONS LAWS OF MOTIONBOOK - ALLENCHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - EXERCISE-III
28 Videos
Similar Questions
A boy of mass 40kg climbs up a rope with an acceleration of 1.2ms−2.What is the tension in the rope?
View SolutionA light rope is passed over a pulley such that at its one end a block is attached , and on the other end a boy is climbing up with acceleration g2 relative to rope. Mass of the block is 30 kg and that of the boy is 40 kg. Find the tension and acceleration of the rope .
View Solution
Knowledge Check
A monkey of mass m clings a rope to a slung over a fixed pulley .The opposite end of the rope is tried to a weight of mass M tying on a horizontal table is μ Find the acceleration of weight and the tension of the rope for two cases .The monkey move downward with respect to the rope with an acceleration b.
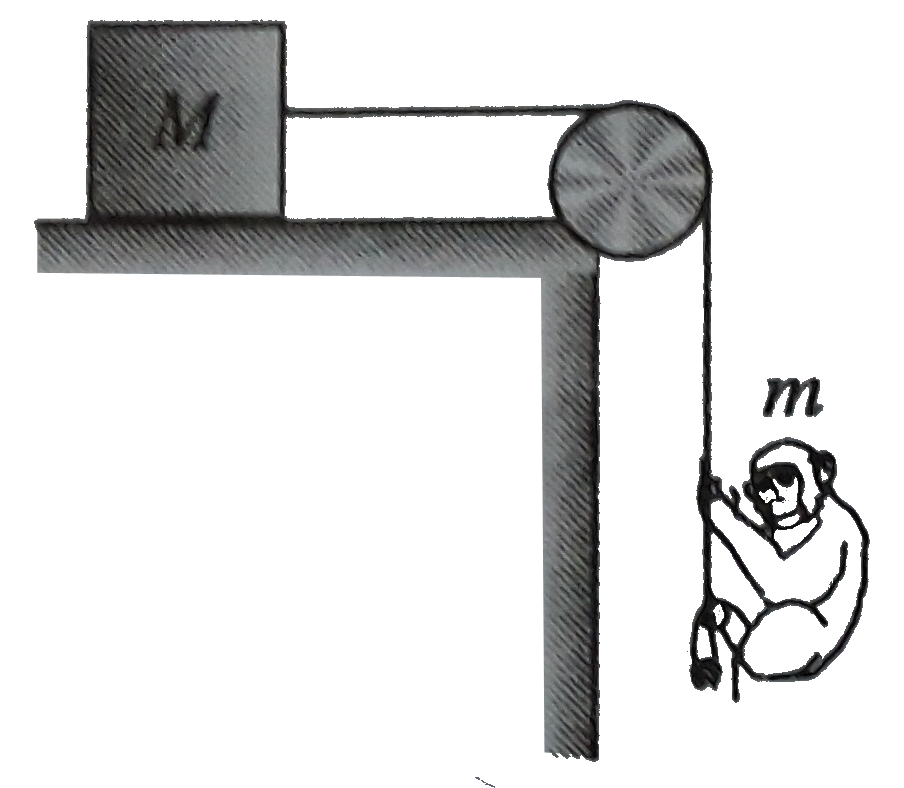
The acceleration of weight isA2m(g+b)−μMgM+2mBm(g+b)−μMg2(M+m)Cm(g+b)−3μMgM+3mDm(g+b)−μMgM+2mA monkey of mass m clings a rope to a slung over a fixed pulley .The opposite end of the rope is tried to a weight of mass M tying on a horizontal table is μ Find the acceleration of weight and the tension of the rope for two cases .The monkey move downward with respect to the rope with an acceleration b.
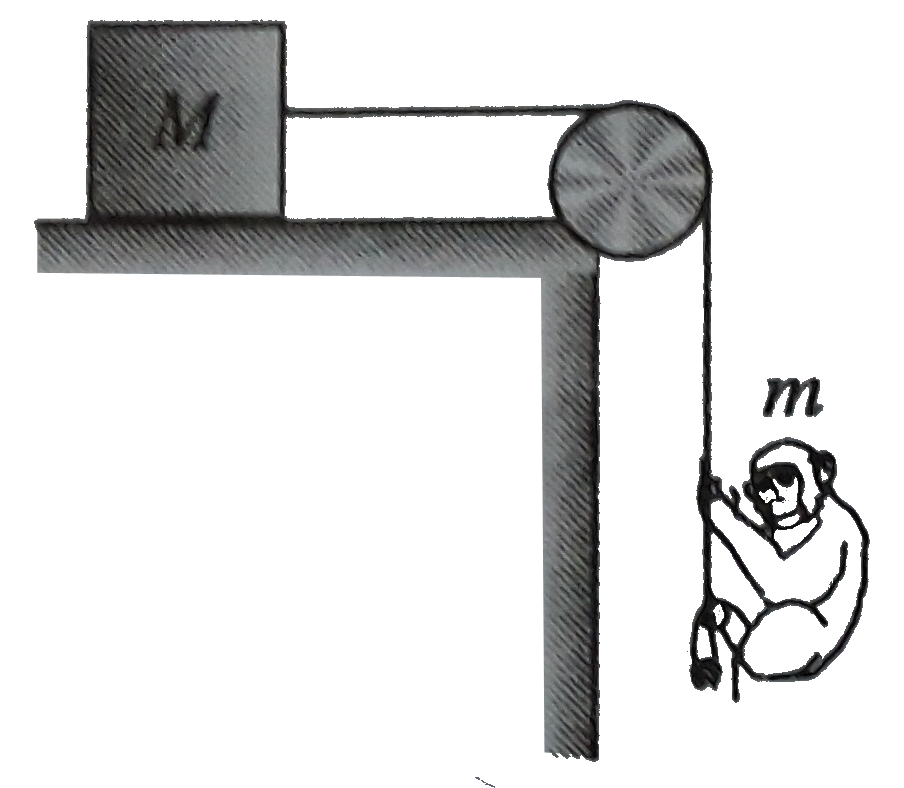
The tension of rope isAMm(μg+g+b)M+mBMm(μg−g+b)M+mCMm(μg+g−b)M+mDMm(μg−g−b)M+mA cat of mass m = 1 kg climbs to a rope hung over a leight frictionless pulley. The opposite end of the rope is tied to a weight of mass M = 2m lying on a smooth horizontal plane. What is the tension of the rope where the cat move upwards with in acceleration a=2m/s2 relative to the rope ?
 A2 NB12NC8ND4N
A2 NB12NC8ND4N
A 10 kg monkey climbs up a massless rope that runs over a frictionless tree limb and back down to a 15 kg package on the ground (Fig. 5-56). (a) What is the magnitude of the least acceleration the monkey must have if it is to lift the package off the ground? If, after the package has been lifted, the monkey stops its climb and holds on to the rope, what are the (b) magnitude and (c) direction of the monkey's acceleratiohn and (d) the tension in the rope ?

View SolutionA monkey of mass m climbs up to a rope hung over a fixed pulley. The opposite end of the rope is tied to a weight of mass M lying on a horizontal plane. Neglecting the friction, find the acceleration of both the bodies (relative to the plane) and the tension of the rope for the three cases:
(i) The monkey does not move with respect to the rope
(ii) The monkey moves upwards with respect to the rope with acceleration a.
(iii) The monkey moves downwards with respect to the rope, with acceleration a.
View SolutionA man of mass m stands on a frame of mass M. He pulls on a light rope, which passes over a pulley. The other end of the rope is attached to the frame. For the system to be in equilibrium, what force must the man exert on the rope?
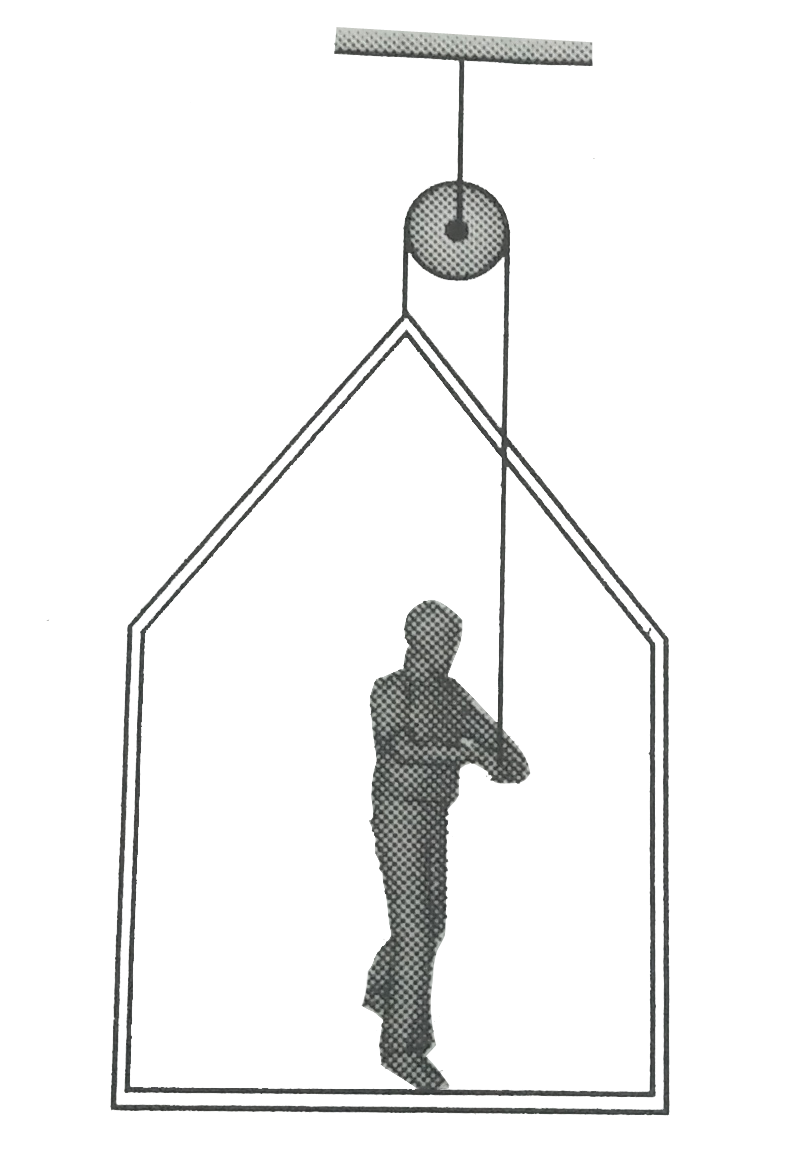
View SolutionA body of mass 40 kg wants to climb up a rope hanging vertically. The rope can withstands a maximum tension of 500 N. What is the maximum acceleration with which the boy can climb rope? Tak e g=10m/s2
View SolutionA monkey of 25 kg is holding a vertical rope. The rope does not break if a body of mass 30 kg is suspended with the rope exceeds 30 kg. What will be the maximum acceleration with which the monkey can climb up along the rope ? ( Take ,=10ms−2)
View Solution
