A glass hemispher of refractive index 4/3 and of radius 4cm is placed on a plane mirror. A point object is placed on axis of this sphere at a distance 'd' from O as shown in the figure. If the final image is formed at infinity, then find the value of d in mm .
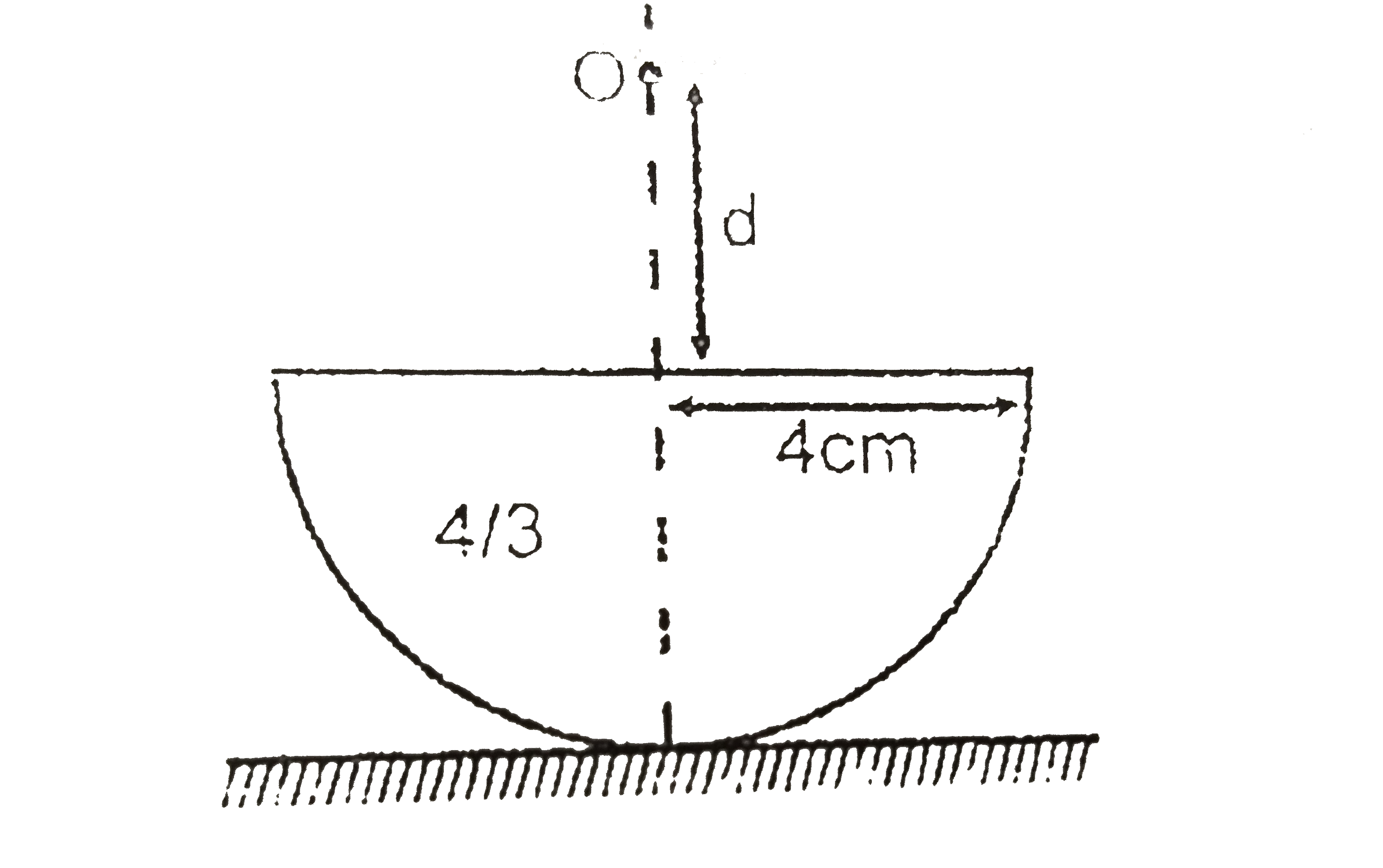
The correct Answer is:30
The image of object O by refraction at plane surface is formed at I such that
AI=43d
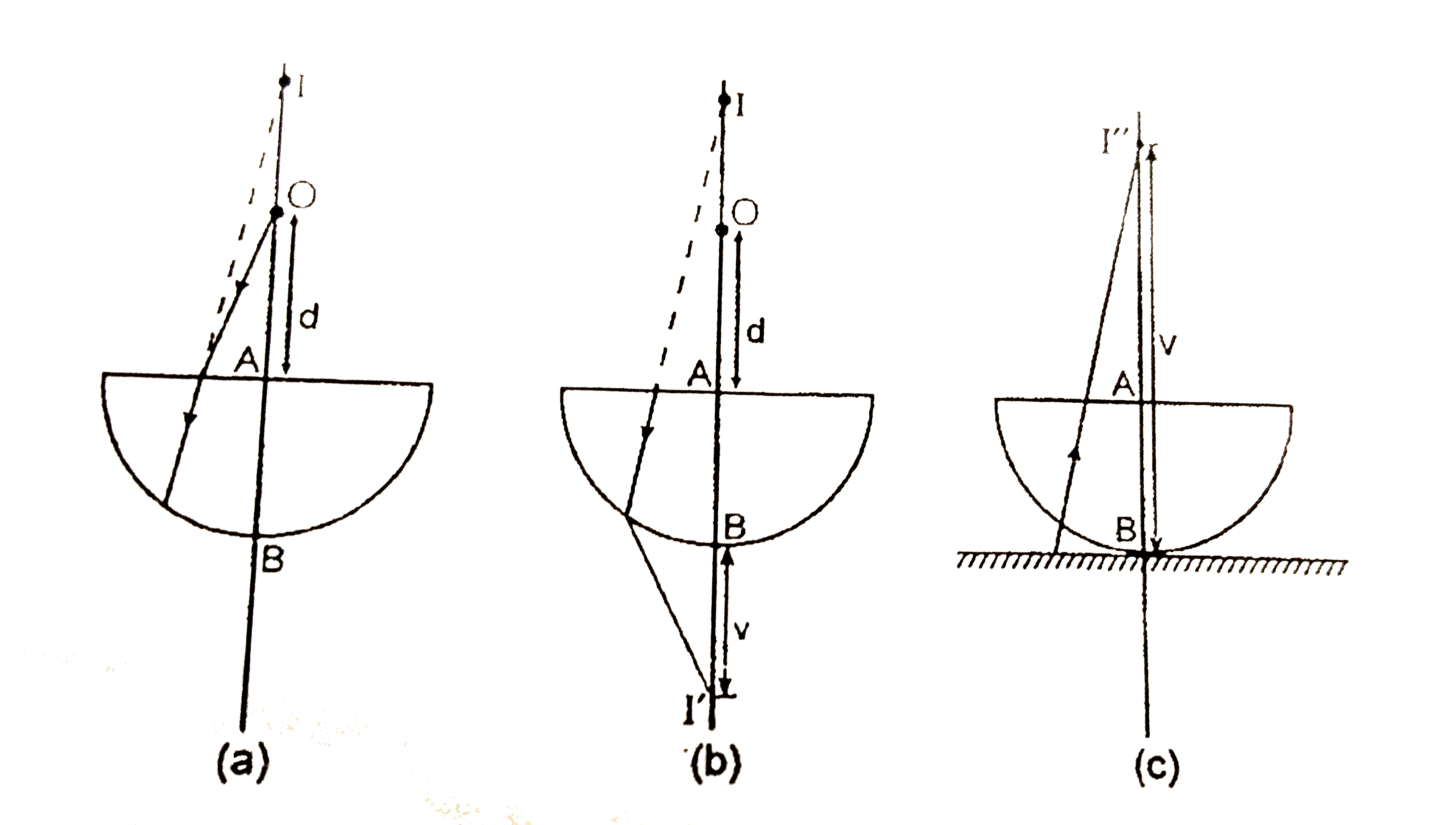
I acts object for curved surved. The curved surface makes image of I at I
1v−43−(R+43d)=1−43−Ror1v=13R−43R+4d
I' acts as object for mirror. Mirror makes its image at I" distance v above B .
I" acts as virtual object for the curved surface which makes its image at infinity
43∞−[13R−43R+4d]=13R
Solving we get d=34R34×4=3cm
Topper's Solved these Questions
- GEOMATRICAL OPTICS Book:RESONANCEChapter:GEOMATRICAL OPTICS Exercise:Exercise-3
Explore 79 Videos
- GEOMATRICAL OPTICS Book:RESONANCEChapter:GEOMATRICAL OPTICS Exercise:Advance level Problems
Explore 35 Videos
- GEOMATRICAL OPTICS Book:RESONANCEChapter:GEOMATRICAL OPTICS Exercise:Exercise-1
Explore 142 Videos
- EXPERIMENTAL PHYSICSBook:RESONANCEChapter:EXPERIMENTAL PHYSICSExercise:PART -II
Explore 10 Videos
- GRAVITATIONBook:RESONANCEChapter:GRAVITATIONExercise:HIGH LEVEL PROBLEMS
Explore 16 Videos
Similar Questions
- A glass sphere of refractive index μ 2.4 has one half silvered. A point object O is placed in front of the sphere at a distance d as shown in the figure. The value of d for which image of O is coinciding with the object O itself is given by d = kR then value of K will be? Consider paraxial rays approximation to be valid.
View SolutionTwo concave mirrors each of focal length 'f' are placed infront of each other co=axially at a distance of 4d in a medium of refractive index n0. A plane galss slab of refractive index 'n'& thickness 'd' is placed at a distance of 'd' from M1.A point object O is placed at a distace of 'd' from M2 as shown in the figure. Consider first reflection by M2, then refraction on slab and then reflection by M1. Determine the distance of this iamge after reflection from M1
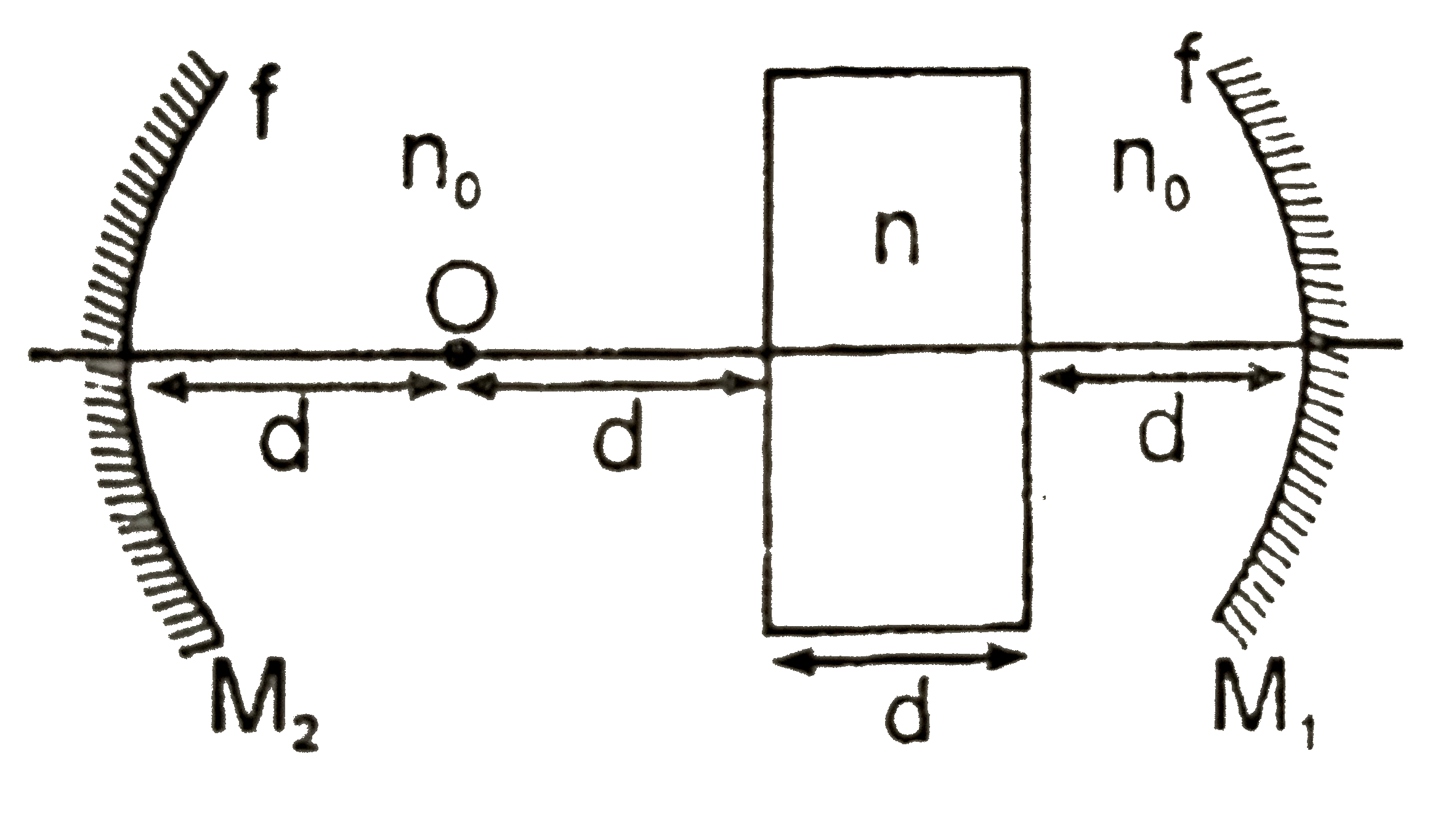
View Solution
Knowledge Check
A point is placed in front of thick plane mirror as shown in figure. Find the location of final image w.r.t. object.
 A15/2cmB15cmC40/3cmD80/3cm
A15/2cmB15cmC40/3cmD80/3cmFind the distance of object placed in the slab of refractive index μ from point P of the curved surface of radius R so that image is formed at infinity:
 A(μ−1)RμBμR(μ−1)CR(μ−1)D(μ−1)R2μ
A(μ−1)RμBμR(μ−1)CR(μ−1)D(μ−1)R2μAn object (O) is placed between two parallel plane mirror as shown in figure. Distance between the 4th image is
 A16 mB32 mC8 mD64 m
A16 mB32 mC8 mD64 m
A thin biconvex lens of refractive index 3/2 and radius of curvature 50cm is placed on a reflecting convex surface of radius of curvature 100cm. A point object is placed on the principal axis of the system such that its final image coincides with itself. Now, few drops of a transparent liquid is placed between the mirror and lens such that final image of the object is at infinity. Find refractive index of the liquid used. Also, find the position of the object.
View SolutionA thin equiconvex lens of refractive index 3/2 and radius of curvature 50cm is placed on a reflecting convex surface of radius of curvature 100cm. A point object is placed on the principle axis of the system such that its final image coincides with itself. Now few drops of a transparent liquid are placed between the mirror and lens such that final image of the object is at infinity. Find (a) the refractive index of the liquid used and (b the position of the object.
View SolutionA double convex spherical glass has refractive index 1.5 and both radius of curvature 30 cm. A point object is placed at a distance of 120 cm from the spherical glass. What will be the position of the image formed ?
View SolutionAn object is placed 50cm from the surface of a glass sphere of radius 10cm along the diameter. Where will the final image be formed after refraction at both the surfaces ? μ of glass =1.5.
View SolutionA concave mirror of focal length 2cm is placed on a glass slab as shown in the figure. The image of point object O formed due to reflection at mirror and then refraction by the slab
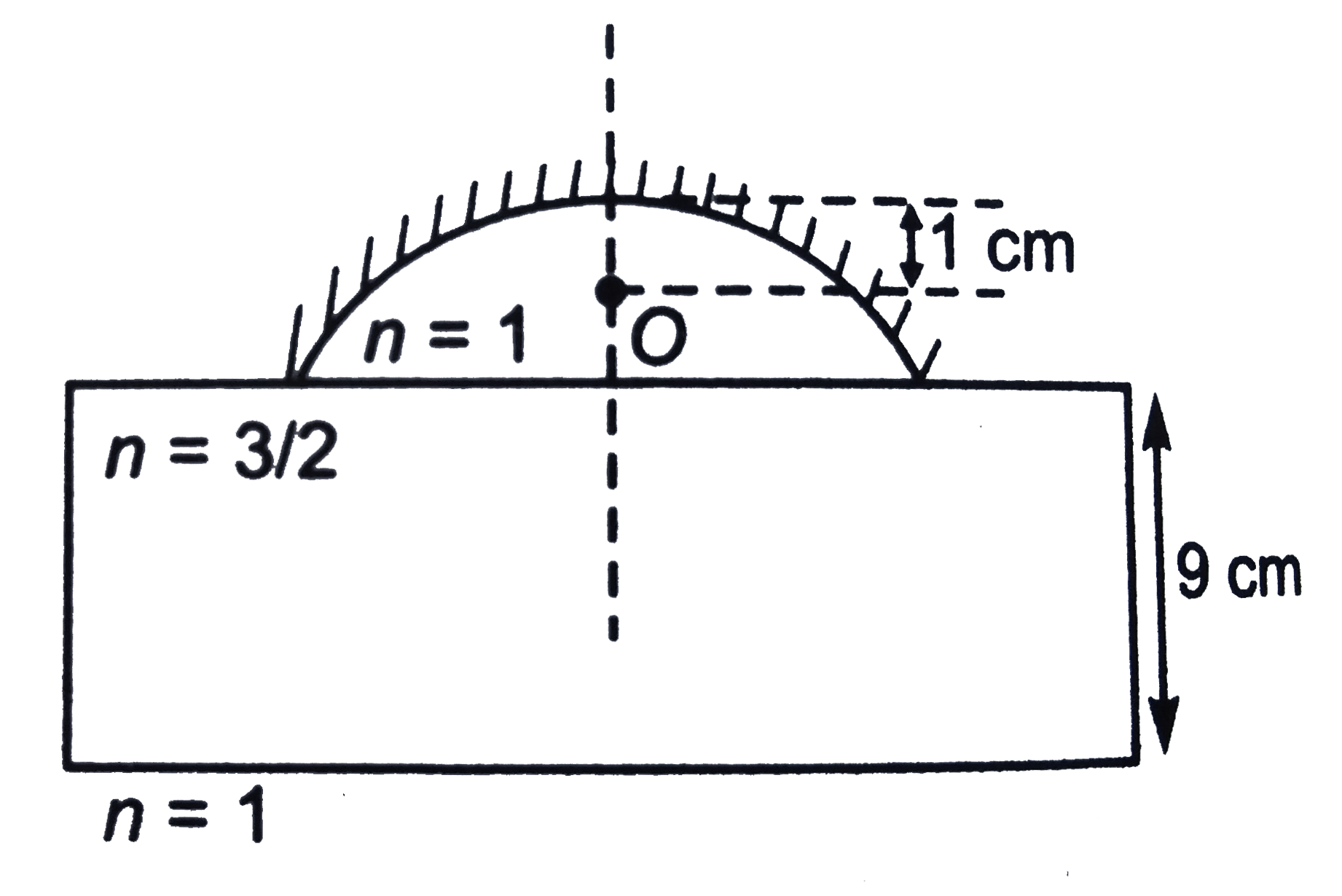
View Solution