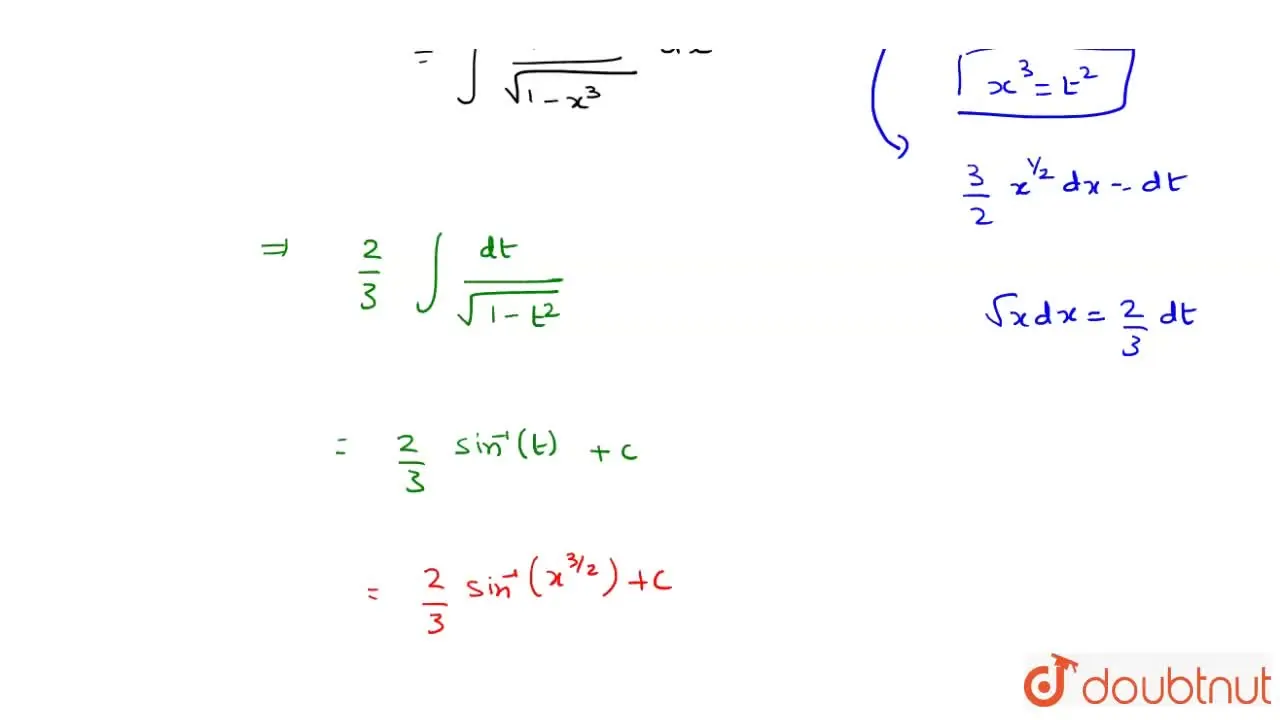Evaluate ∫√x1−x3dx
Video Solution
Text Solution
The correct Answer is:23sin−1(x3/2)+c
|
Answer
Step by step video, text & image solution for Evaluate intsqrt((x)/(1-x^3))dx by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023
Related Playlists
- BOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - PRACTICE PAPER I EXERCISE - Section B9videos
PRACTICE PAPER I
- BOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - PRACTICE PAPER I EXERCISE - Section C9videos
PRACTICE PAPER I
- BOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - MATRICES AND DETERMINANTSEXERCISE - SIX MARK QUESTIONS20videos
MATRICES AND DETERMINANTS
- BOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - PRACTICE PAPER IIEXERCISE - Section D6videos
PRACTICE PAPER II
Similar Questions
Evaluate ∫√1−x1+xdx
View SolutionEvaluate: ∫√1+x1−xdx
View Solution∫√x−ab−xdx
View SolutionEvaluate: ∫√1−x1+xdx
View SolutionEvaluate ∫√1−x1+xdx.
View SolutionEvaluate ∫√x1−xdx
View SolutionEvaluate ∫√(x−1)(2−x)dx.
View Solution∫√1+x1−xdx=?
View SolutionEvaluate: ∫√1−x1+x dx
View SolutionEvaluate: ∫√a−xa+xdx
View Solution