Question

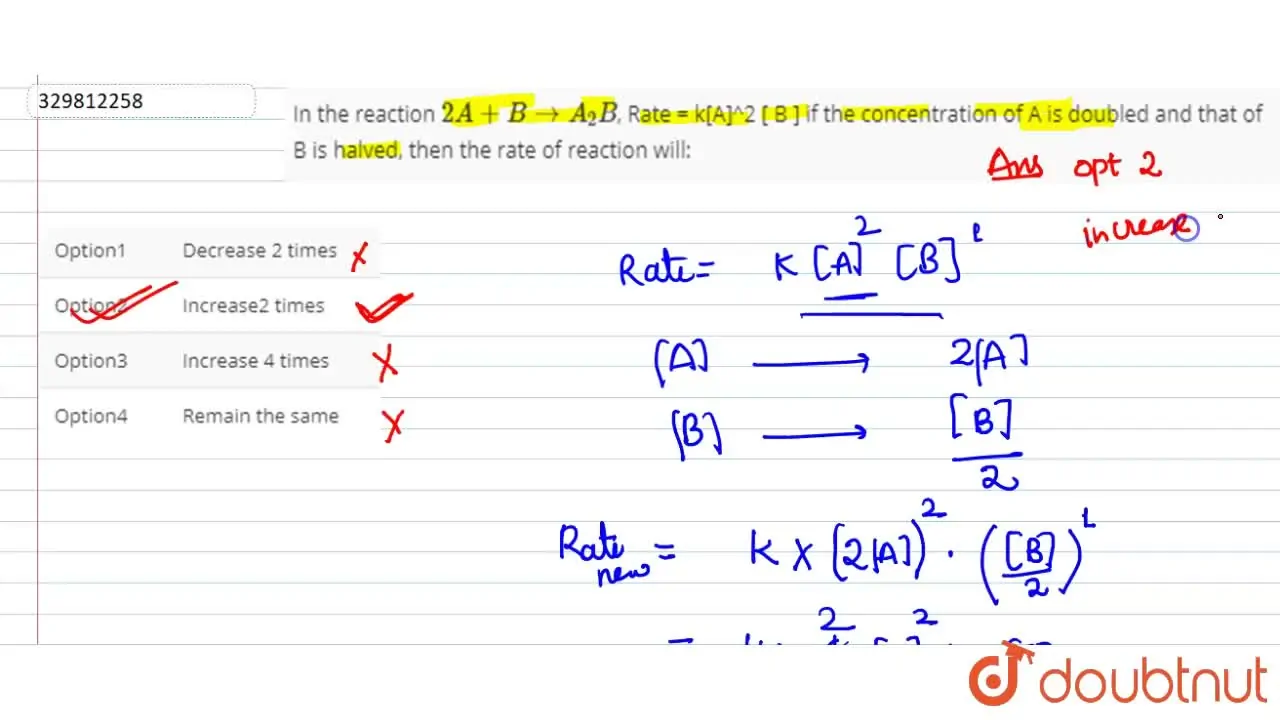
In the reaction $2A+B\to A_{2}B$,
Rate = k[A]^2
[
B
]
if the concentration of A is doubled and that of B is halved, then the rate of reaction will:

Options

A. Decrease 2 times
B. Increase2 times
C. Increase 4 times
D. Remain the same

Answer

B

Explanation

To solve the problem, we need to analyze how changing the concentrations of reactants A and B affects the rate of the reaction. The rate of the reaction is given by the equation:

$$\text{Rate}=k\left(A{)}^2\right(B)$$

Step 1: Write down the initial rate expression
The initial rate expression is:
\( \text{Rate} = k(A)^2(B) \)

Step 2: Substitute the new concentrations
We are told that the concentration of A is doubled and the concentration of B is halved. Therefore, we can express the new concentrations as:
- New concentration of A = \( 2(A) \)
- New concentration of B = \( \frac{(B)}{2} \)

Step 3: Substitute the new concentrations into the rate equation
Now, we substitute these new concentrations into the rate expression:
\( \text{New Rate} = k(2(A))^2\left(\frac{(B)}{2}\right) \)

Step 4: Simplify the new rate expression
Now, let's simplify the expression:
\( \text{New Rate} = k(4(A)^2)\left(\frac{(B)}{2}\right) \)
\( \text{New Rate} = k \cdot 4(A)^2 \cdot \frac{(B)}{2} \)
\( \text{New Rate} = k \cdot 2(A)^2(B) \)

Step 5: Relate the new rate to the old rate
From the original rate expression, we know:
\( \text{Old Rate} = k(A)^2(B) \)

Thus, we can express the new rate in terms of the old rate:
\( \text{New Rate} = 2 \cdot \text{Old Rate} \)

Conclusion
This means that the new rate of the reaction is twice the old rate. Therefore, the rate of reaction will increase by 2 times.

Final Answer
The rate of reaction will increase by 2 times.

---