The following distribution gives the daily income of 50 workers of a factory:
Convert the distribution to a less than type cumulative frequency distribution and draw its ogive. Hence obtain the median daily income.
The correct Answer is:Median daily income = 345
To convert the given distribution of daily income into a less than type cumulative frequency distribution and to find the median, we will follow these steps:
Step 1: Create a Cumulative Frequency Table
We will first create a table to organize the data. The table will have the following columns: Daily Income Range, Number of Workers (Frequency), and Less Than Type Cumulative Frequency.
| Daily Income Range | Number of Workers (Frequency) | Less Than Type Cumulative Frequency |
|---------------------|-------------------------------|-------------------------------------|
| 200 - 250 | 10 | 10 |
| 250 - 300 | 5 | 15 |
| 300 - 350 | 11 | 26 |
| 350 - 400 | 8 | 34 |
| 400 - 450 | 6 | 40 |
| 450 - 500 | 10 | 50 |
Step 2: Calculate Less Than Type Cumulative Frequencies
- For the range 200 - 250: Cumulative frequency = 10
- For the range 250 - 300: Cumulative frequency = 10 + 5 = 15
- For the range 300 - 350: Cumulative frequency = 15 + 11 = 26
- For the range 350 - 400: Cumulative frequency = 26 + 8 = 34
- For the range 400 - 450: Cumulative frequency = 34 + 6 = 40
- For the range 450 - 500: Cumulative frequency = 40 + 10 = 50
Step 3: Plot the Ogive
To draw the ogive, we will plot the cumulative frequencies against the upper limits of the income ranges.
- Points to plot:
- (250, 10)
- (300, 15)
- (350, 26)
- (400, 34)
- (450, 40)
- (500, 50)
Step 4: Draw the Ogive
1. Draw a graph with the x-axis representing Daily Income and the y-axis representing Cumulative Frequency.
2. Mark the points obtained from the table.
3. Connect the points with a smooth curve.
Step 5: Find the Median
To find the median, we need to locate n/2 where n is the total number of workers.
- Total number of workers, n=50
- Therefore, n/2=25
Now, we look at the cumulative frequency to find the median class:
- The cumulative frequency just greater than 25 is 26, which corresponds to the income range 300 - 350.
Median Class: 300 - 350
Step 6: Apply the Median Formula
Using the median formula:
Median=L+(n2−CFf)×h
Where:
- L = lower limit of the median class = 300
- n = total frequency = 50
- CF = cumulative frequency of the class preceding the median class = 15 (for the class 250 - 300)
- f = frequency of the median class = 11
- h = class width = 50 (from 300 to 350)
Substituting the values:
Median=300+(25−1511)×50
=300+(1011)×50
=300+50011
=300+45.45≈345.45
Final Result
The median daily income is approximately 345.45.
---
|---------------------|-------------------------------|-------------------------------------|
| 200 - 250 | 10 | 10 |
| 250 - 300 | 5 | 15 |
| 300 - 350 | 11 | 26 |
| 350 - 400 | 8 | 34 |
| 400 - 450 | 6 | 40 |
| 450 - 500 | 10 | 50 |
- For the range 250 - 300: Cumulative frequency = 10 + 5 = 15
- For the range 300 - 350: Cumulative frequency = 15 + 11 = 26
- For the range 350 - 400: Cumulative frequency = 26 + 8 = 34
- For the range 400 - 450: Cumulative frequency = 34 + 6 = 40
- For the range 450 - 500: Cumulative frequency = 40 + 10 = 50
- (250, 10)
- (300, 15)
- (350, 26)
- (400, 34)
- (450, 40)
- (500, 50)
2. Mark the points obtained from the table.
3. Connect the points with a smooth curve.
- Therefore, n/2=25
- The cumulative frequency just greater than 25 is 26, which corresponds to the income range 300 - 350.
- L = lower limit of the median class = 300
- n = total frequency = 50
- CF = cumulative frequency of the class preceding the median class = 15 (for the class 250 - 300)
- f = frequency of the median class = 11
- h = class width = 50 (from 300 to 350)
=300+(1011)×50
=300+50011
=300+45.45≈345.45
Topper's Solved these Questions
- PRACTICE TEST-IIBOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - PRACTICE TEST-IIEXERCISE - Section B
6 Videos
- PRACTICE TEST-1 BOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - PRACTICE TEST-1 EXERCISE - SECTION-C
14 Videos
- PROBABILITYBOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - PROBABILITYEXERCISE - PRACTICE-TEST
11 Videos
Similar Questions
The following distribution gives the daily income of 50 workers of a factory.
Daily income400−420420−440440−460460−480480−500Number of workers12148610
Convert this distribution to less than type of cumulative frequency distribution and draw its ogive.
View SolutionThe following distribution gives the daily income of 50 workers of a factory: Daily income (in Rs): 100-120 120-140 140-160 160-180 180-200 Number of workers: 12 14 8 6 10 Convert the above distribution to a less than type cumulative frequency distribution and draw its ogive.
View SolutionThe following table gives the height of 42 trees in meters:

Change the above distribution to less than type distribution and draw its ogive. Hence obtain the median value.
View SolutionThe following table gives the daily income of 50 workers of a factory: Daily income (in Rs): 100-120 120-140 140-160 160-180 180-200 Number of workers: 12 14 8 6 10 Find the mean, mode and median of the above data.
View SolutionThe following distribution gives the daily income of 50 workers of a factory: Convert the distribution above to a less than type cumulative frequency distribution and draw its ogive
View SolutionThe following distribution gives the daily income of 50 workers of a factory.
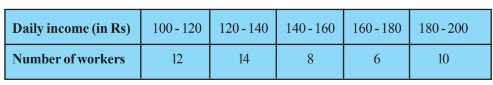
Convert the distribution above to a less than type cumulative frequency distribution, and draw its ogive.
View SolutionThe following distribution gives the daily income of 60 workers of a factory. Convert the distribution above to a less than type cumulative frequency distribution, and draw its ogive.
View SolutionFind out mode of the following series :
Wages (Rs.) less than200300400500600700800900Number of Workers5183870909598100
View SolutionThe following table gives the daily income of ten workers in a factory. Find the arithmetic mean.
WorkersABCDEFGHIJDaily Income(in Rs.)120150180200250300220350370260
View SolutionThe following table gives the daily income of ten workers in a factory. Find the arithmetic mean.
WorkersABCDEFGHIJDaily Income(in Rs.)120150180200250300220350370260
View Solution
