IN the centre of a rectangular lawn of dimensions 50m×40m, a rectangular pond has to be cnstructed, so that the area of the grass surrounding the pond would be 1184m2. Find the length and breadth of the pond.
The correct Answer is:Breadth of pond = 24 m
To solve the problem of finding the dimensions of the pond in the rectangular lawn, we can follow these steps:
Step 1: Understand the dimensions of the lawn
The dimensions of the rectangular lawn are given as:
- Length (L) = 50 m
- Breadth (B) = 40 m
Step 2: Calculate the area of the lawn
The area of the rectangular lawn can be calculated using the formula:
Area of lawn=Length×Breadth=50×40=2000m2
Step 3: Set up the equation for the area of the pond
Let the width of the grass surrounding the pond be x. Then, the dimensions of the pond can be expressed as:
- Length of the pond = 50−2x
- Breadth of the pond = 40−2x
Step 4: Calculate the area of the grass surrounding the pond
The area of the grass surrounding the pond is given as 1184m2. Therefore, the area of the pond can be calculated as:
Area of pond=Area of lawn−Area of grass=2000−1184=816m2
Step 5: Set up the equation for the area of the pond
Using the dimensions of the pond, we can express the area of the pond as:
Area of pond=(50−2x)(40−2x)
Setting this equal to the area of the pond we calculated:
(50−2x)(40−2x)=816
Step 6: Expand the equation
Expanding the left-hand side:
2000−100x−80x+4x2=816
This simplifies to:
4x2−180x+2000−816=0
4x2−180x+1184=0
Step 7: Simplify the quadratic equation
We can divide the entire equation by 4 to simplify:
x2−45x+296=0
Step 8: Solve the quadratic equation using the quadratic formula
The quadratic formula is given by:
x=−b±√b2−4ac2a
Here, a=1, b=−45, and c=296:
b2−4ac=(−45)2−4×1×296=2025−1184=841
Now substituting back into the formula:
x=45±√8412×1=45±292
Calculating the two possible values for x:
1. x=742=37
2. x=162=8
Step 9: Calculate the dimensions of the pond
Using x=8 (since x=37 would yield negative dimensions):
- Length of the pond = 50−2(8)=34m
- Breadth of the pond = 40−2(8)=24m
Final Answer
The dimensions of the pond are:
- Length = 34 m
- Breadth = 24 m
The dimensions of the rectangular lawn are given as:
- Length (L) = 50 m
- Breadth (B) = 40 m
The area of the rectangular lawn can be calculated using the formula:
Area of lawn=Length×Breadth=50×40=2000m2
Let the width of the grass surrounding the pond be x. Then, the dimensions of the pond can be expressed as:
- Length of the pond = 50−2x
- Breadth of the pond = 40−2x
The area of the grass surrounding the pond is given as 1184m2. Therefore, the area of the pond can be calculated as:
Area of pond=Area of lawn−Area of grass=2000−1184=816m2
Using the dimensions of the pond, we can express the area of the pond as:
Area of pond=(50−2x)(40−2x)
Setting this equal to the area of the pond we calculated:
(50−2x)(40−2x)=816
Expanding the left-hand side:
2000−100x−80x+4x2=816
This simplifies to:
4x2−180x+2000−816=0
4x2−180x+1184=0
We can divide the entire equation by 4 to simplify:
x2−45x+296=0
The quadratic formula is given by:
x=−b±√b2−4ac2a
Here, a=1, b=−45, and c=296:
b2−4ac=(−45)2−4×1×296=2025−1184=841
Now substituting back into the formula:
x=45±√8412×1=45±292
Calculating the two possible values for x:
1. x=742=37
2. x=162=8
Using x=8 (since x=37 would yield negative dimensions):
- Length of the pond = 50−2(8)=34m
- Breadth of the pond = 40−2(8)=24m
The dimensions of the pond are:
- Length = 34 m
- Breadth = 24 m
Topper's Solved these Questions
- QUADRATIC EQUATIONS BOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - QUADRATIC EQUATIONS EXERCISE - PRACTICE TEST
10 Videos
- QUADRATIC EQUATIONS BOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - QUADRATIC EQUATIONS EXERCISE - SHORT ANSWER TYPE QUESTIONS-II
19 Videos
- PROBABILITYBOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - PROBABILITYEXERCISE - PRACTICE-TEST
11 Videos
- REAL NUMBERSBOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - REAL NUMBERSEXERCISE - LONG ANSWER TYPE QUESTION
15 Videos
Similar Questions
In the centre of a rectangular lawn of dimensioms 50m×40m a rectangular pond has to be constructed so that the area of the grass surroundng the pond would be 1184m2 Find the length and breadth of the pond
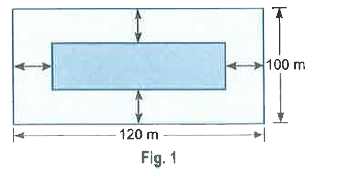
View SolutionIn the centre of rectangular plot of land of dimensions 120m×100 m, a rectangular portion is to be covered with trees so that the area of the remaining part of the plot is 10500m2 . Find the area to be planted.
View SolutionThe length of a rectangular playground is 2m longer than its breadth. If the area of the playground is 195sq.m. Find the length and breadth of the field
View SolutionThe length and breadth of a rectangular park are 50 m and 40 m respectively. Find the ratio of the length to the breadth of the park.
View SolutionHow many rectangular plots of dimensions 40 m × 60 m can be cut from a rectangular ground having dimensions 120 m × 160 m?
View SolutionThe area of a rectangular plot is 528m2. The length of the plot (in metres) is one metre more then twice its breadth. Find the length and the breadth of the plot.
View SolutionThe perimeter of a rectangular plot of land is 80 m and its breadth is 16 m . Find the length and area of the plot .
View SolutionThe length and breadth of a rectangular park is 250 m and 125 m respectively. Find the area and perimeter of the park.
View SolutionA square lawn has a 2 m wide path surrounding it. If the area of the path is 136 m2 , find the area of the lawn.
View SolutionLength and breadth of a rectangular field are 50m and 15m respectively. Find the ratio of the length to the breadth of the field.
View Solution
