Moment of inertia of a ring of mass M and radius R about an axis passing through the centre and perpendicular to the plane is
Remember that in case of continuous mass distribution, we use the formula I=∫(dm)r2 to find out the moment of inertia of the body. AA. is the axis about which rotation of the ring is being considered.
Mass of the ring = M, circumference of the ring =2πR
Mass per unit length of the ring =(M2πR)=λ (say)
Consider a small element of the ring at an angle θ from a particular reference radius. The element subtends an angle dθ at the centre.
Length of the element =Rdθ
Mass of the element =(λRdθ)
Moment of inertia of the element =(λRdθ)R2
Moment of inertia of the ring =∫2π0(λRdθ)R2=MR2

Topper's Solved these Questions
- ROTATIONAL MECHANICSBOOK - FIITJEECHAPTER - ROTATIONAL MECHANICSEXERCISE - SOLVED PROBLEMS (SUBJECTIVE)
10 Videos
- ROTATIONAL MECHANICSBOOK - FIITJEECHAPTER - ROTATIONAL MECHANICSEXERCISE - SOLVED PROBLEMS (OBJECTIVE)
14 Videos
- PHYSICS PART2BOOK - FIITJEECHAPTER - PHYSICS PART2EXERCISE - Numerical Based Question Decimal Type
6 Videos
- SEMICONDUCTOR AND DEVICEBOOK - FIITJEECHAPTER - SEMICONDUCTOR AND DEVICEEXERCISE - SOLVED PROBLEMS Objective: Level-I
20 Videos
Similar Questions
Calculate the moment of inertia of a ring of mass 2 kg and radius 50 cm about an axis passing through its centre and perpendicular to its plane
View SolutionWhat is the moment of inertia of a ring of mass 2kg and radius 50 cm about an axis passing through its centre and perpendicular to its plane ? Also find the moment of inertia about a parallel axis through its edge.
View Solution
Knowledge Check
The moment of inertia of a thin uniform ring of mass I kg about an axis passing through the centre and perpendicular to the plane of the ring is 0.25 kg m2. Then the diameter of the ring is
A0.25mB0.5mC0.75mD1mThe moment of inertia of a circular ring of mass 1 kg about an axis passing through its centre and perpendicular to its plane is 4 kg m2. The diameter of the ring is
A2 mB4 mC5 mD6 m
Moment of inertia of a half ring of mass m and radius R about an axis passing through point A perpendicular to the plane of the paper is IA. If IC is the moment of inertia of the ring about an axis perpendicular to the plane of paper and passing through point C, then
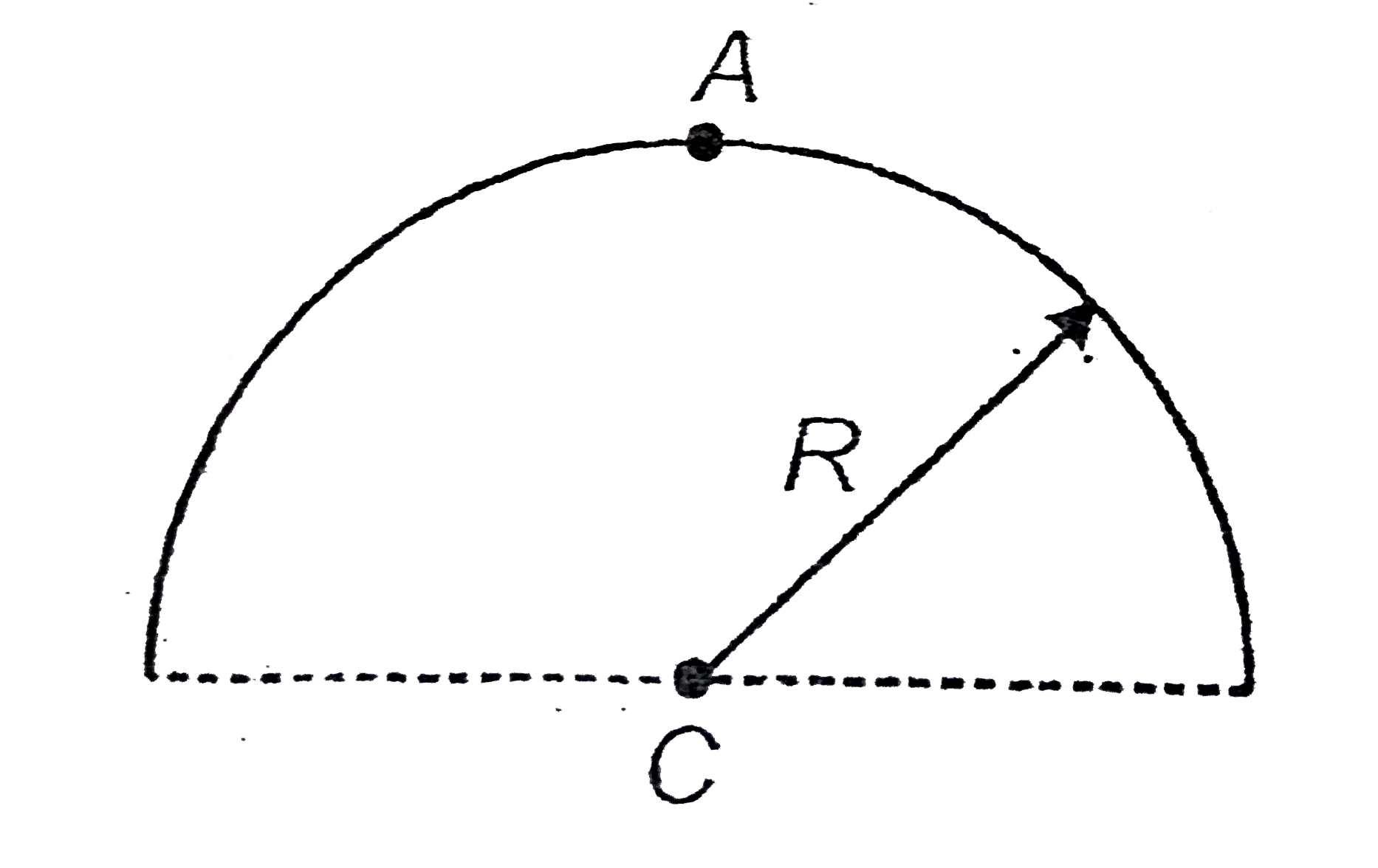
View SolutionCalculate the moment of inertia of a ring of mass 2kg and radius 2cm about an axis passing through its centre and perpendicular to its surface.
View SolutionCalculate the moment of inertia of a disc of radius R and mass M, about an axis passing through its centre and perpendicular to the plane.
View SolutionCalculate the moment of Inertia of a semicircular disc of mass M and radius R about an axis passing through its centre and perpendicular to its plane.
View SolutionThe M.I. of a disc of mass M and radius R, about an axis passing through the centre O and perpendicular to the plane of the disc is MR22 . If one quarter of the disc is removed, the new moment of inertia of the disc will be
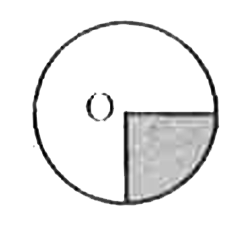
View SolutionThe moment of inertia of a ring of mass M and radius R about PQ axis will be

View Solution
