Find the potential $V$ at $P$ in the gravitational field of fixed point mass $M$ .

Video Solution

Text Solution

Generated By DoubtnutGPT

To find the gravitational potential $V$ at a point $P$ in the gravitational field of a fixed point mass $M$ , we can follow these steps:
Step 1: Understand the Definition of Gravitational Potential
Gravitational potential $V$ at a point is defined as the work done by an external agent in bringing a unit mass from infinity to that point against the gravitational field.
Step 2: Set Up the Problem
Let the distance from the point mass $M$ to the point $P$ be $x$ . We will calculate the work done in bringing a unit mass from infinity to the point $P$ .
Step 3: Write the Expression for Gravitational Force
The gravitational force $F$ acting on a mass $m$ due to the point mass $M$ is given by:
$F = \frac{G M m}{x^{2}}$
where $G$ is the gravitational constant.
Step 4: Calculate the Work Done
The work done $W$ in moving a unit mass from infinity to a distance $x$ can be expressed as:
$W = \int_{\infty}^{x} F d x$
Substituting the expression for $F$ :
$W = \int_{\infty}^{x} \frac{G M}{x^{2}} d x$
Step 5: Evaluate the Integral
Now, we can evaluate the integral:
$W = G M \int_{\infty}^{x} \frac{1}{x^{2}} d x$
The integral of $\frac{1}{x^{2}}$ is:
$\int \frac{1}{x^{2}} d x = - \frac{1}{x}$
Thus, we have:
$W = G M {(- \frac{1}{x})}_{\infty}^{x} = G M (- \frac{1}{x} - 0) = - \frac{G M}{x}$
Step 6: Find the Gravitational Potential
Since we are considering the work done per unit mass, the gravitational potential $V$ at point $P$ is:
$V = \frac{W}{m} = - \frac{G M}{x}$
Conclusion
Therefore, the gravitational potential $V$ at point $P$ due to the point mass $M$ is:
$V = - \frac{G M}{x}$
---

Updated on:18/9/2024

Find the potential energy of the gravitational interaction of a point mass $m$ and a rod of mass $m$ and length $l$ , if they are along a straight line. Point mass is at a distance of a from the end of the rod.

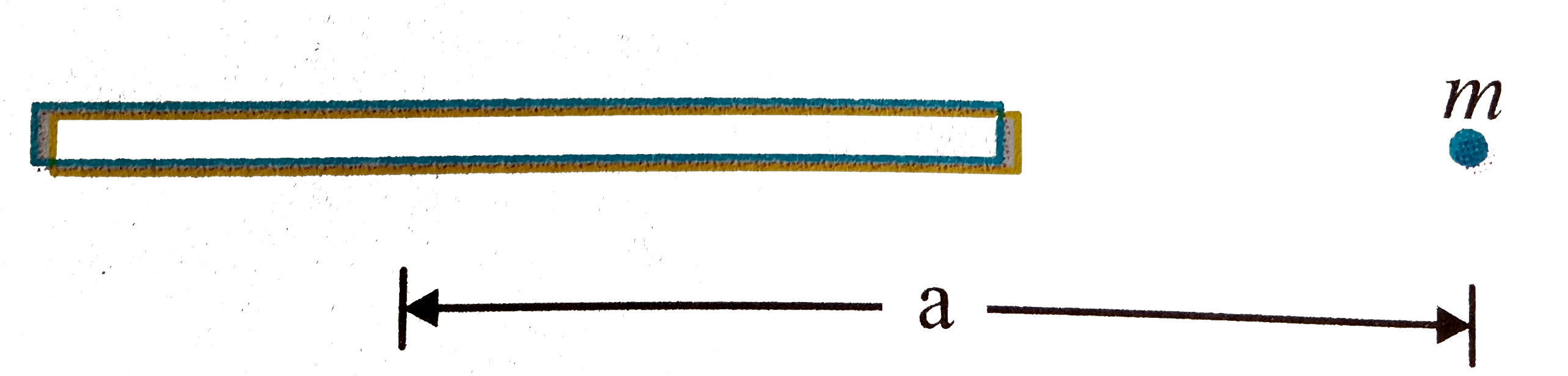
Find the potential energy of the gravitational
A $U = \frac{- G m^{2}}{l} ln (\frac{2 a + l}{2 a - l})$
B $U = \frac{- G m^{2}}{l^{2}} ln (\frac{2 a + l}{2 a - l})$
C $U = \frac{- G m^{2}}{l^{2}} ln (\frac{2 a - l}{2 a + l})$
D $U = \frac{- G m^{2}}{l^{2}} {ln (\frac{2 a - l}{2 a + l})}^{2}$
Two bodies of masses m and M are placed at distance d apart. The gravitational potential (V) at the position where the gravitational field due to them is zero V is
A $V = - \frac{G}{d} (m + M)$
B $V = - \frac{G}{d}$
C $V = - \frac{G M}{d}$
D $V = - \frac{G}{d} {\sqrt{\sqrt{m} + \sqrt{M}}}^{2}$
Two bodies of masses $M_{1}$ and $M_{2}$ are kept separeated by a distance d. The potential at the point where the gravitational field produced by them is zero, is :-
A $- \frac{G}{d} (M_{1} + M_{2} + 2 \sqrt{M_{1} M_{2}})$
B $- \frac{G}{d} (M_{1} M_{2} + 2 \sqrt{M_{1} + M_{2}})$
C $- \frac{G}{d} (M_{1} - M_{2} + 2 \sqrt{M_{1} M_{2}})$
D $- \frac{G}{d} (M_{1} M_{2} - 2 \sqrt{M_{1} + M_{2}})$