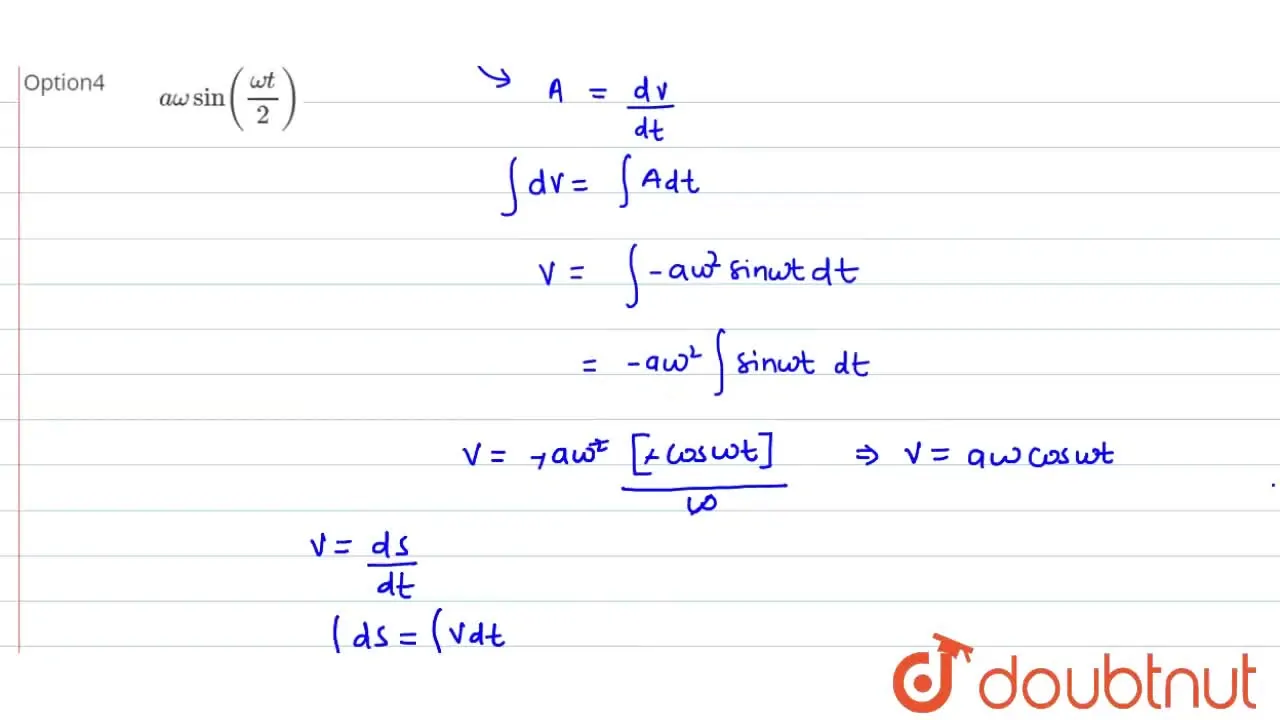
The acceleration of a particle at time is given by A=−aω2sinωt. Its displacement at time t is
−aω2sinωt
asinωt
acosωt
aωsin(ωt2)
The correct Answer is:B
Step by step video, text & image solution for The acceleration of a particle at time is given by A=-aomega^(2) sinomegat. Its displacement at time t is by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Related Playlists
- BOOK - FIITJEECHAPTER - KINEMATICSEXERCISE - ASSIGNMENT PROBLEMS (OBJECTIVE) LEVEL-I (ASSERTION REASONING TYPE)2videos
KINEMATICS
- BOOK - FIITJEECHAPTER - KINEMATICSEXERCISE - ASSIGNMENT PROBLEMS (OBJECTIVE) LEVEL-II20videos
KINEMATICS
- BOOK - FIITJEECHAPTER - KINEMATICSEXERCISE - ASSIGNMENT PROBLEMS (SUBJECTIVE) LEVEL-II & III15videos
KINEMATICS
- BOOK - FIITJEECHAPTER - HEAT AND TEMPERATUREEXERCISE - NUMERICAL BASES QUESTIONS1videos
HEAT AND TEMPERATURE
- BOOK - FIITJEECHAPTER - LAWS OF MOTIONEXERCISE - COMPREHENSION-III2videos
LAWS OF MOTION
Similar Questions
The displacement s of a particle at time t is given by s=αsinωt+βcosωt then acceleration at time t is
View SolutionThe acceleration of a particle, given by relation, a=−5ω2sin(ωt). At t=0, x=0 and v=5ω. The displacement of this paticle at time t will be
View SolutionAcceleration of a particle is given by
a=3t2+2t+1
Where t is time . Find
(i) its velocity at time t assuming velocity to be 10 m/s at t = 0.
(ii) its position at time t assuming that the particle is at origin at t = 0.
(iii) What is the average speed of the particle in the duration t = 0 to t = 1s?
(iv) What is the average acceleration of the particle in the duration t = 0 to t = 1s?View SolutionThe acceleration of a particle which is depend on time is given by following function a = 2t + 1 and at time t = 0, x = 1m and ui = 2m/s. Then find out displacement of the particle at t = 3 sec.
View SolutionThe position of a point in time t is given by x=a+bt−ct2,y=at+bt2. Its acceleration at time t is
View SolutionThe position of a point in time t is given by x=a+bt−ct2,y=at+bt2. Its acceleration at time t is
View SolutionThe acceleration of a moving particle whose space time equation is given by s=3t2+2t−5, is
View SolutionThe displacement of a particle in S.H.M. is given by x=sinωt+√3cosωtm. Then at t = 0 its displacement in metres is
View SolutionThe displacement of a particle in SHM is given by x=sinωt+cosωt. Then its amplitude and initial displacement are
View SolutionThe position of a point in time 't' is given by
x=a+bt−ct2,y=at+bt2. Its acceleration at time 't' isView Solution